Question

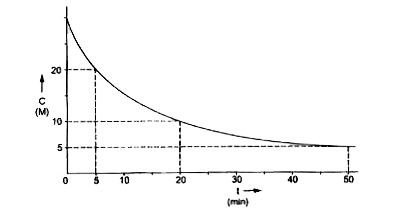
In a first ordr reaction, reactant concentration 'C' varies with time 't' as

Options

A. C decreases with `(1)/(t)`
B. log C decreases with `(1)/(t)`
C. `(1)/(c)` increases linearly with t
D. log C decreases lineary with t

Answer

D

Explanation

To solve the problem regarding how the reactant concentration 'C' varies with time 't' in a first-order reaction, we can follow these steps:

1. Understand the First-Order Reaction:
In a first-order reaction, the rate of reaction is directly proportional to the concentration of one reactant. The general form of the rate equation is:
\( \text{Rate} = -\frac{dC}{dt} = kC \)
where \( k \) is the rate constant.

2. Integrate the Rate Equation:
Rearranging the rate equation gives:
\( \frac{dC}{C} = -k \, dt \)
Integrating both sides, we get:
\( \int \frac{dC}{C} = -k \int dt \)
This results in:
\( \ln C = -kt + \ln C_0 \)
where \( C_0 \) is the initial concentration at time \( t = 0 \).

3. Rearranging the Equation:
Exponentiating both sides gives:
\( C = C_0 e^{-kt} \)
Alternatively, we can express it in logarithmic form:
\( \ln C = \ln C_0 - kt \)

4. Expressing in Logarithmic Form:
We can convert the natural logarithm to base 10 logarithm using the conversion factor \( \ln x = 2.303 \log_{10} x \):
\( \log_{10} C = \log_{10} C_0 - \frac{kt}{2.303} \)

5. Identifying the Linear Relationship:
This equation can be rearranged to fit the linear equation format \( y = mx + b \):
\( \log_{10} C = -\frac{k}{2.303} t + \log_{10} C_0 \)
Here, \( y = \log_{10} C \), \( m = -\frac{k}{2.303} \), \( x = t \), and \( b = \log_{10} C_0 \).

6. Conclusion:
From the linear relationship, we can conclude that as time \( t \) increases, \( \log_{10} C \) decreases linearly. Therefore, the concentration \( C \) decreases exponentially with time.

Final Answer:
The correct answer is that \( \log_{10} C \) decreases linearly with time \( t \).