A shell is fired from a cannon with a speed of 100m/s at an angle 30∘with the vertical (y-direction). At the highest point of its trajectory, the shell explodes into two fragments of masses in the ratio 1:2. The lighter fragment moves vertically upwards with an initial speed of 200m/s. What is the speed of the heavier fragment at the time of explosion?
The velocity of shell at the highest point is v=usinθ=100×sin30∘=50m/s. Let in be the mass of the shell. Then the mass of the lighter fragment is m/3 and that of heavier fragment is 2m/3. Initial momentum of the shell before explosion is
mv=50m
As no external force is acting on the shell, we can conserve momentum of the shell before and after its explosion.
In x-direction
mv=2m3v2cosθ⇒v2cosθ=32v..............i
in y- direction
0=m3v1−2m3v2sinθ⇒v2sinθ=v12........ii ltbr Squar∈gandadd∈geqniandiiwe≥t<br>v_(2)^(2)=9/4v^(2)+1/4v_(1)^(2)<br>implies v_(2)=1/2sqrt(9v^(2)+v_(1)^(2))=1/2sqrt(9xx2500+40000)=125m//s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.324videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Subjective23videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.120videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
A shell is fixed from a cannon with a speed of 100m/s at an angle 60∘ with the horizontal (positive x-direction). At the highest point of its trajectory, the shell explodes into two equal fragments. One of the fragments moves along the negative x-direction with a speed of 50m/s. What is the speed of the other fragment at the time of explosion.
View SolutionA shell is fired from a cannon with a speed of 100m/s at an angle 60∘ with the horizontal (positive x-direction). At the highest point of its trajectory, the shell explodes in to two equal fragments. One of the fragments moves is the speed of the other fragment at the time of explosion.
View Solution
Knowledge Check
- Question 1 - Select One
A shell is fired from a cannot with a speed of 100ms−1 at an anlge 60∘ with the horizontal (x-direction). At the highest point of its trajectory the shell explodes into two equal fragments. One of the fragments moves along the negative x-direction with a speed of 50ms−1. What is the speed of the other fragment at the time of explosion ?
A150ms−1B50ms−1C100ms−1D200ms−1 - Question 2 - Select One
A bullet fired from gun with a velocity 30 m/s at an angle of 60∘ with horizontal direction. At the highest point of its path, the bullet explodes into two parts with masses in the ratio 1 : 3. The lighter mass comes to rest immediately. Then the speed of the heavier mass is
A30 m/sB20 m/sC10 m/sD5 m/s - Question 3 - Select One
A shell of mass 20kg at rest explodes into two fragments whose masses are in the ratio 2:3. The smaller fragment moves with a velocity of 6m/s. The kinetic energy of the larger fragment is
A96JB216JC144JD360J
A body of mass 1 kg at rest explodes into three fragments of masses in the ratio 1 : 1 : 3 . The two pieces of equal mass fly in mutually perpendicular directions with a speed of 30m/s each . What is the velocity of the heavier fragment ?
View SolutionA shell is projected by a cannon at an angle 30∘ with the vertical for a horizontal range R. It explodes at the highest point of its trajectory. Now match the column I and II.
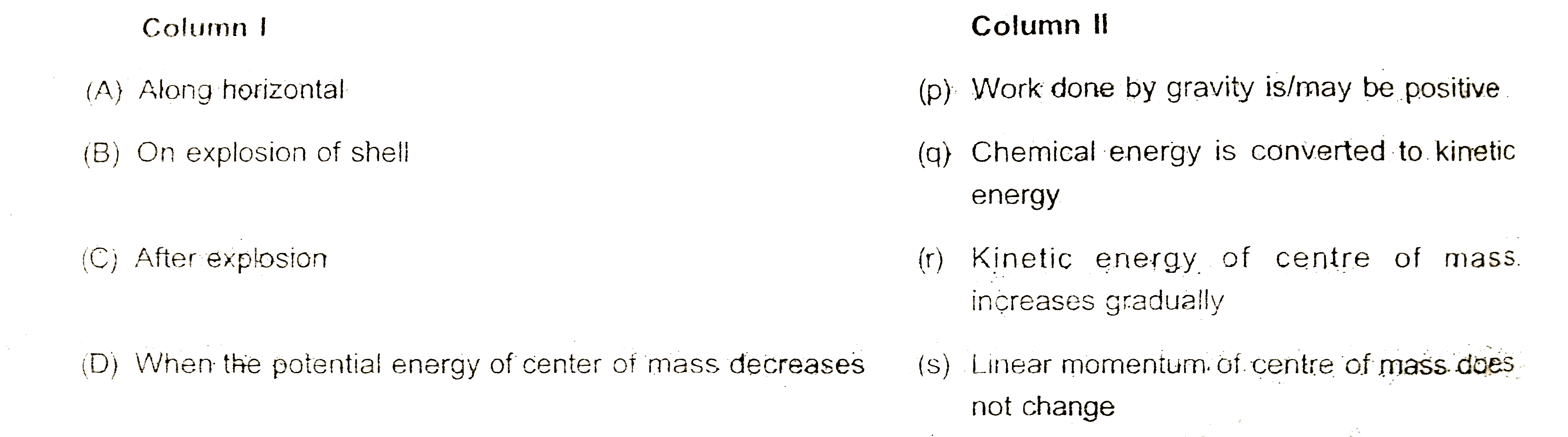 View Solution
View SolutionA projectile of mass 20 kg is fired with a velocity of 400m/sat an angle of 45∘ with the horizontal .At the highest point of the trajectocy the projectile explodes into two fragments of equal mass,one of which falls vertically downward with zero initial speed, The distance of the point where the other fragment falls from the point offiring is:
View SolutionA shell is fired from a cannon with a velocity V at an angle θ with the horizontal direction. A the highest point i its path, it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon. The speed of the other priece immediately after the explocison is
View SolutionA shell is fired from a cannon with a velocity V at an angle θ with the horizontal direction. A the highest point i its path, it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon. The speed of the other priece immediately after the explocison is
View Solution

