A particle of mass $m$ collides with another stationary particle of mass $M$ such that the second particle starts moving and the first particle stops just after the collision. Then which of the following conditions must always be valid ?

A

$\frac{m}{M} \leq 1$

B

$\frac{m}{M} = 1$

C

$e = 1$

D

$e \leq 1$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, D

If nothing is given about the nature of collision, we can not presume it as perfectly elastic. Let $e$ be the coefficient of restitution of collision. For inelastic collision $e < 1$ . The collision cannot be perfectly inelastic becaue the particles do not stick together after collision.

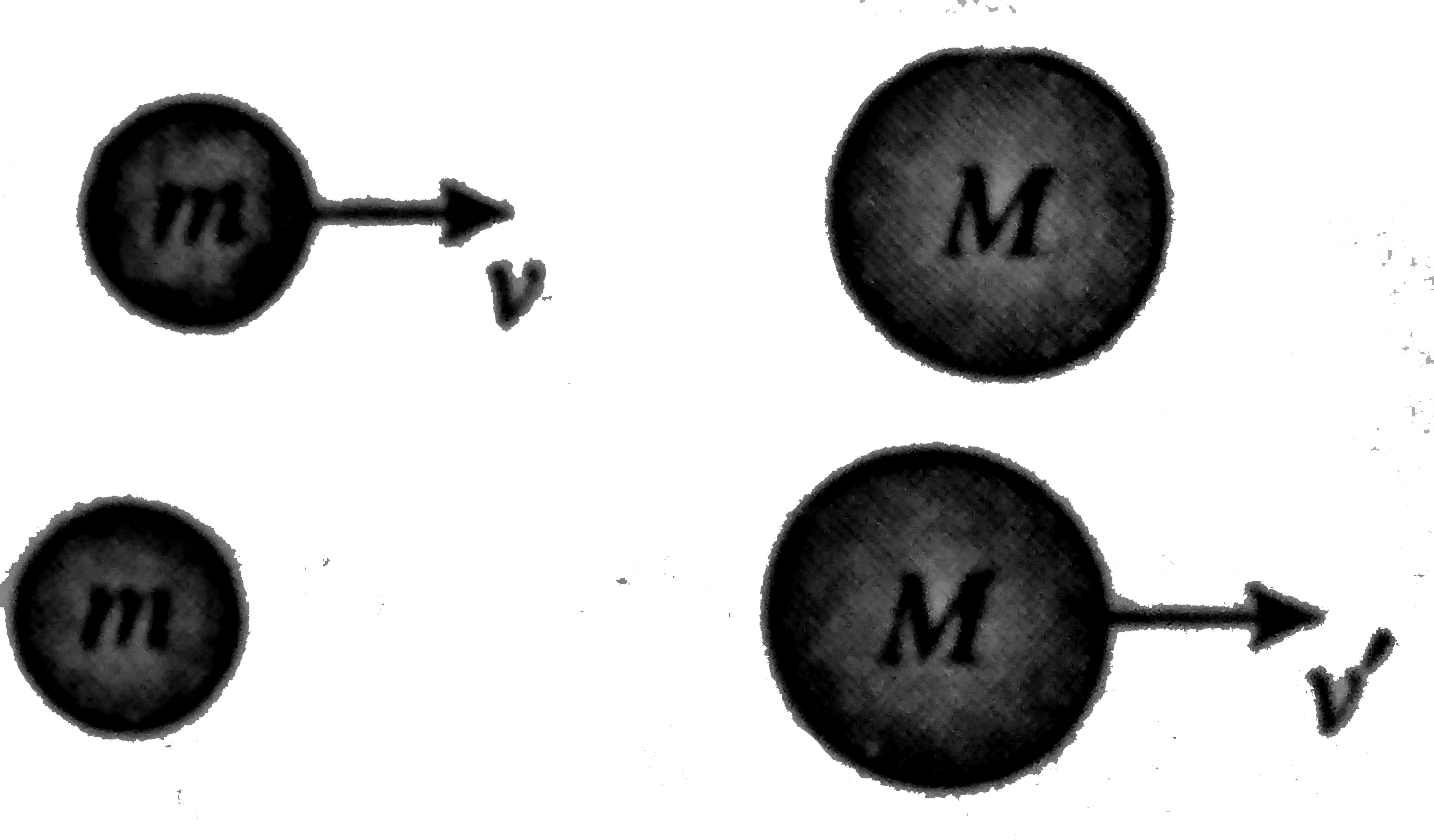
Just after collision
Conservation of linear momentum yields
$m v = M v' \Rightarrow \frac{v'}{v} = \frac{m}{M}$
Since $e = \frac{v'}{v}$ (numerically)
$\frac{m}{M} = e$
Since $e \leq 1, \frac{m}{M} \leq 1$
Hence a and d are correct.

|

Updated on:21/07/2023

Class 11 PHYSICS SYSTEM OF PARTICLES AND ROTATIONAL MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
A particle $(A)$ of mass $m_{1}$ elastically collides with another stationary particle $(B)$ of mass $m_{2}$ . Then :
A $\frac{m_{1}}{m_{2}} = \frac{1}{2}$ and the particles fly a part in the opposite direction with equal velocities.
B $\frac{m_{1}}{m_{2}} = \frac{1}{3}$ and the particles fly apart in the opposite direction with equal velocities.
C $\frac{m_{1}}{m_{2}} = \frac{2}{1}$ and the collision angle between the particles is $60^{\circ}$ symmetrically.
D $\frac{m_{1}}{m_{2}} = \frac{2}{1}$ and the particles fly apart symmetrically at an angle $90^{\circ}$
Question 2 - Select One
A particle of mass m, collides with another stationary particle of mass M. If the particle m stops just after collision, then the coefficient of restitution for collision is equal to
A1
B $\frac{m}{M}$
C $\frac{M - m}{M + m}$
D $\frac{m}{M + m}$
Question 3 - Select One
A particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
A $0.8 v$
B $0.2 v$
C $0.6 v$
D $0.4 v$

CENGAGE PHYSICS-CENTRE OF MASS-Multiple Correct

Home
Ask Doubt
Profile