According to the principle of conservation of linear momentum if the external force acting on the system is zero, the linear momentum of the system will remain conserved. It means if the centre of mass of a system is initially at rest, it will remain at rest in the absence of external force, that is, the displacement of centre of mass will be zero.
A plank of mass $M$ is placed on a smooth horizontal surface. light identical springs, each of stiffness $K$ , are rigidly connected to struts at the end of the plank as shown in Fig. When the springs are in their unextended position, the distance between their free ends is $3 l$ . A block of mass $m$ is placed on the plank and pressed against one of the springs so that it is compressed to $l$ . To keep the block at rest it is connected to the strut means of a light string. Initially, the system is at rest, Now the string is burnt.

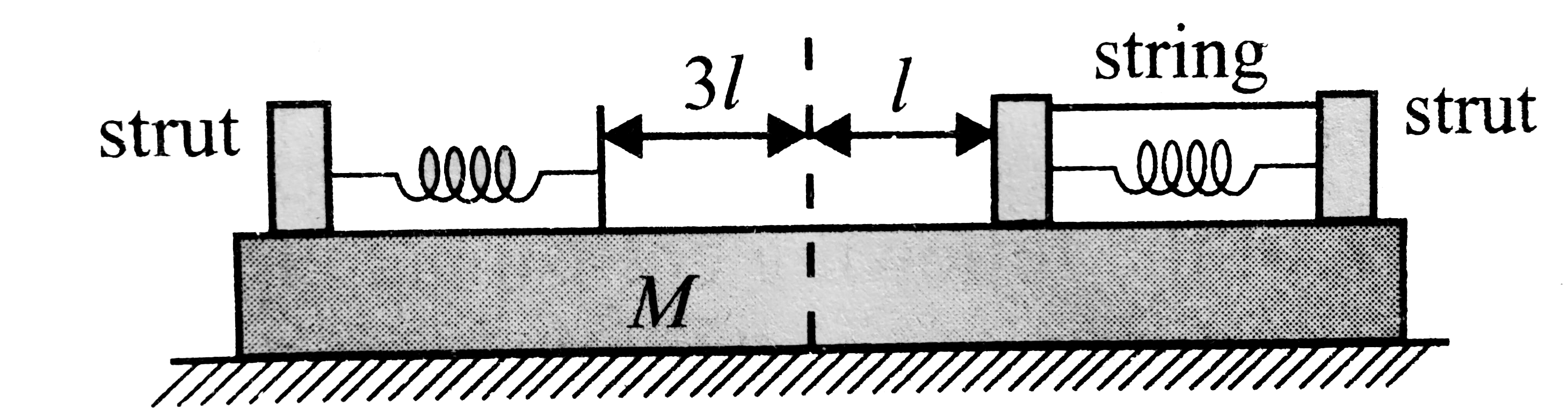
The maximum velocity of the plank is

$\sqrt{\frac{K m}{(M + m)} l}$

$\sqrt{\frac{k}{(M + m)}} l$

$\sqrt{\frac{K m}{M (M + m)}} l$

$\sqrt{\frac{k M}{m (M + m)}} l$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$M v_{M} - m v_{m} = 0$ ……….i
$\frac{1}{2} k l^{2} = \frac{1}{2} m v_{m}^{2} + \frac{1}{2} M v_{m}^{2}$ ………..ii
solving eqn i and ii
$v_{M} = \sqrt{\frac{K m}{M (M + m)} l}$

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
According to the principle of conservation of linear momentum if the external force acting on the system is zero, the linear momentum of the system will remain conserved. It means if the centre of mass of a system is initially at rest, it will remain at rest in the absence of external force, that is, the displacement of centre of mass will be zero.
A plank of mass $M$ is placed on a smooth horizontal surface. light identical springs, each of stiffness $K$ , are rigidly connected to struts at the end of the plank as shown in Fig. When the springs are in their unextended position, the distance between their free ends is $3 l$ . A block of mass $m$ is placed on the plank and pressed against one of the springs so that it is compressed to $l$ . To keep the block at rest it is connected to the strut means of a light string. Initially, the system is at rest, Now the string is burnt.

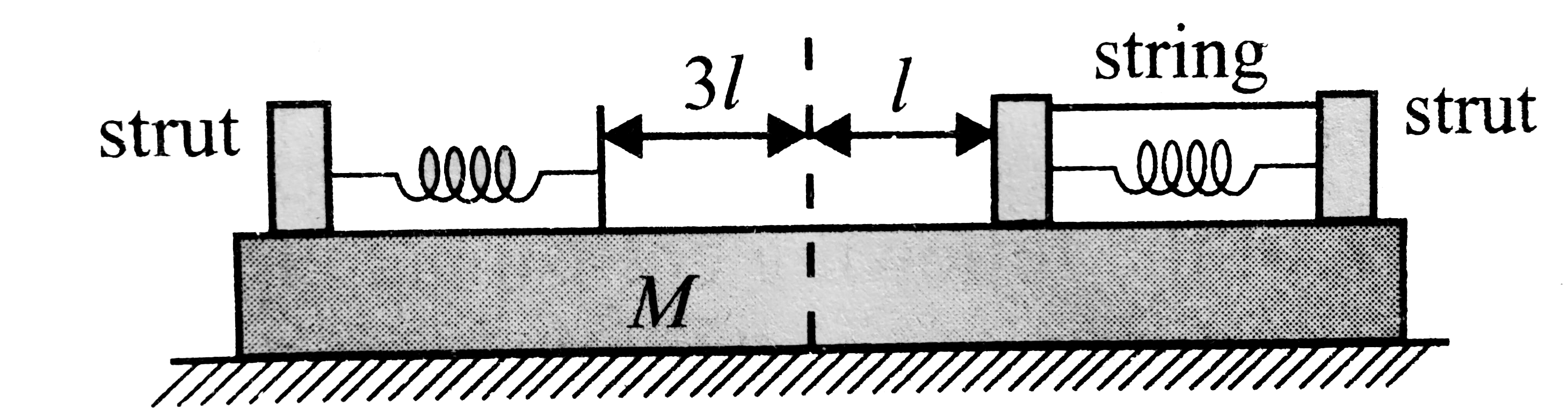
The maximum displacement of the plank is
A $\frac{5 m l}{M}$
B $\frac{5 m l}{M + m}$
C $\frac{3 m l}{M + m}$
D $\frac{4 m l}{M + m}$
Question 2 - Select One
According to the principle of conservation of linear momentum if the external force acting on the system is zero, the linear momentum of the system will remain conserved. It means if the centre of mass of a system is initially at rest, it will remain at rest in the absence of external force, that is, the displacement of centre of mass will be zero.
A plank of mass $M$ is placed on a smooth horizontal surface. light identical springs, each of stiffness $K$ , are rigidly connected to struts at the end of the plank as shown in Fig. When the springs are in their unextended position, the distance between their free ends is $3 l$ . A block of mass $m$ is placed on the plank and pressed against one of the springs so that it is compressed to $l$ . To keep the block at rest it is connected to the strut means of a light string. Initially, the system is at rest, Now the string is burnt.

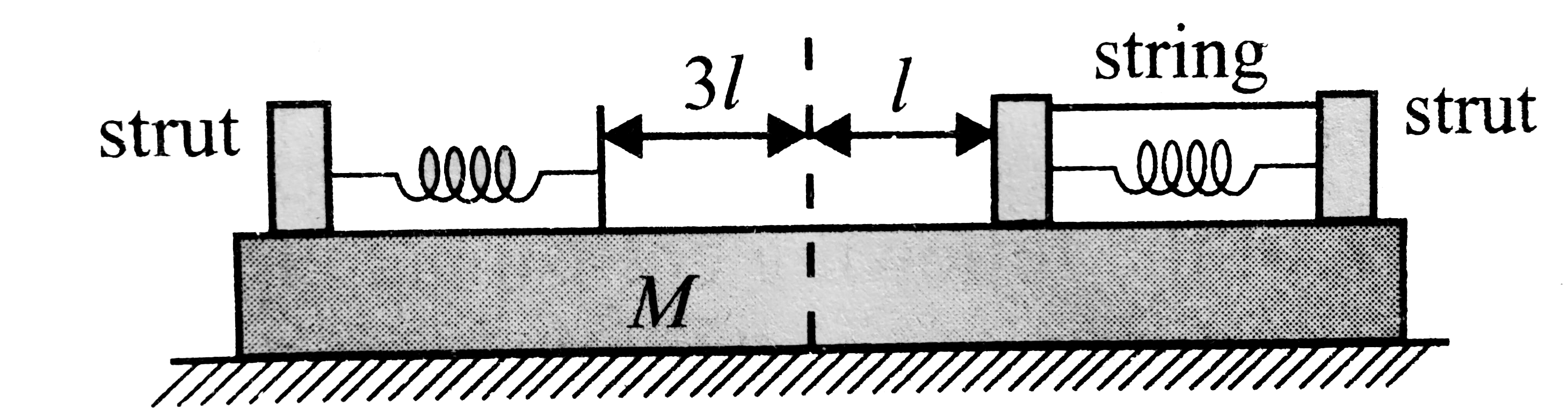
The maximum kinetic energy of the block $m$ is
A $\frac{K m l^{2}}{2 M (M + m)}$
B $\frac{K m l^{2}}{M (M + m)}$
C $\frac{K M l^{2}}{2 (M + m)}$
Dnone of these
Question 3 - Select One
If net external force acting on the system is zero then
AVelocity of the individual particle of the system will remain constant
BVelocity of the center of mass of the system will remain constant
CVelocity of the center of mass of the system will be zero
DVelocity of the individual particle of the system will be zero