A spaceship is sent to investigate a planet of mass $M$ and radius $R$ . While hanging motionless in space at a distance $5 R$ from the centre of the planet, the spaceship fires an instrument package of mass $m$ , which is much smaller than the mass of the spaceship. For what angle $θ$ will the package just graze the surface of the planet?

Video Solution

Text Solution

Verified by Experts

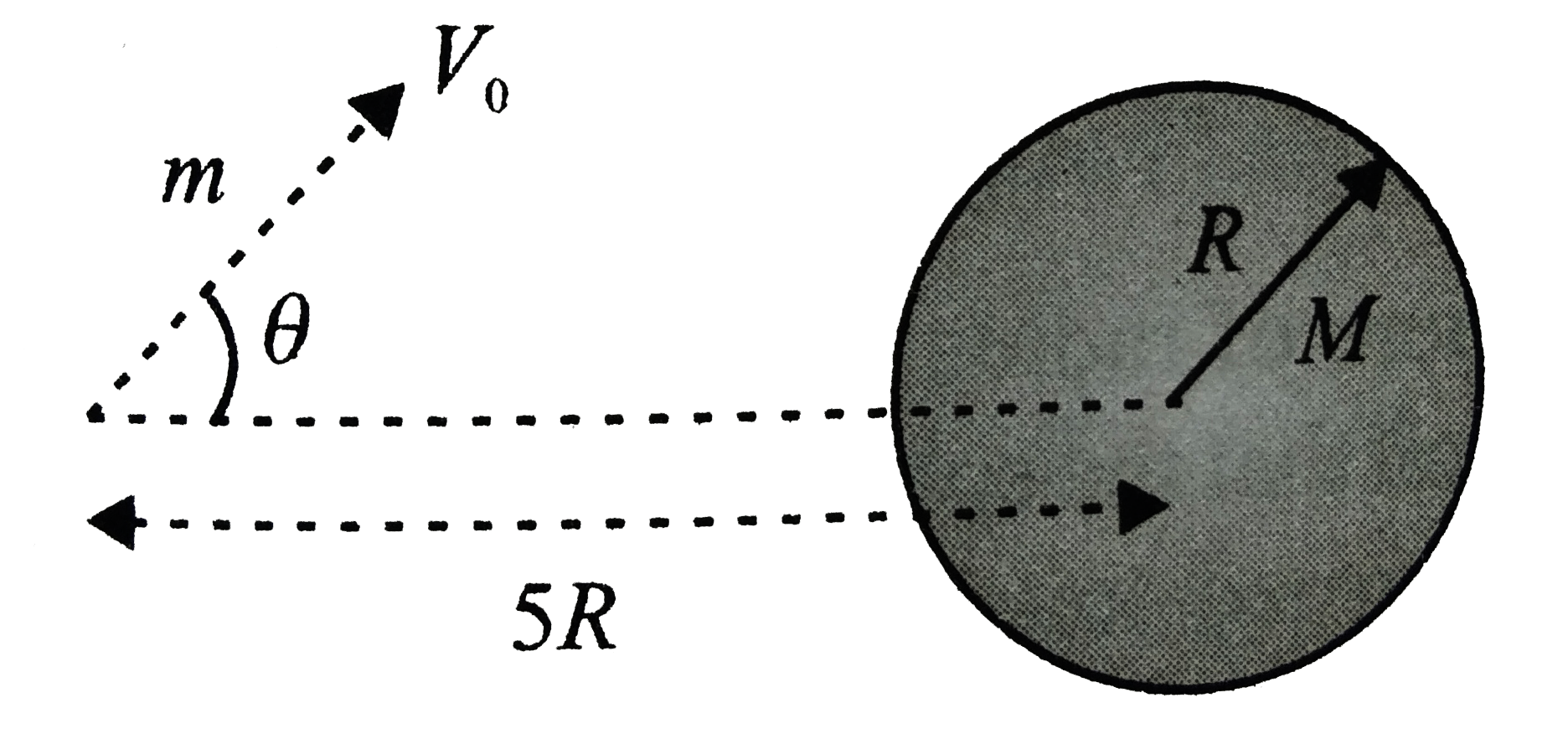
The correct Answer is: ${sin}^{- 1} (\frac{1}{5} \sqrt{1 + \frac{8 G M}{5} v_{0}^{2} R}))$

Updated on:21/07/2023

A particle at a distance r from the centre of a uniform spherical planet of mass M radius R (ltr) has a velocity of magnitude v.
Afor $0 < v < \sqrt{\frac{G M}{r}}$ trajectory may be ellipse
Bfor v $= \sqrt{\frac{G M}{r}}$ trajectory may be ellipse
Cfor $\sqrt{\frac{G M}{r} < v < \sqrt{\frac{2 G M}{r}}}$ trajectory may be ellipse.
Dfor v =sqrt((GM)/r trajectory may be circle
The angular velocity of rotation of a planet of mass M and radius R, at which the matter start to escape from its equator is
A $\sqrt{\frac{2 G M}{R^{3}}}$
B $\sqrt{\frac{2 G M}{R}}$
C $\sqrt{\frac{2 G^{2} M}{R}}$
D $\sqrt{\frac{2 G M^{2}}{R}}$
A space vehicle approaching a planet has a speed v when it is very far from the planet At that moment tangent of its trajectory would miss the centre of the planet by distance R if the planet has mass M and radius r what is the smallest value of R in order that the resulting orbit of the space vehicle will just miss the surface of the planet?
A $R = \frac{r}{v} {[v^{2} + \frac{2 G M}{r}]}^{1 / 2}$
B $R = v r [1 + \frac{2 G M}{r}]$
C $R = \frac{v}{r} [v^{2} + \frac{2 G M}{r}]$
D $R = \frac{2 G M v}{r}$