Boiling point of water 750 mm Hg is 99.63∘C. How much sucrose is to be added to 500 g of water such that it boils at 100∘C.
[Kb for water is 0.52 K kg mol−1]
i) Since boiling point is changing, apply the formula for elevation in boiling point,
ΔTb=Kbm
ii) m=WBMB.WA
So, ΔTb=Kb.WBMB×WA
Or WB=ΔTb×MB×WAKb
iii) Find ΔTb as ΔTb=Tb=Tb−T0b
Tb= Boiling point of solution
T0b = Boiling point of pure solvent Solution in Telugu
Mass of water (WA)=500 g=0.5 kg
Elevation in boiling point (ΔTb)=100∘C−99.63∘C=0.37∘C=0.37 K
Molal elevation constant (Kb)=0.52 K kg mol−1
Molar mass sucrose C12H22O11
(MB)=(12×12)+(22×1)+(16×11)
=342 g mol−1
WB=MB×ΔTB×WAKb
=(342 g mol−1)×(0.37 K)×(0.5 kg)(0.52 K kg mol−1)=121.7 g
Topper's Solved these Questions
- SOLUTIONS BOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - SOLUTIONS EXERCISE - LONG ANSWER QUESTIONS
10 Videos
- SOLID STATEBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - SOLID STATEEXERCISE - INTEXT QUESTIONS
27 Videos
- SURFACE CHEMISTRYBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - SURFACE CHEMISTRYEXERCISE - INTEXT QUESTIONS
8 Videos
Similar Questions
An aqueous solution of 2% non volatile solute exerts a pressure of 1.004 bar at the normal boiling point of the solvent. What is the molecular mass of the solute ?
View SolutionWhy are solids rigid ?
View SolutionWrite any two characteristics of Chemisorption.
View SolutionWhen 1.22gC6H5COOH is added into two solvents, the following data of ΔTb and Kb are obtained:
i. In 100gCH3COCH3,ΔTb=0.17,Kb=1.7kgKmol−1.
ii. In 100g benzene,ΔTb=0.13 and Kb=2.6kgKmol−1.
Find out the molecular weight of C6H5COOH in both cases and interpret the results.
View SolutionThe boiling point T'_(b) of a solvent becomes T_(b) on addition of X_(1) mole fraction of solute. Heat of vaporisation of solvent is DeltaH_("vap") . The relation between elevation in b.pt. DeltaT_(b)=(T_(b)-T'_(b)) can be given by: (DeltaT_(b))/(T_(b)xxT'_(b))=-(2lnX_(1))/(DeltaH_(vap)) A graph plotted between log_(10)X_(1) vs (1)/(T_(b)) gives:
View SolutionConsider equimolal aqueous solutions of NaHSO_(4) and NaCl with DeltaT_(b) and DeltaT'_(b) as their respective boiling point elevation . The value of underset(m to1)("lim") (DeltaT_(b))/(DeltaT'_(b)) will be :
View SolutionFigure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
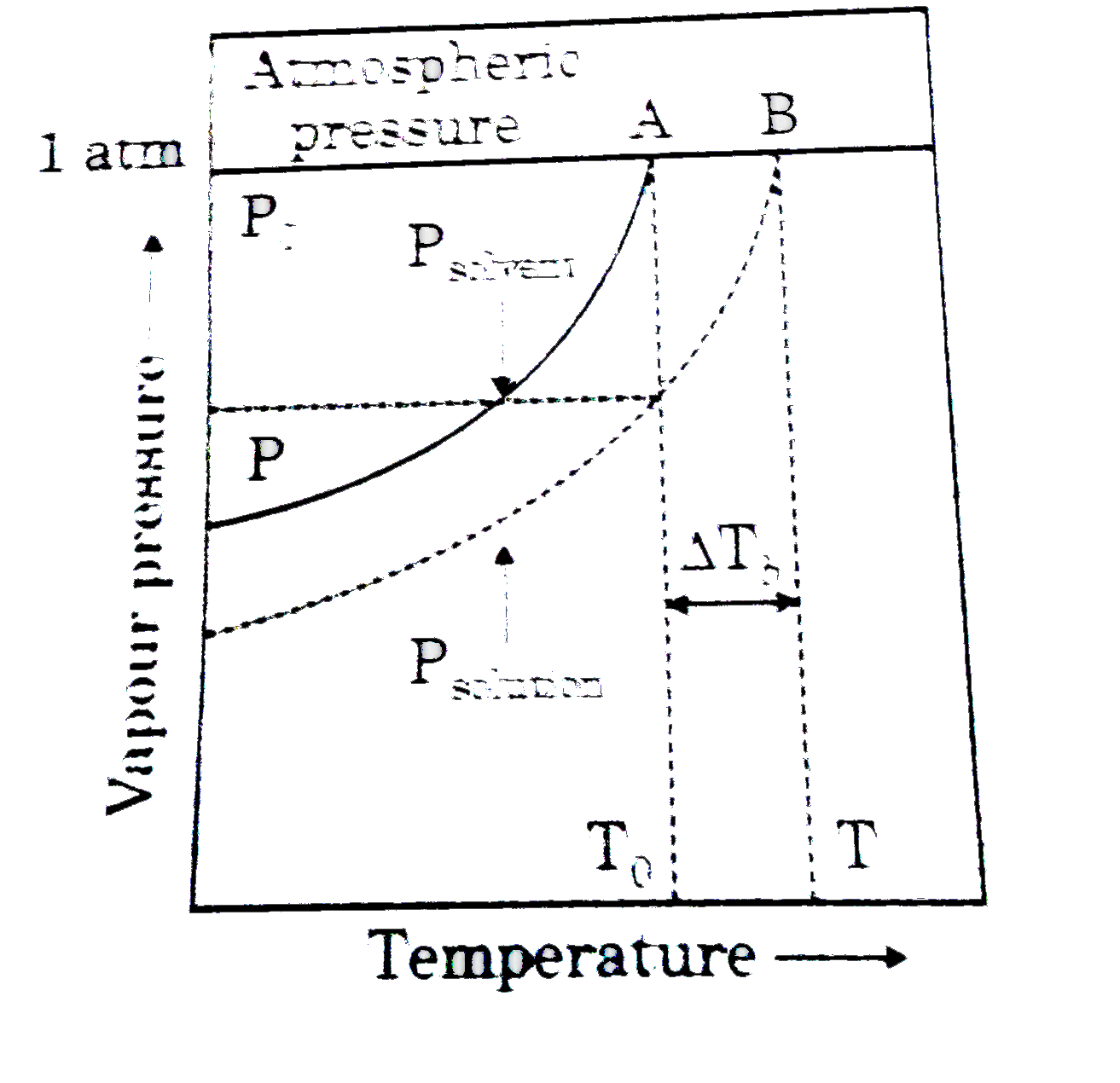
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:
View SolutionThe elevation in boiling point of a solution of 10g of a binary electrolyte (molecular mass 100) in 100g of water is DeltaT_(b) . The value of K_(b) for water is :
View SolutionAfter adding non-volatile solute, freezing point of water decreases to −0.186∘C. Calculate ΔTb if :
Kf=1.86K kg mol−1andKb=0.521K kg mol−1
View Solutionयदि ΔTb क्वथनांक में उन्नयन तथा m विलयन की मोललता को दर्शाता हो तो सिद्ध कीजिए कि,
ΔTb=Kb⋅m
View SolutionCalculate the mass of ascorbic acid (Vitamin C, C6H8O6) to be dissolved in 75g of acetic acid to lower its melting point by 1.5∘C.Kf=3.9 K kg mol−1.
Since, lowering of melting point is given apply the formula for lowering of melting point, i.e.,
ΔTf=Kf.m
ΔTf=Kf.WBMB×WA or WB=ΔTf.MB.WAKf
View SolutionIf the elevation in boiling point of a solution of 50 g of solute (molar mass =100) in 500 g of water is DeltaT_(b) . The ebullioscopic constant K_(b) of water is equal to ___________.
View Solutionयदि X,Y,Z तीन पदार्थों के अणुभार समान हों तो उनके क्वथनांक Tb तथा Kb में सम्बन्ध स्थापित कीजिए।
तत्वTbKbX1000.68Y270.53Z2530.98
View Solutionअवाष्पशील विलेय मिलाने पर जल का हिमांक बिंदु −0.186∘C कम हो जाता है। यदि Kf=1.86Kkgmol−1 तथा Kb=0.521Kkgmol−1 तो ΔTb होगा:
View Solution1g of a non-volatile non-electrolyte solute is dissolved in 100g of two different solvents A and B whose ebullioscopic constants are in the ratio of 1 : 5. The ratio of the elevation in their boiling points, (DeltaT_(b)(A))/(DeltaT_(b)(B)) is
View Solution
