A long wire with a uniform charge density λ is bent in two configurations shown in fig. Determine the electric field intensity at point O.
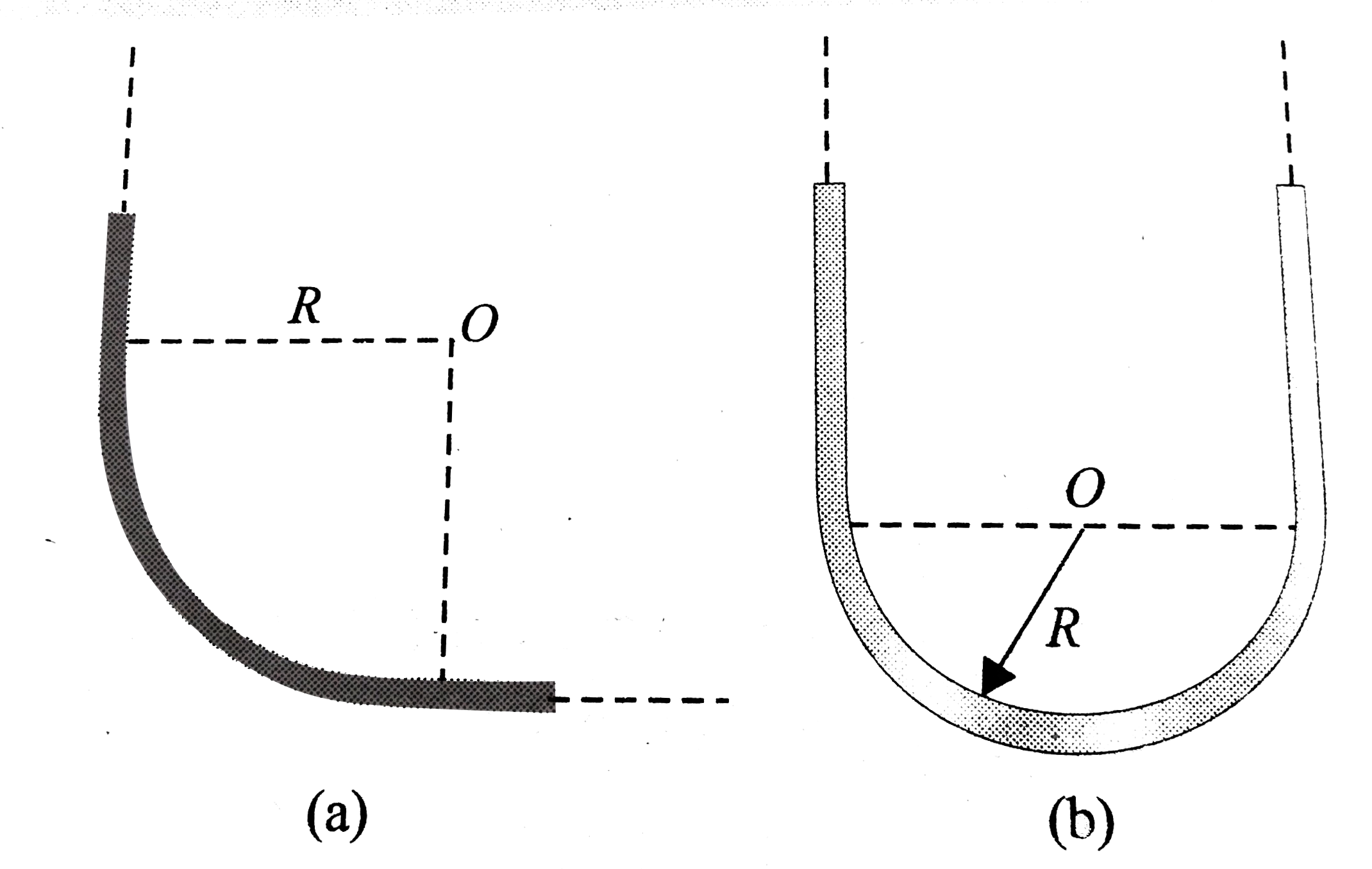
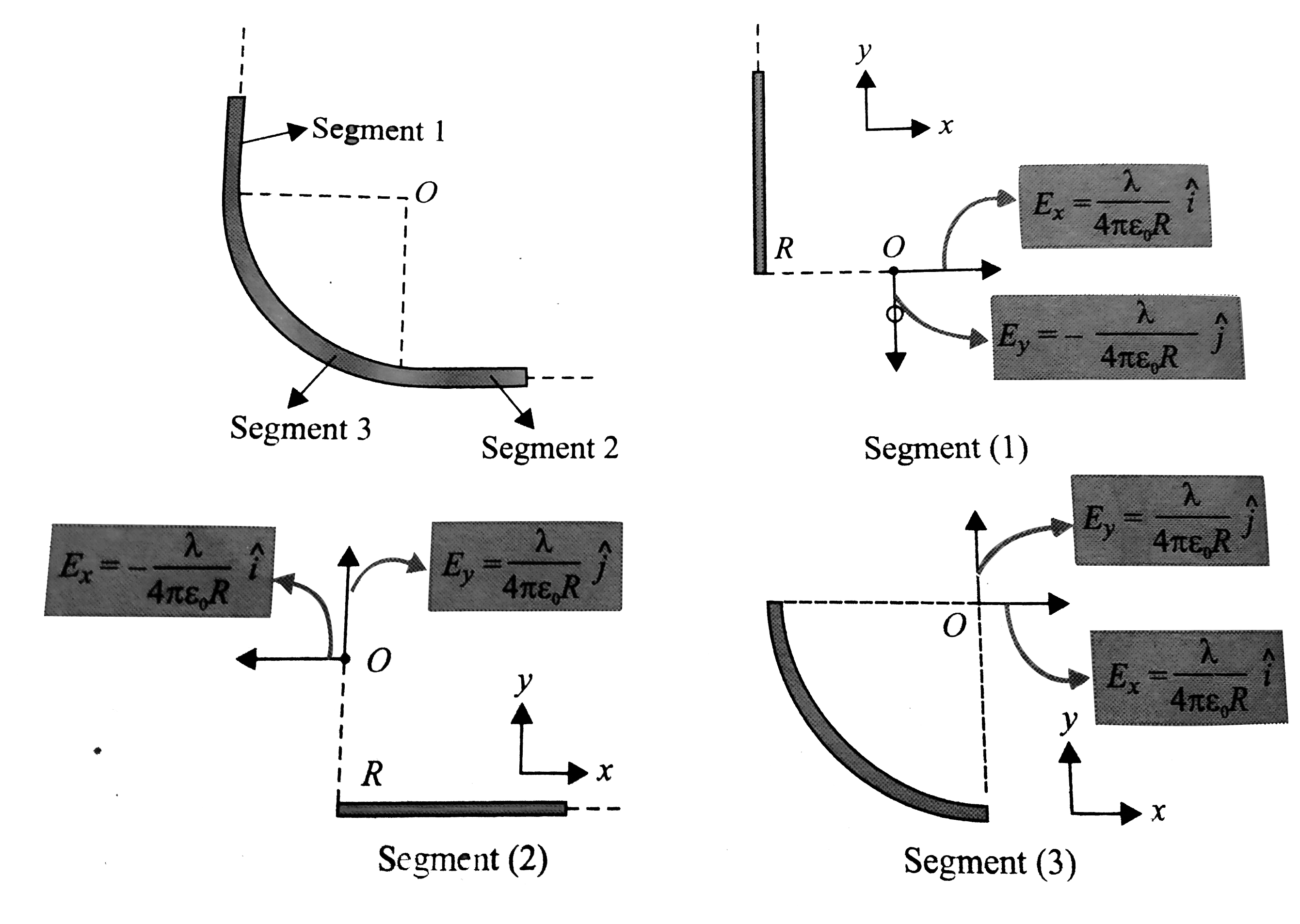
For Fig. Field due to segment 1 is
→E1=[λ4πε_(0)R]ˆi+[−λ4πε_(0)R]ˆj
field dur to segment 2 is
→E2=[λ4πε0R]ˆi+[−λ4πε0R]ˆj
Field due tp quater shape wire segment 3 is
→E3=[λ4πε_(0)R]ˆi+[−λ4πε_(0)R]ˆj(∴θ1=90∘,θ2=0∘)
 ,
, 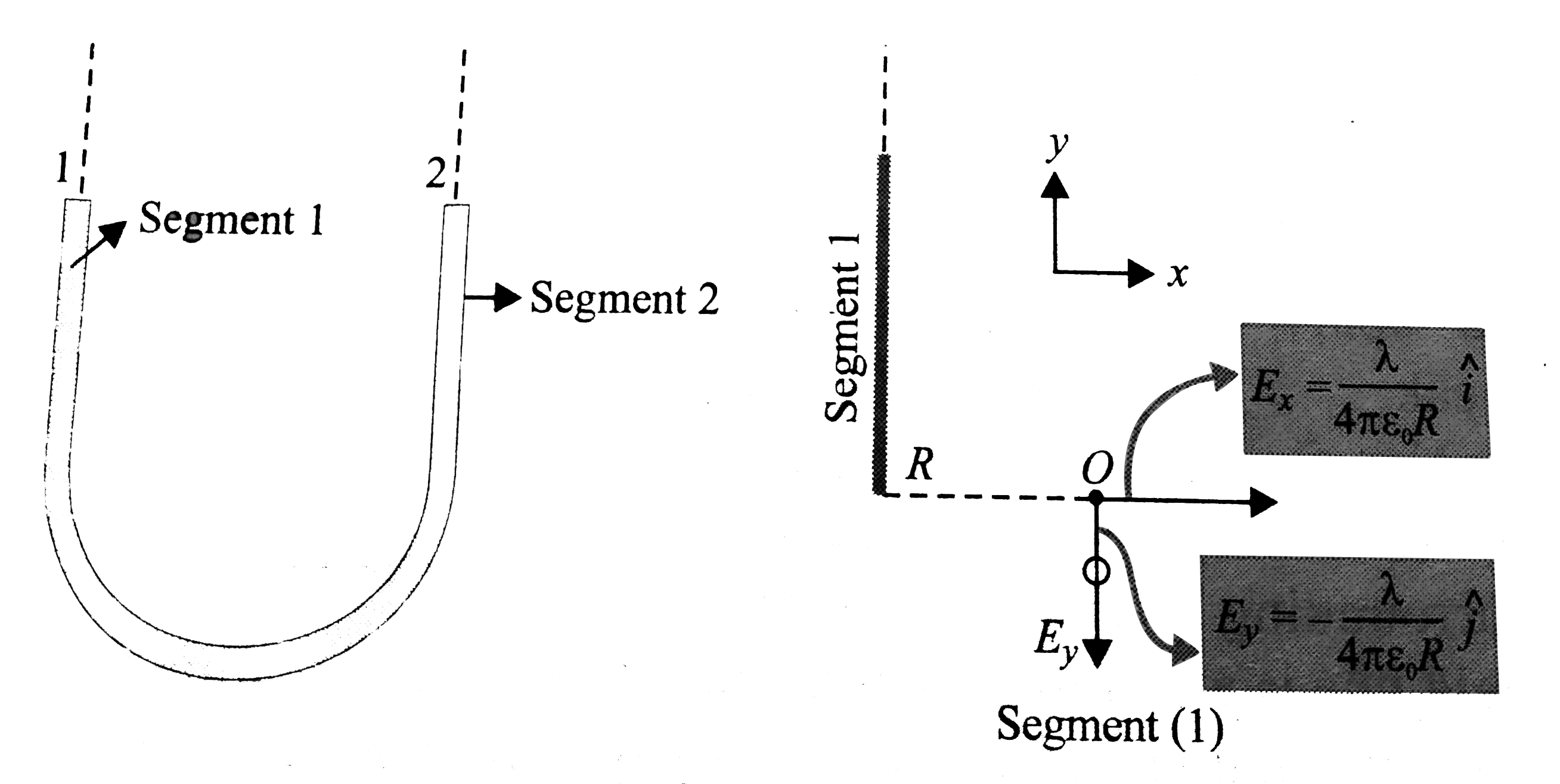
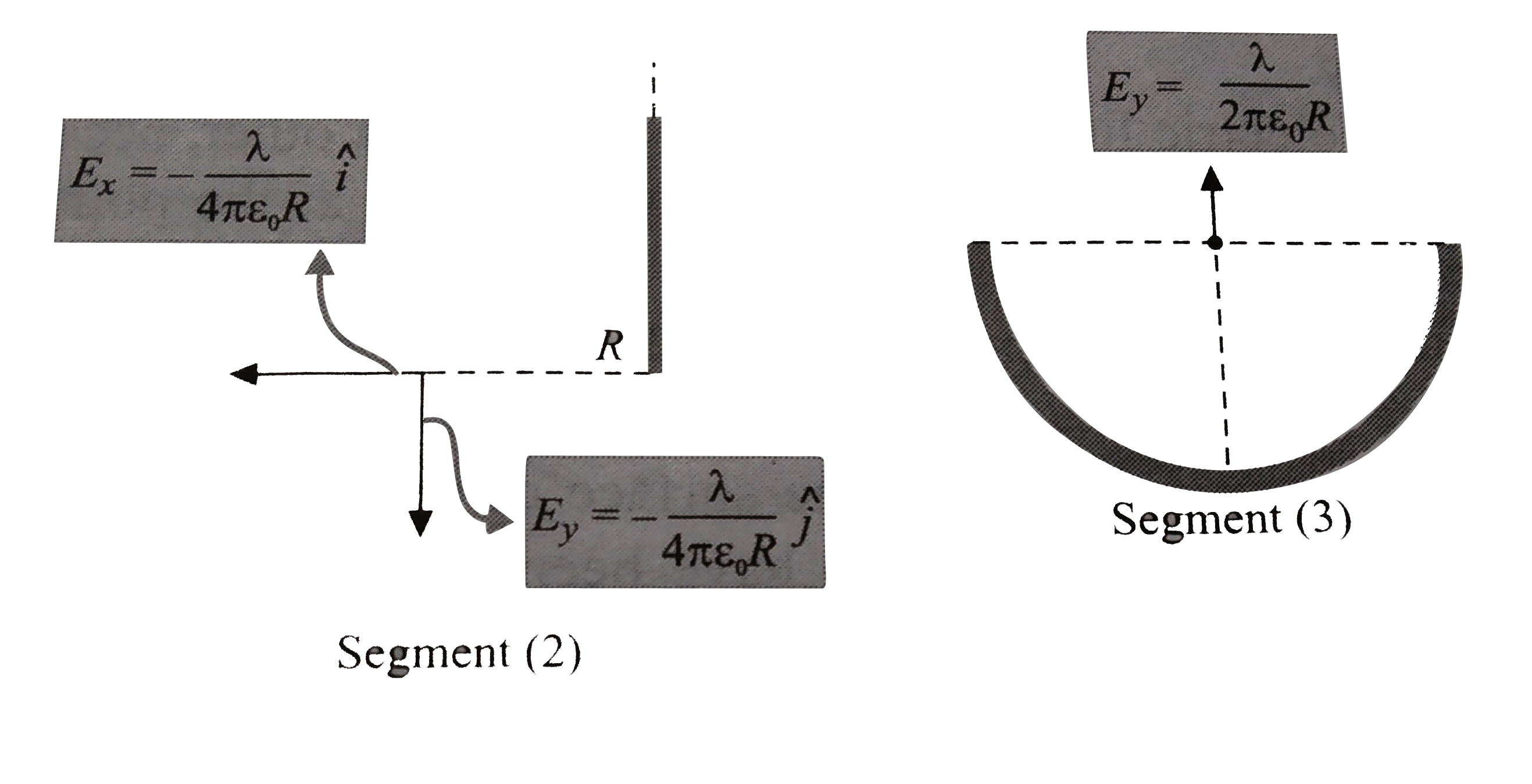 ,
,
The resultant field is the superpositon of the fields due to each part, i.e.
→E=→E1+→E2+→E3 ...(i)
Substituting the values of →E1,→E2, and →E3 in eq. (i) we get,
→E=[λ4πε0R]ˆi+[λ4πε0R]ˆj
∣∣→E1∣∣=[(λ4πε0R)2]+(λ4πε0R)2]1/2=√2λ4πε0R
here, Ex=Ey=λ4πε0R
Hence, the resulant field will make an angle of 45∘ with the axis.
(b). For fig. field due to segment 1 is
→E1=λ4πε0R[ˆi−ˆj]
Field due to segment 2 is
→Ex2=λ4πε0Rˆi
→Ey2=λ4πε0Rˆi
→E2=λ4πε0R[ˆi−ˆj]
Field due to segment 3 is
→E3=λ2πε0Rˆj
from the principle of superposition of electric fields.
→E=→E1+→E2+→E3
=λ4πε0R[ˆi−ˆj]−λ4πε0R[ˆi−ˆj]+λ2πε0R[ˆi−ˆj]=0
Hence, the net field is zero.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples10videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises58videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICSCHAPTER - COMMUNICATION SYSTEMEXERCISE - QUESTION BANK19videos
COMMUNICATION SYSTEM
- BOOK - CENGAGE PHYSICSCHAPTER - Current ElectricityEXERCISE - QUESTION BANK40videos
Current Electricity
Similar Questions
Figure shows a long wire having uniform charge density λ as shown in figure. Calculate electric field intensity at point P.
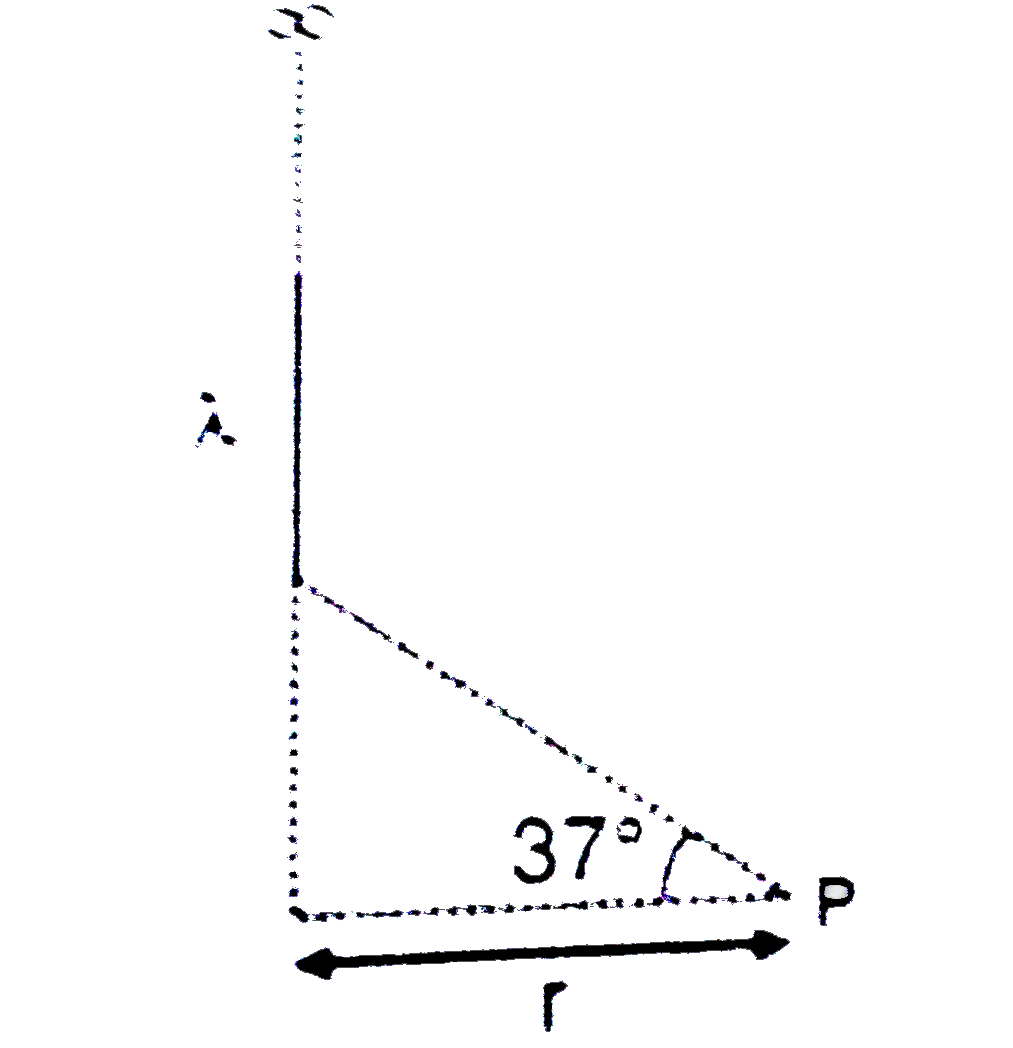 View Solution
View SolutionA very long charged wire (lying in the xy plane ) which is having a linear charge density λ is having one of its end at a point P as shown in figure . What is electric field intensity at point Q :
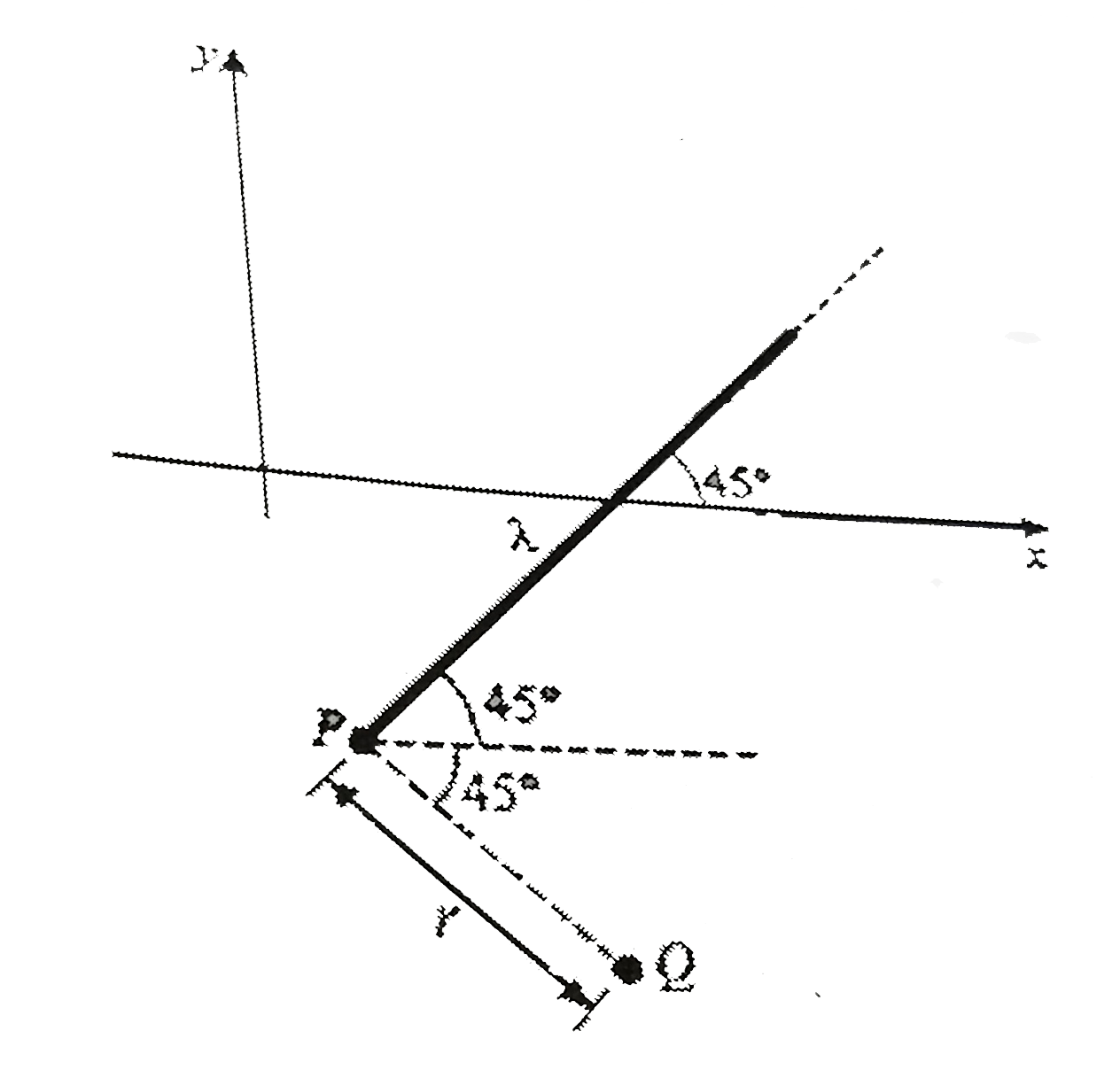 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
An infinitely long wire with linear charge density +λ is bent and the two parts are inclined at angle 30∘ as shown in fig, the electric field at point P is :
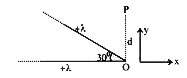 Aλ4π∈0d[(1+√3)ˆi+ˆj]Bλ4π∈0d[(1−√3)ˆi+2ˆj]Cλ4π∈0d[(1+√3)ˆi−2ˆj]Dλ4π∈0d[(1+√3)ˆi+2ˆj]
Aλ4π∈0d[(1+√3)ˆi+ˆj]Bλ4π∈0d[(1−√3)ˆi+2ˆj]Cλ4π∈0d[(1+√3)ˆi−2ˆj]Dλ4π∈0d[(1+√3)ˆi+2ˆj] - Question 2 - Select One
In the diagram shown electric field intensity will be zero at a point.
 ABetwee -q and +2q chargeBTowards +2 cahrge on the lineCAway from line towards +2q chargeDAway from line towards -charge
ABetwee -q and +2q chargeBTowards +2 cahrge on the lineCAway from line towards +2q chargeDAway from line towards -charge - Question 3 - Select One
Two plane sheets of charge densities +σand−σ are kept in air as shown in Fig. What are electric field intensities at points A and B ?
 Aσε0,0B0,0Cσε0,σε0D0,σε0
Aσε0,0B0,0Cσε0,σε0D0,σε0
(i) Two infinitely long line charges each of linear charge density λ are placed at an angle θ as shown in figure. Find out electric field intensity at a point P, which is at a distance x from point O along angle bisector of line charges.
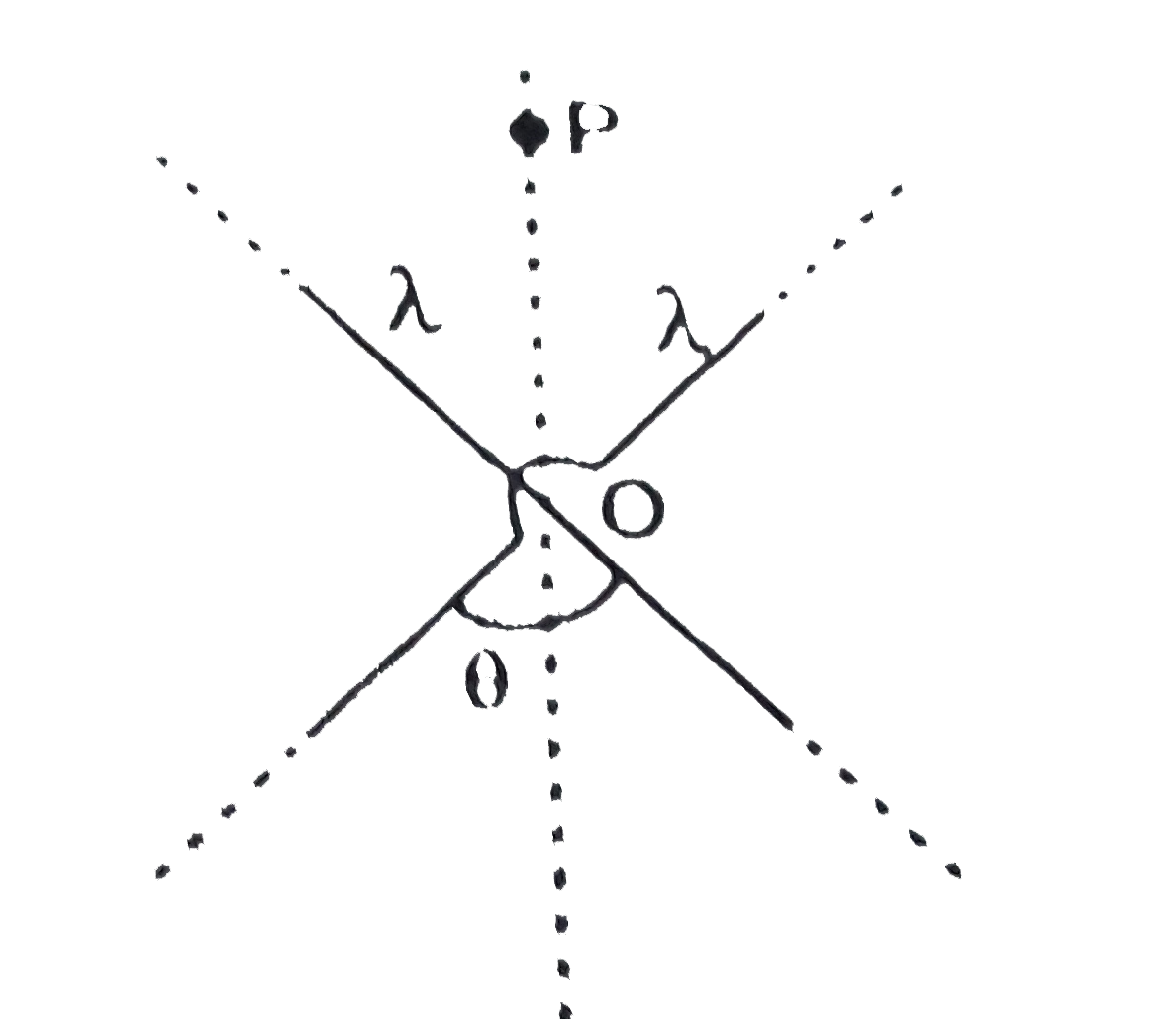
(ii) Repeat the above question if the line charge densities are λ and −λ. as shown in figure.
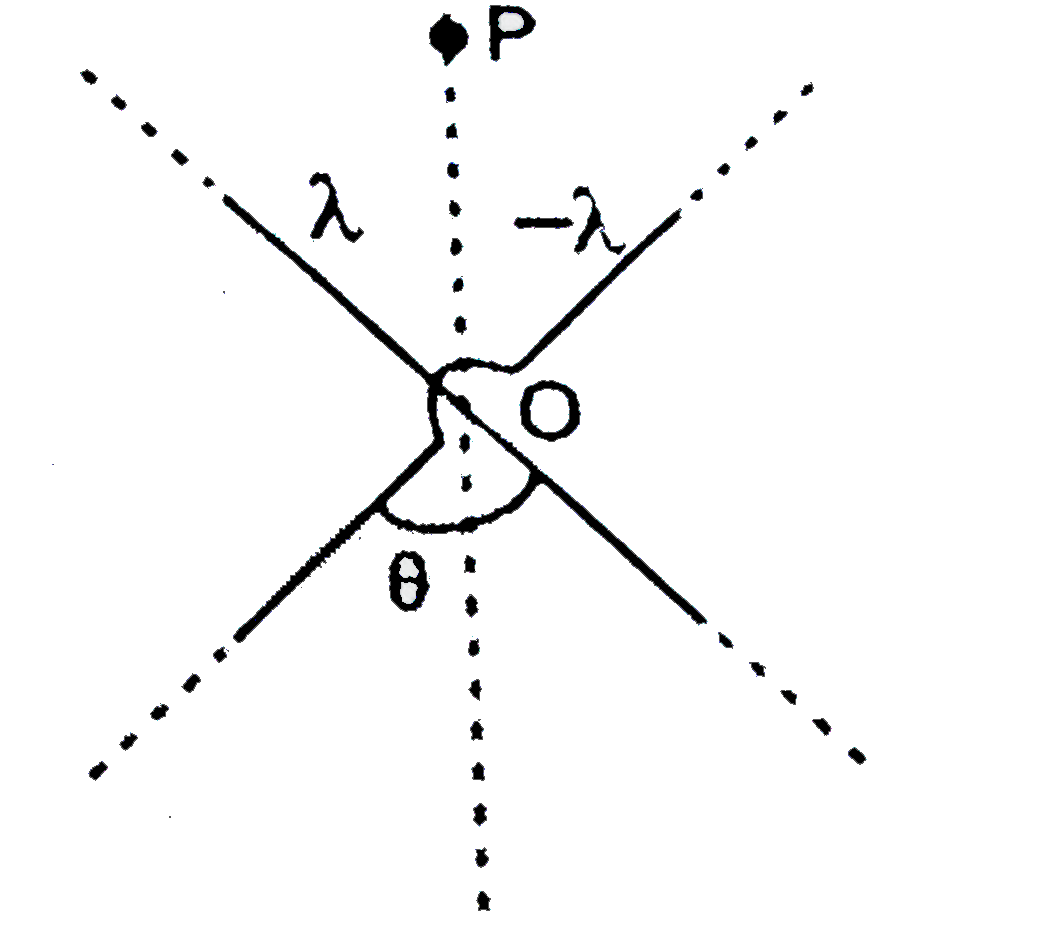 View Solution
View SolutionIf three infinite charged sheets of uniform surface charge densities σ,2σ and −4σ are placed as shown in figure, then find out electric field intensities at points A, B, C and D.
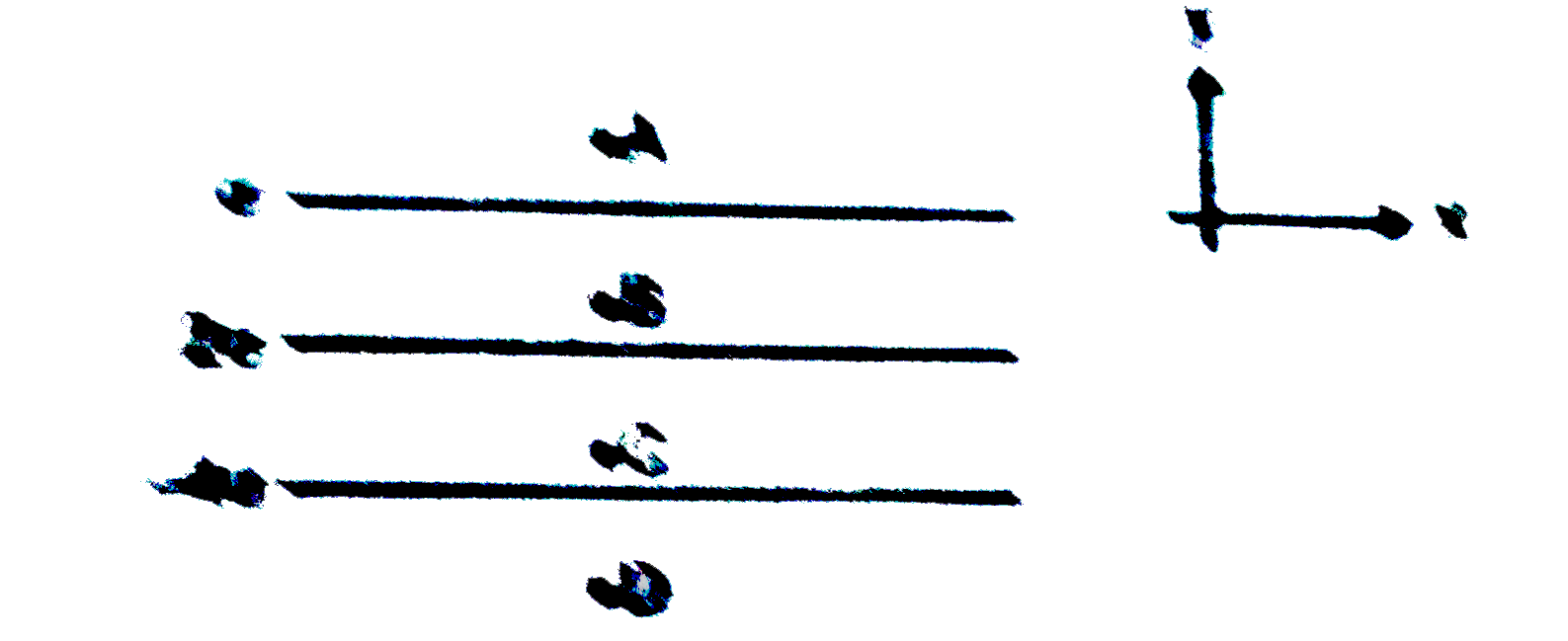 View Solution
View SolutionShown in Fig. is a conductor carrying current I. Find the magnetic field intensity at point O.
View SolutionAn infinitely long line charge is bent in U shape as shown in figure. The semicircular part has radius R and linear charge density is λC/m. Using the results obtained in last two problems, calculate the electric field intensity at centre of the circle (point O)
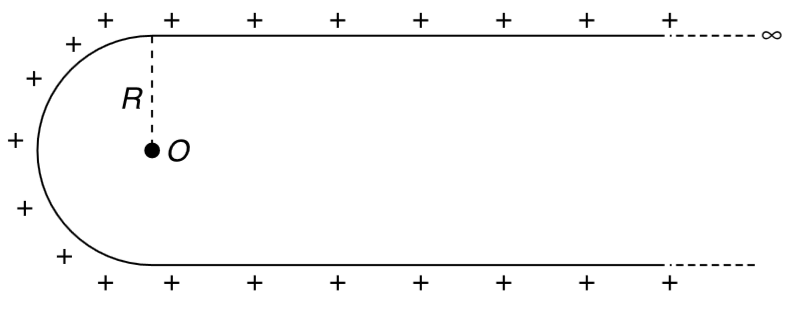 View Solution
View SolutionA long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is
 View Solution
View Solution

