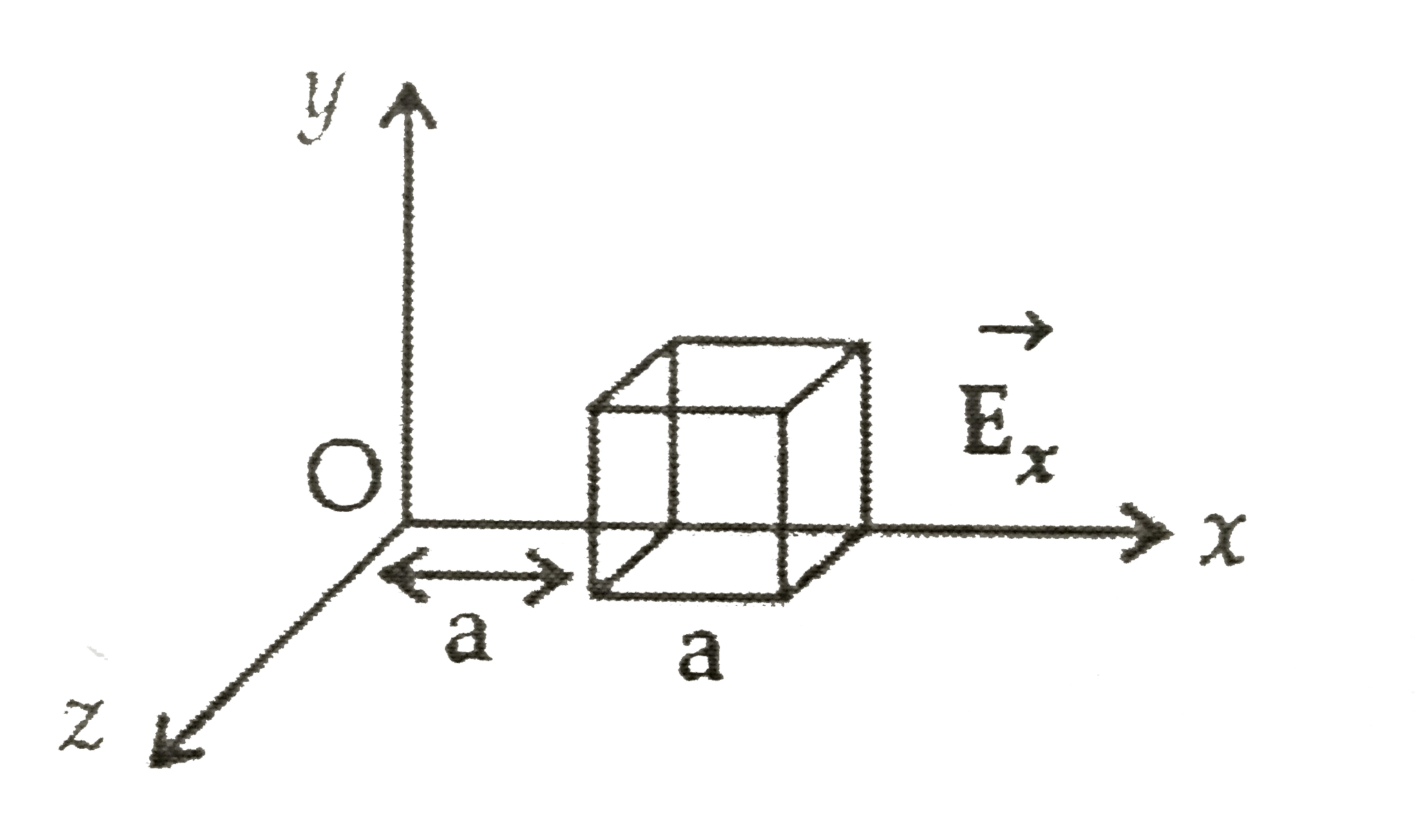
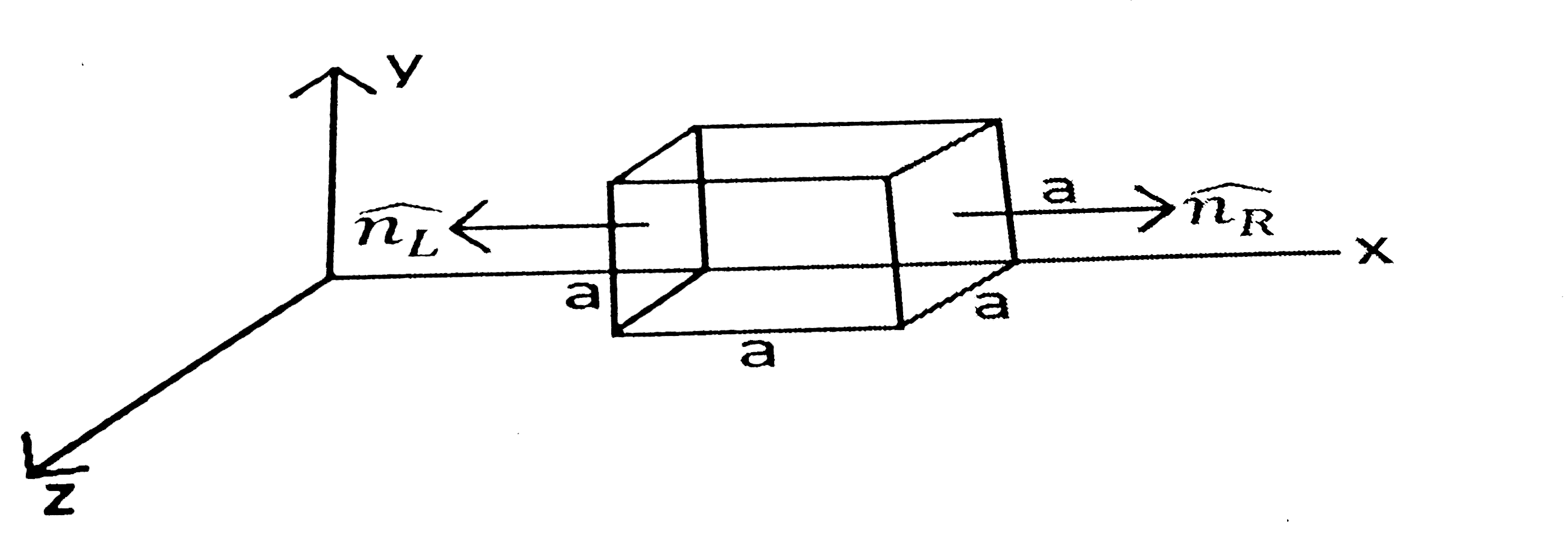
A cube of side a is placed such that the nearest face , which is parallel to the yz plane , is at a distance a from the origin . The electric field components are
Ex=αx1/2,Ey=Ez=0.
The flux ϕE through the cube is

2√2αa5/2
αa5/2
(√2−1)αa5/2
zero
The correct Answer is:C
ϕ1=E1a2=−αa1/2a2=−αa5/2
and ϕ2=E2a2=α(2a)1/2a2=√2αa5/2
ϕnet=(√2−1)αa5/2
Using the Gauss theorem , we get
ϕ=q∈ε0
or q∈=ϕε0=(√2−1)ε0αa5/2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Subjective type7videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct8videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger8videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.514videos
ELECTRIC POTENTIAL
Similar Questions
Define electric flux and write its SI unit . The electric field components in the figure shown are : Ex=αx,Ey=0,Ez=0 where α=100NCm.
Calculate the charge within the cube , assuming a = 0.1 m .
 View Solution
View SolutionThe electric field componets due to a charge inside the cube of side 0.1m are Ex=αx, where α=500(N/C)m−1,
Ey=0,Ez=0. Calculate the flux through the cube and the charge inside the cube.View Solution
Knowledge Check
- Question 1 - Select One
A cube of side a is placed such that the nearest face , which is parallel to the yz plane , is at a distance a from the origin . The electric field components are
Ex=αx1/2,Ey=Ez=0.
The charge within the cube is
 A√2αε0a5/2Bαε0a5/2C(√2−1)αε0a5/2Dzero
A√2αε0a5/2Bαε0a5/2C(√2−1)αε0a5/2Dzero - Question 2 - Select One
Due to a charge inside a cube the electric field is Ex=600x1/2,Ey=0,Ez=0. The charge inside the cube is (approximately):
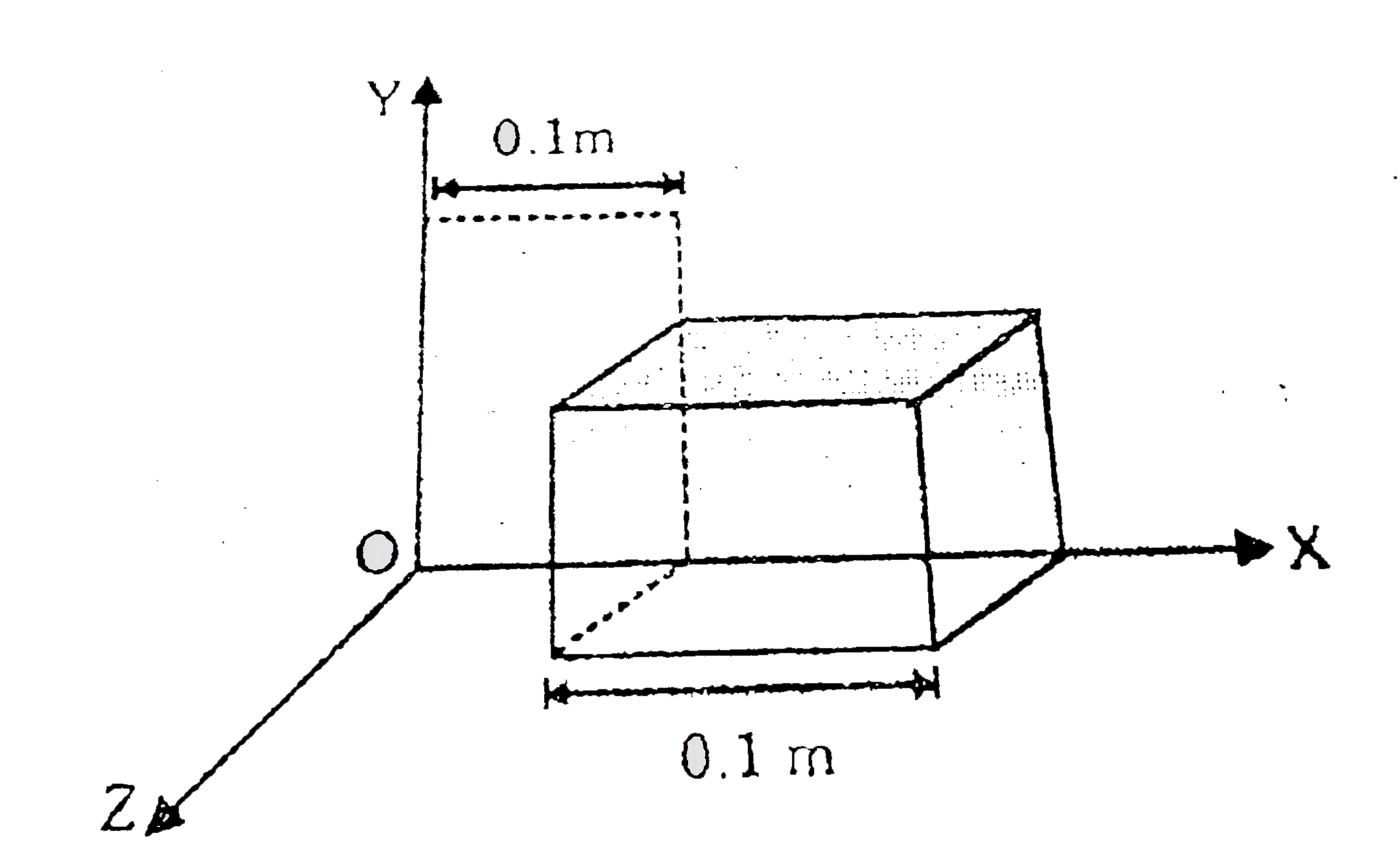 A600μCB60μCC7μμCD6μμC
A600μCB60μCC7μμCD6μμC - Question 3 - Select One
Due to a charge inside the cube, the electric field is: Ex = 600x, Ey = 0, Ez = 0. The charge inside the cube is nearly -
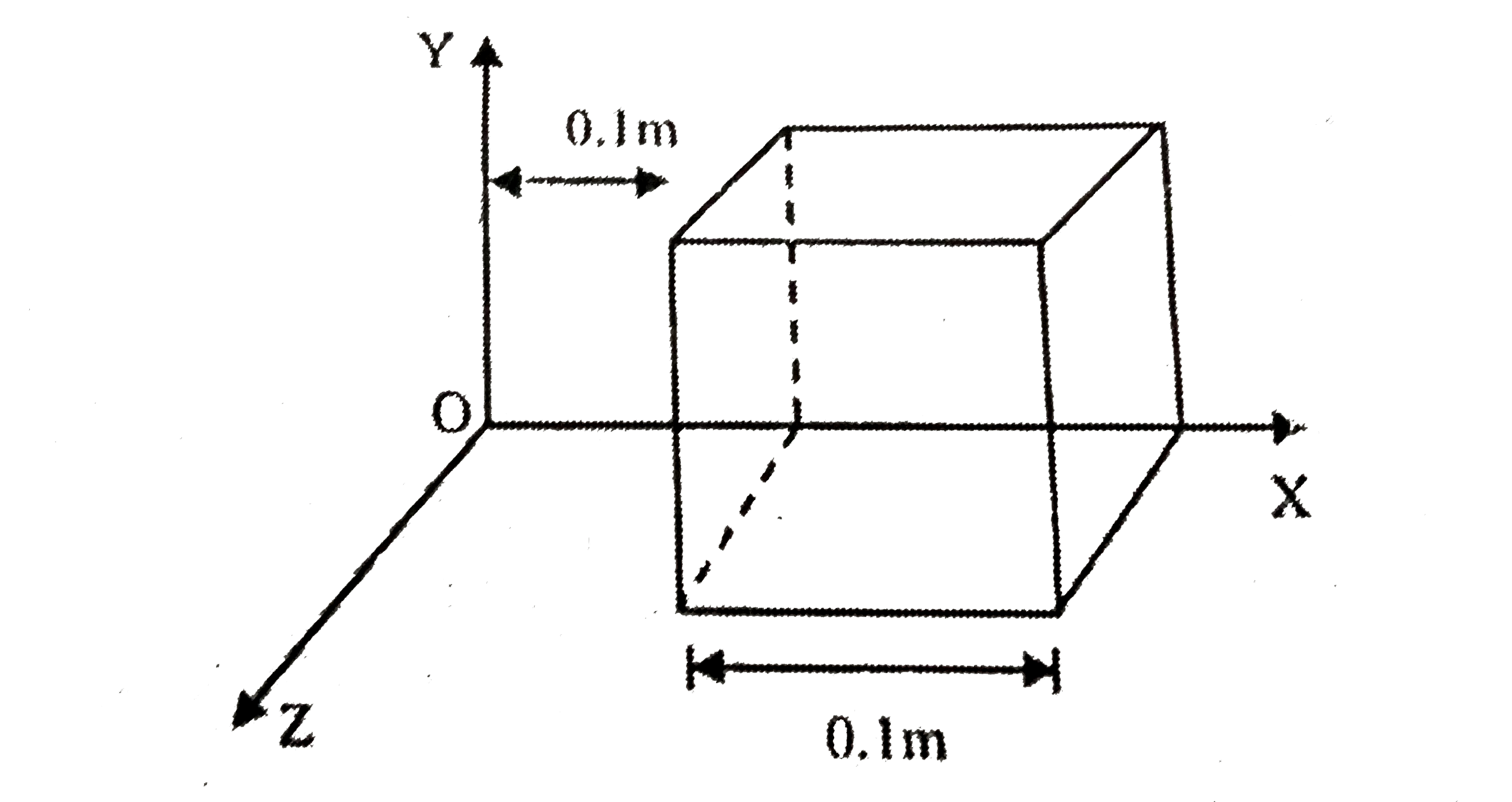 A600μCB60μCC53μCD6μC
A600μCB60μCC53μCD6μC
Using Gauss’s law, derive expression for intensity of electric field at any point near the infinitely long straight uniformly charged wire.
The electric field components in the following figure are Ex=αx,Ey=0,Ez=0, in which α=400N/C m. Calculate (i) the electric flux through the cube, and (ii) the charge within the cube assume that a = 0.1m.
 View Solution
View SolutionA cube of side a is placed in a uniform electric field E=E0ˆi+E0ˆj+E0ˆk. Total electric flux passing through the cube would be
View SolutionThe electric components in the figure are Ex=αx1/2,Ey=0,Ez=0 where α=800N/m2 if a=0.1m is the side of cube then the charge with in the cube is
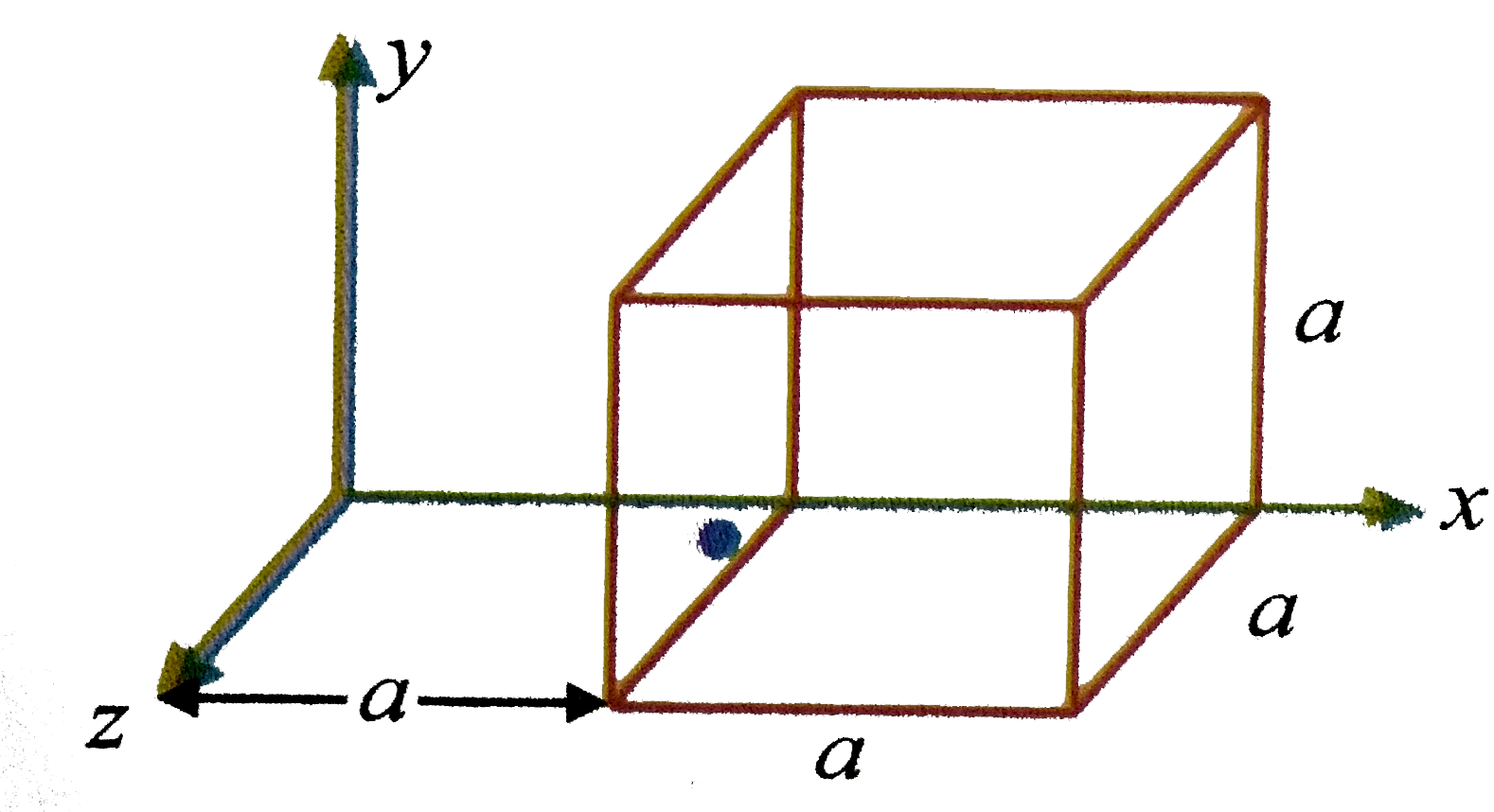 View Solution
View SolutionA cube of side l is p laced in a uniform field E, where E=Eˆi. The net electric flux through the cube is
View SolutionA cube of side l is placed in a uniform field E, where E= Eˆi .The net electric flux through the cube is
View Solution

