A resistance R of therimal coefficient of resistivity $α$ is connected in parallel with a resistance 3R. Having thermal coeffiecinet of resistivity $2 α$ Find the value of $α_{e f f}$

Video Solution

Text Solution

Verified by Experts

The equivalent resistance at $0^{\circ} C$ is
$R_{0} = \frac{R_{10} R_{20}}{R_{10} + R_{20}}$ …(i)
The equivalent resistance at $t^{\circ}$ C is
$R = \frac{R_{1} R_{2}}{R_{1} + R_{2}}$ .....(ii)
But $R_{1} = R_{10} (1 + α t)$ .....(iii)
$R_{2} = R_{20} (1 + 2 α t)$ ....(iv)
and $R = R_{0} (1 + α_{e f t} t)$ ...(v)
Putteing the value of (i), (iii), (iv) and (v) in Eq. (ii), we have
$α_{e f f} = \frac{5}{4} α$

|

Updated on:21/07/2023

Class 12 PHYSICS CURRENT ELECTRICITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A wire of cross-section area $A$ , length $L_{1}$ , resistivity $ρ_{1}$ and temperature coefficient of resistivity $α_{1}$ is connected in series to a second wire of length $L_{2}$ , resistivity $ρ_{2}$ temperature coefficient of resistivity $α_{2}$ and the same area $A$ , so that wires carry same current. Total resistance $R$ is independent of temperature for small temperature change if (Thermal expansion effect is negligible)
A $α_{1} = - α_{2}$
B $ρ_{1} L_{1} α_{1} + ρ_{2} L_{2} α_{2} = 0$
C $L_{1} α_{1} + L_{2} α_{2} = 0$
DNone
Question 2 - Select One
If a resistance $R_{2}$ is connected in parallel with the resistance $R$ in the circuit shown, then possible value of current through $R$ and the possible value of $R_{2}$ will be

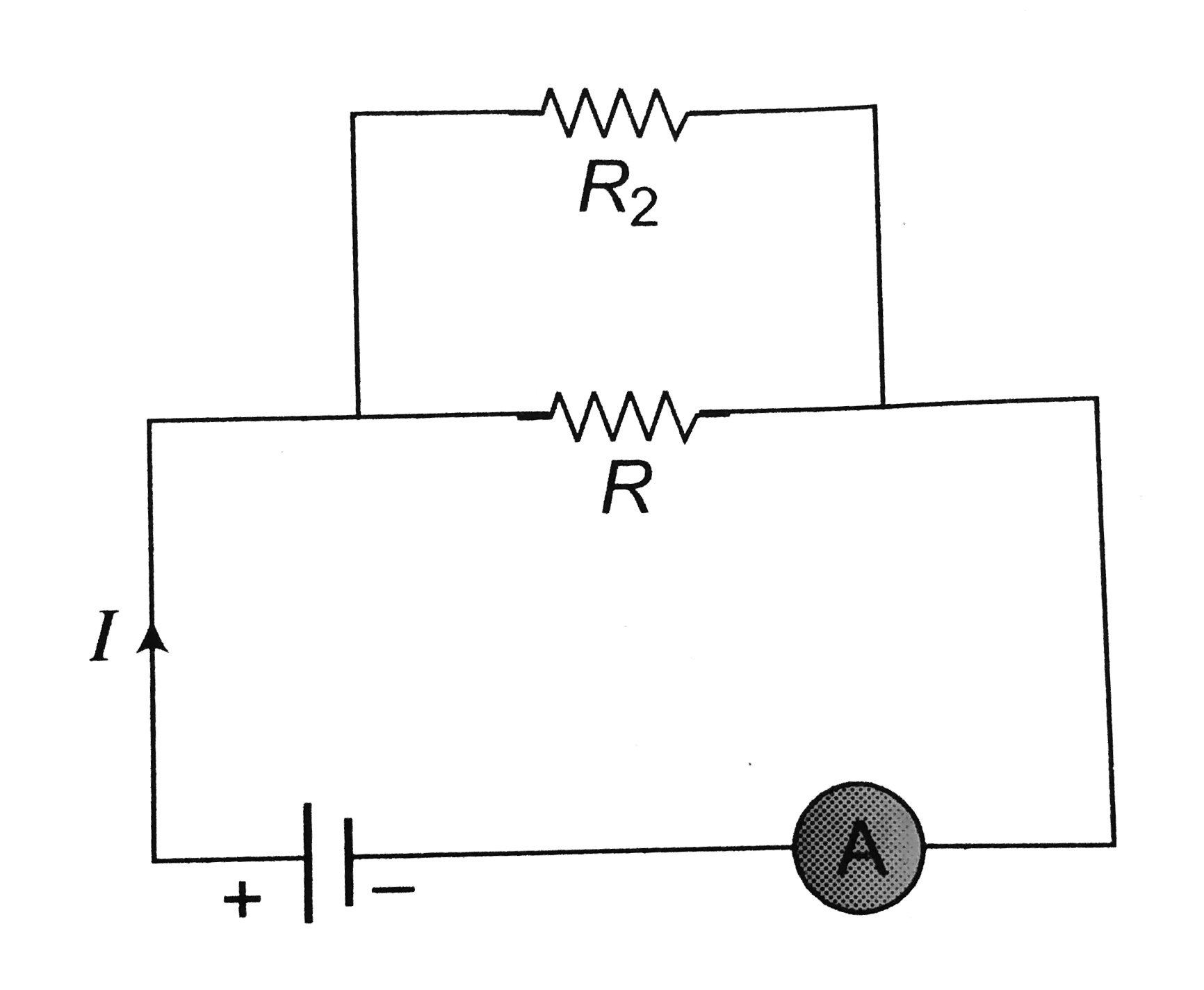A $\frac{I}{3}, R$
B $I .2 R$
C $\frac{I}{3}, 2 R$
D $\frac{I}{2}, R$
Question 3 - Select One
A wire of resistance $R$ is divided in 10 equal parts. These parts are connected in parallel, the equivalent resistance of such connection will be
A $0.01 R$
B $0.1 R$
C $10 R$
D $100 R$

CENGAGE PHYSICS-ELECTRIC CURRENT AND CIRCUIT-Interger

Home
Ask Doubt
Profile