What should be the value of E1/E2 so that current flowing through 7Ω resistor could be increased by short- circuiting the battery of emf E2?
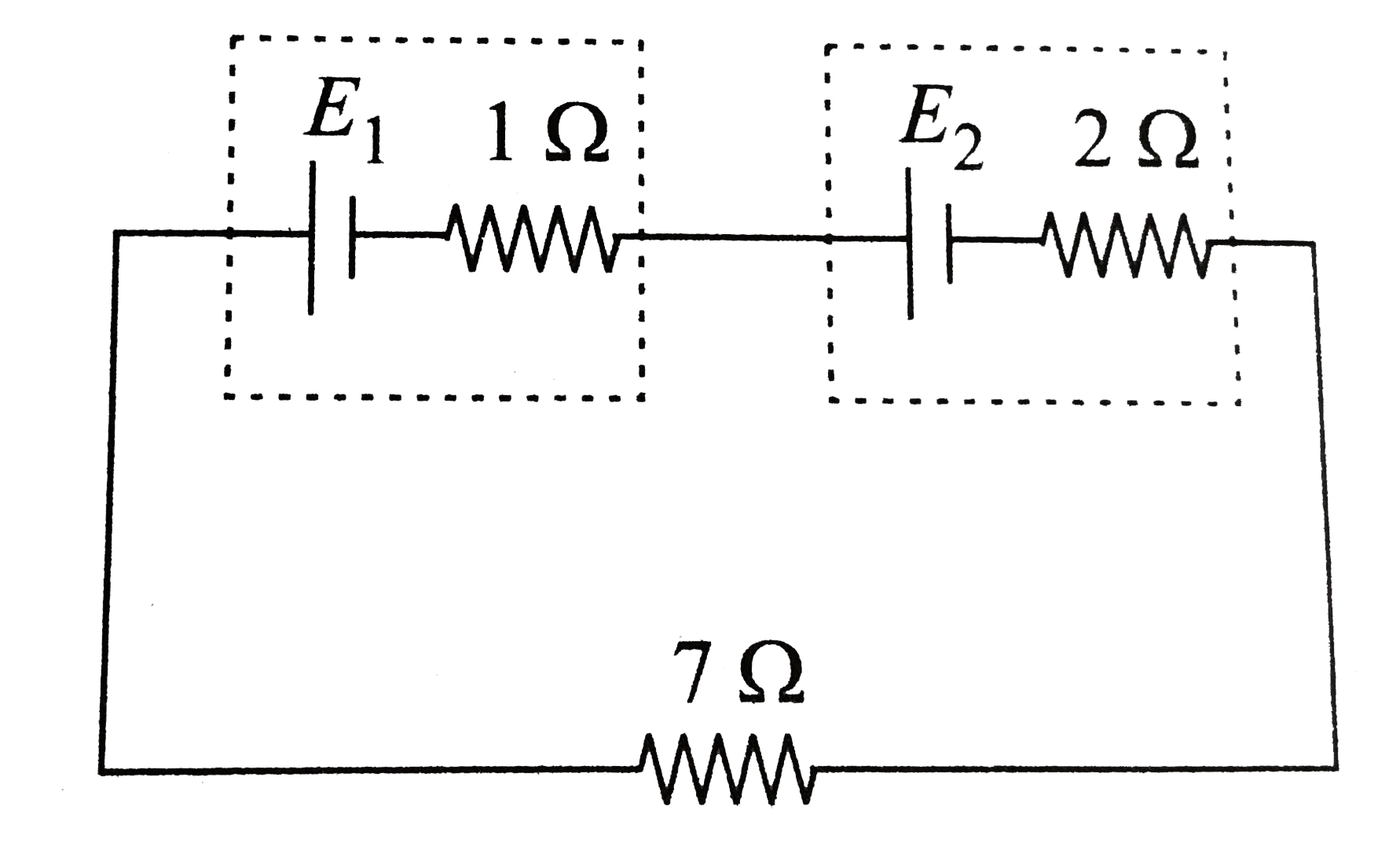
A
E1E2<4
B
E1E2>3
C
E1E2>4
D
E1=E2
Video Solution
Text Solution
The correct Answer is:C
c. Initially I1=E1+E2(1+2+7)=E1+E210
When E2 is shorted I2=E11+7=E18
If I2>I1
E18>E1+E210or54>E1+E2E1
or 54>1+E2E1orE2E1<14
or E1E2>4 .
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The current flowing through 10Ω resistor in the circuit shown in the figure is
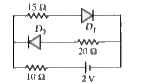 A50 mAB20 mAC40 mAD40 mA
A50 mAB20 mAC40 mAD40 mA - Question 2 - Select One
The current (in A) flowing through 6Ω resistor in the following circuit is:
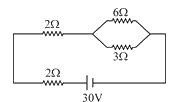 A1.2B1.5C1.7D2
A1.2B1.5C1.7D2 - Question 3 - Select One
Under what condition, the current passing through the resistance R can be increased by short - circuiting the battery of emf E2 The internal resistances of the two batteries are r1andr2, respectively.
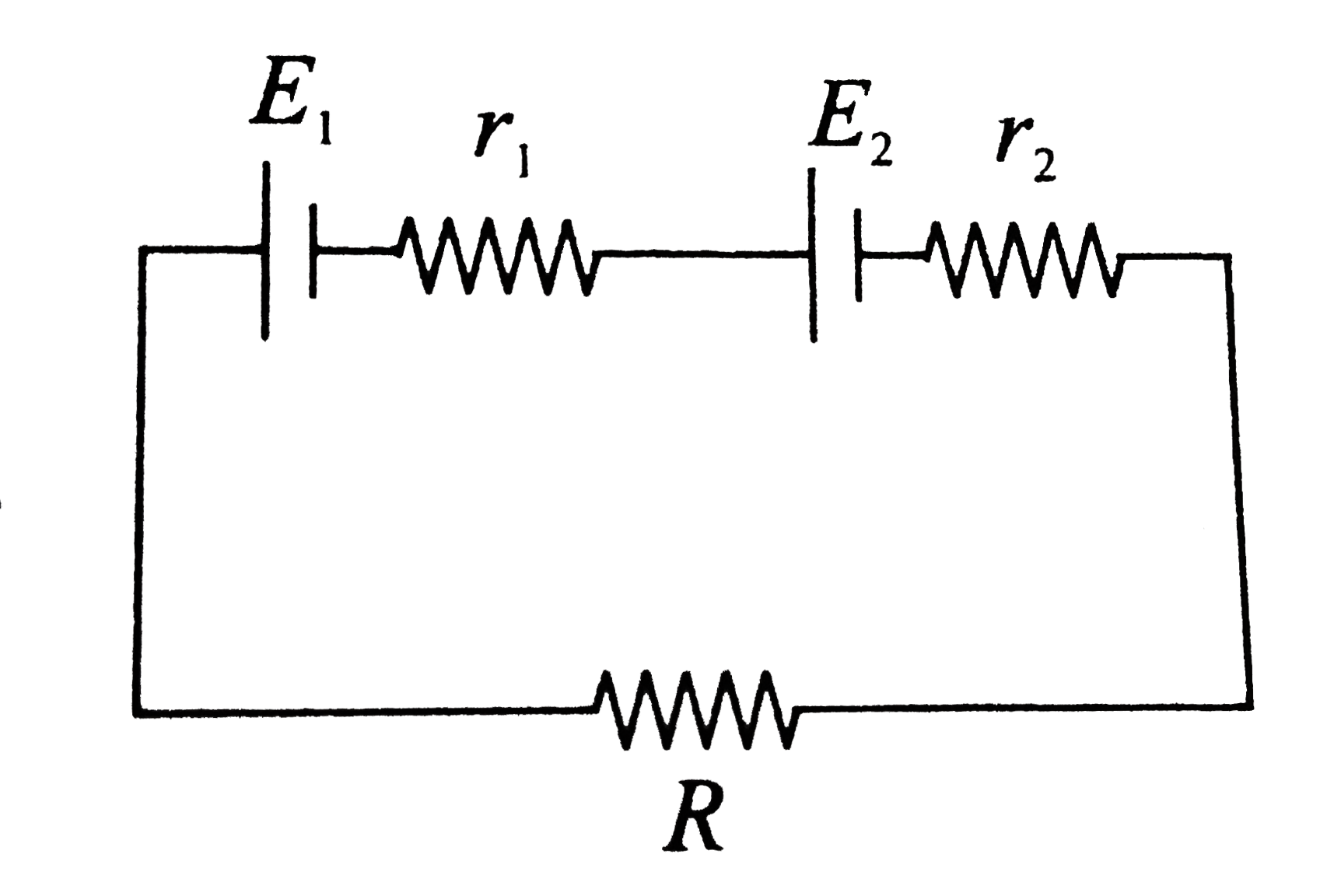 AE2r1>E1(R+r)BE1r2>E2(R+r)CE2r2>E1(R+r)DE1r1>E2(R+r1)
AE2r1>E1(R+r)BE1r2>E2(R+r)CE2r2>E1(R+r)DE1r1>E2(R+r1)
The current I1 (in A) flowing through the 1Ω resistor in the given circuit is
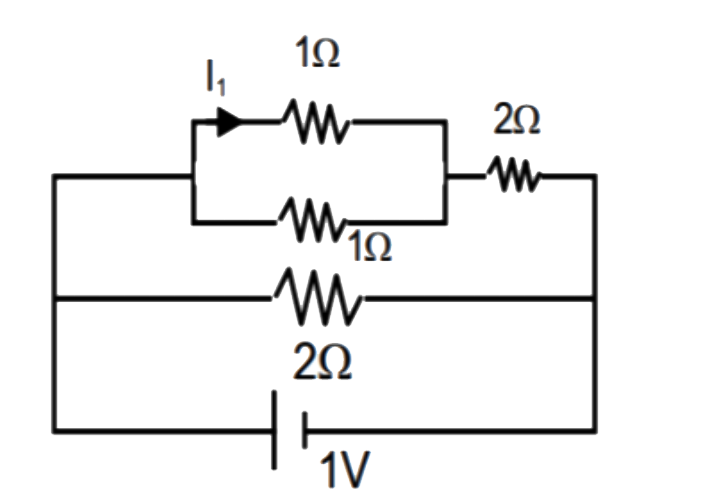 View Solution
View SolutionThe current I1 ( in A ) flowing through 1 Ω resistor in the following circuit is :
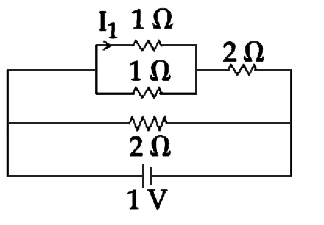 View Solution
View SolutionThe current I1 (in A) flowing through 3Ω resistor in the following circuit is:
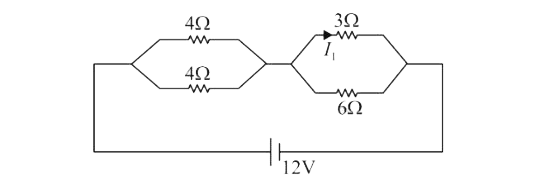 View Solution
View SolutionThe current I1 (in A) flowing through 3Ω resistor in the following circuit is:
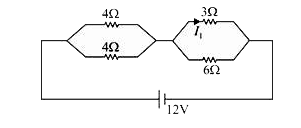 View Solution
View SolutionCurrent passing through 1Ω resistance is zero. Then the emf E is
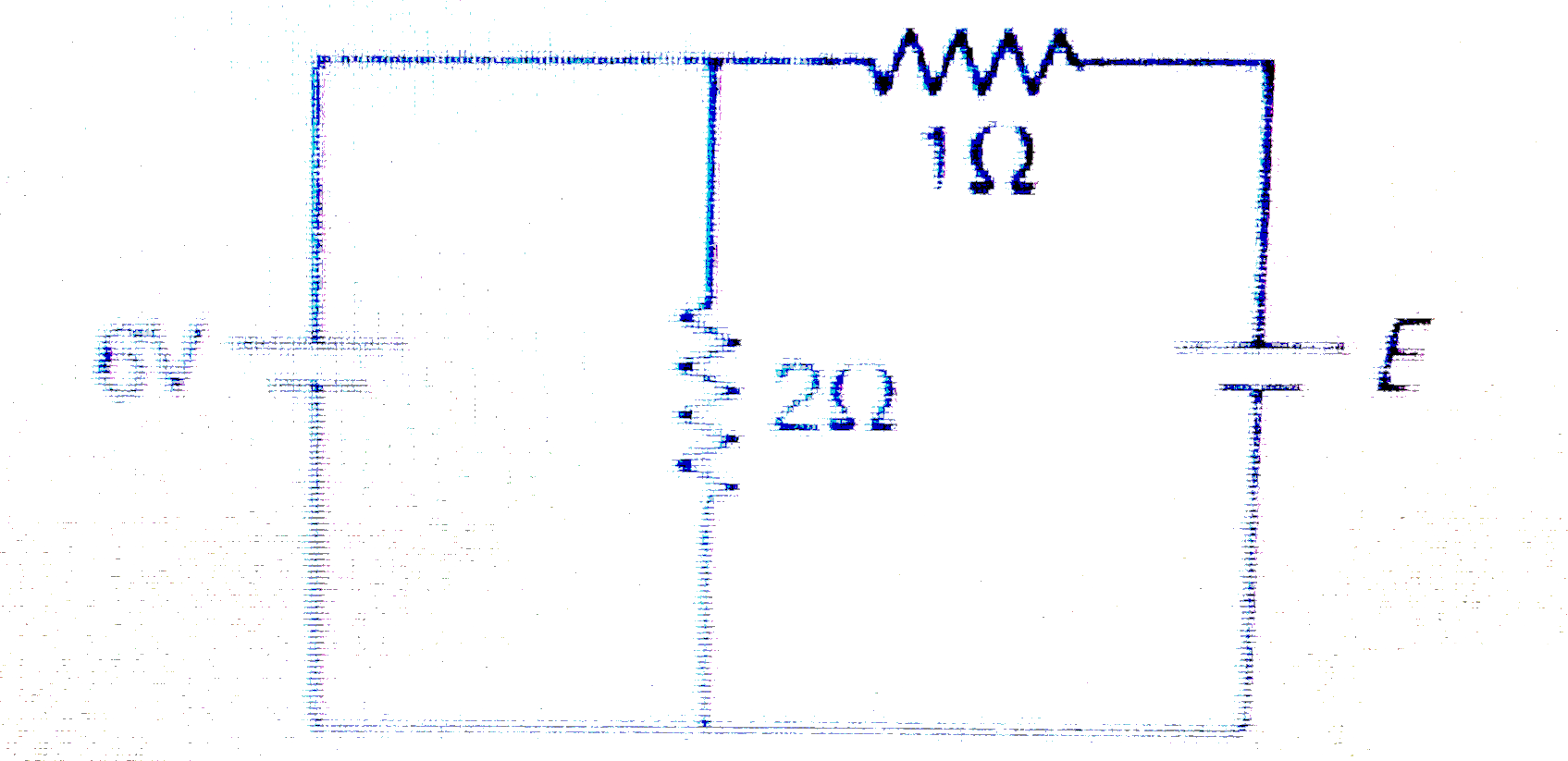 View Solution
View Solution