An object of height 2cm is kept 2m in front of a convex lens of focal length 1m. A plane mirror is placed at 3m from the lens on its other side. Find the nature, position, and magnification of the final image that will be seen by an observer looking toward the mirror through the lens.
To solve the problem step by step, we will follow the process of finding the image formed by the convex lens first, then determine the effect of the plane mirror, and finally calculate the magnification and nature of the final image.
Step 1: Identify the parameters
- Object height (h) = 2 cm
- Object distance from lens (u) = -2 m (negative as per sign convention)
- Focal length of lens (f) = 1 m (positive for convex lens)
- Distance of plane mirror from lens = 3 m
Step 2: Use the lens formula to find the image distance (v)
The lens formula is given by:
1f=1v−1u
Rearranging gives:
1v=1f+1u
Substituting the known values:
1v=11+1−2
1v=1−0.5=0.5
Thus,
v=10.5=2 m
Step 3: Determine the nature and position of the first image
The image formed by the lens is at a distance of 2 m on the opposite side of the lens (since v is positive). This image is real and inverted.
Step 4: Calculate the magnification (M) of the lens
The magnification (M) is given by:
M=h′h=−vu
Substituting the values:
M=−2−2=1
This means the image height (h') = 2 cm (same size as the object but inverted).
Step 5: Consider the plane mirror
The plane mirror is located 3 m from the lens. The image formed by the lens acts as a virtual object for the mirror. The distance from the mirror to the image is:
Distance from mirror to image=3 m−2 m=1 m
Since the image is 2 m from the lens, it is 1 m in front of the mirror.
Step 6: Find the image distance for the mirror
The image formed by the mirror will be at the same distance behind the mirror:
Image distance from mirror=−1 m
This means the virtual image is 1 m behind the mirror.
Step 7: Determine the position of the final image with respect to the lens
The final image distance from the lens will be:
Distance from lens to mirror+Distance from mirror to final image=3 m+1 m=4 m
Step 8: Calculate the final magnification through the lens again
Now we consider this final image as an object for the lens:
- New object distance (u) = -4 m (since it is on the same side as the original object)
Using the lens formula again:
1f=1v−1u
1v=11+1−4
1v=1−0.25=0.75
Thus,
v=10.75=43 m
Step 9: Calculate the final magnification
Using the magnification formula again:
M=−vu=−43−4=13
The final image height will be:
h′=M×h=13×2 cm=23 cm
Conclusion
- Nature of the final image: Virtual and upright.
- Position of the final image: 4/3 m from the lens on the same side as the object.
- Magnification: 1/3, meaning the final image is smaller than the original object.
- Object height (h) = 2 cm
- Object distance from lens (u) = -2 m (negative as per sign convention)
- Focal length of lens (f) = 1 m (positive for convex lens)
- Distance of plane mirror from lens = 3 m
The lens formula is given by:
1f=1v−1u
Rearranging gives:
1v=1f+1u
Substituting the known values:
1v=11+1−2
1v=1−0.5=0.5
Thus,
v=10.5=2 m
The image formed by the lens is at a distance of 2 m on the opposite side of the lens (since v is positive). This image is real and inverted.
The magnification (M) is given by:
M=h′h=−vu
Substituting the values:
M=−2−2=1
This means the image height (h') = 2 cm (same size as the object but inverted).
The plane mirror is located 3 m from the lens. The image formed by the lens acts as a virtual object for the mirror. The distance from the mirror to the image is:
Distance from mirror to image=3 m−2 m=1 m
Since the image is 2 m from the lens, it is 1 m in front of the mirror.
The image formed by the mirror will be at the same distance behind the mirror:
Image distance from mirror=−1 m
This means the virtual image is 1 m behind the mirror.
The final image distance from the lens will be:
Distance from lens to mirror+Distance from mirror to final image=3 m+1 m=4 m
Now we consider this final image as an object for the lens:
- New object distance (u) = -4 m (since it is on the same side as the original object)
Using the lens formula again:
1f=1v−1u
1v=11+1−4
1v=1−0.25=0.75
Thus,
v=10.75=43 m
Using the magnification formula again:
M=−vu=−43−4=13
The final image height will be:
h′=M×h=13×2 cm=23 cm
- Nature of the final image: Virtual and upright.
- Position of the final image: 4/3 m from the lens on the same side as the object.
- Magnification: 1/3, meaning the final image is smaller than the original object.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples15videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.114videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK1videos
FRICTION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank39videos
GRAVITATION
Similar Questions
An object of height 2 cm is kept in front of a concave lens of focal length 20 cm. If the object distance is 30 cm, find the position and the height of the image.
View SolutionAn object is placed at 10 cm from a convex lens of focal length 12 cm. Find the position, nature and magnification of the image.
View Solution
Knowledge Check
- Question 1 - Select One
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Areal, at 30 cm in front of the mirrorBreal, at 30 cm behind the mirrorCreal, at 10 cm in front of the mirrorDvirtual, at 10 cm behind the mirror - Question 2 - Select One
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed 20 cm from the lens. The image is formed
A12 cm in front of the mirrorB60 cm behind the mirrorC60 cm in front of the mirrorD30 cm in front of the mirror
A point object is placed 60cm in front of a convex lens of focal length 15cm. A plane mirror is placed 10cm behind the convex lens. Where is the image formed by the system ?
View SolutionA point like object is placed at a distance of 1 m in front of a convex lens of focal length 0.5 m . A plane mirror is placed at a distance of 2 m behind the lens. The position and nature of the final image formed by the system is :
View SolutionL is a lens such that a parallel beam of light I ncident on it, after refraction, converges to a point at a distance x from it and M is a mirror such that a parallel lbeam of light incident on it, after reflection, converges to a point at a distance y from it. Now the lens L and mirror M are placed at a distan 2(x+y) from each other. An object of size 2 cm is kept infront of lens at a distance 2x from it. Find the nature, position , and size of the image that willl be seen by an observer looking toward the mirror through the lens.
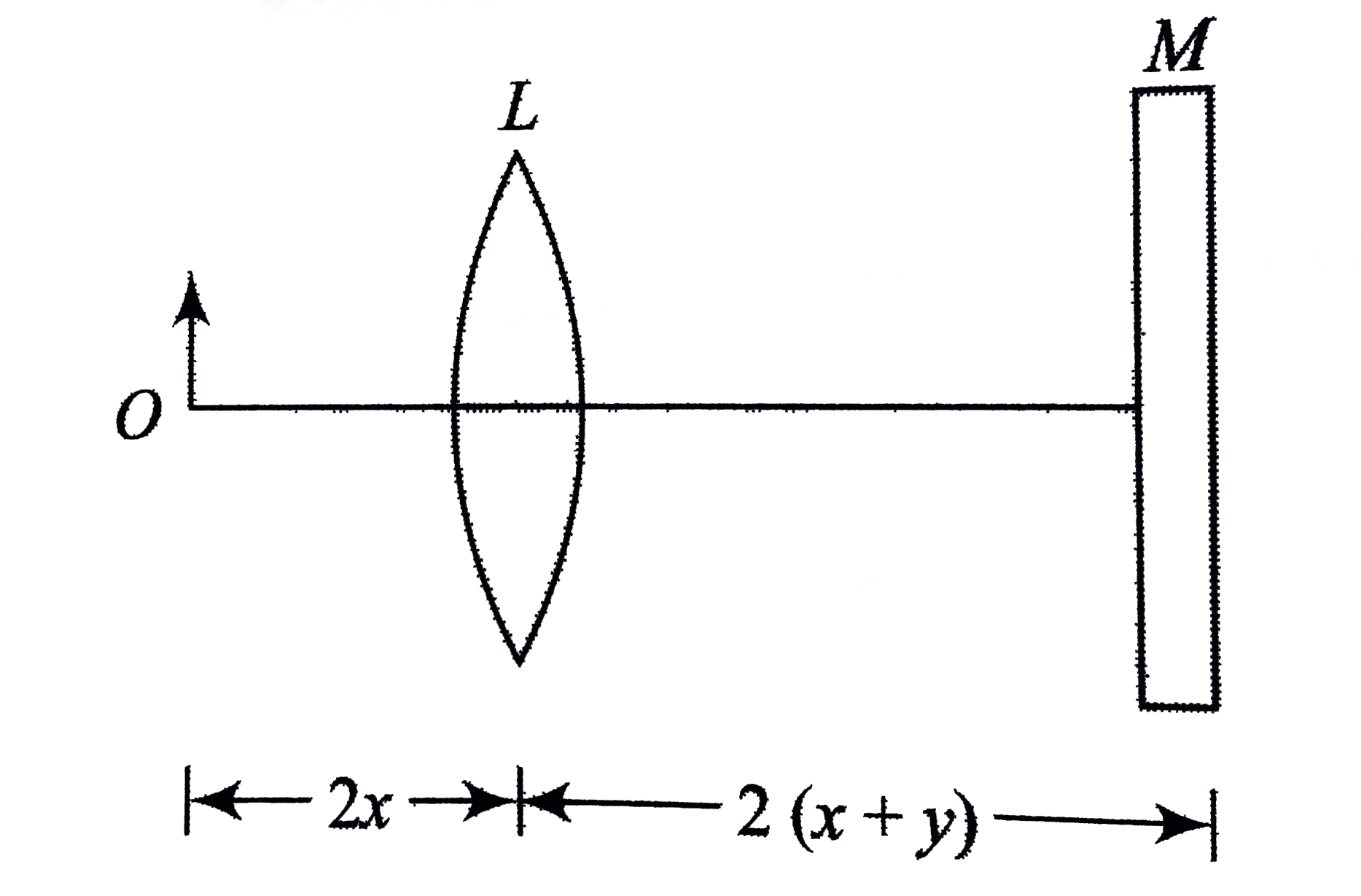 View Solution
View SolutionAn object is kept at 0.2m from a convex lens of focal length 0.15 m. Find the position of the image produced.
View SolutionThe plane surface of a plano-convex lens of focal length 60 cm is silvered. A point object is placed at a distance 20 cm from the lens. Find the position and nature of the final image formed.
View SolutionAn object of height 2 cm is kept at 30 cm from a convex lens . Its real image is formed at 60 cm from the lens. Find the focal length of power of the lens.
View Solution

