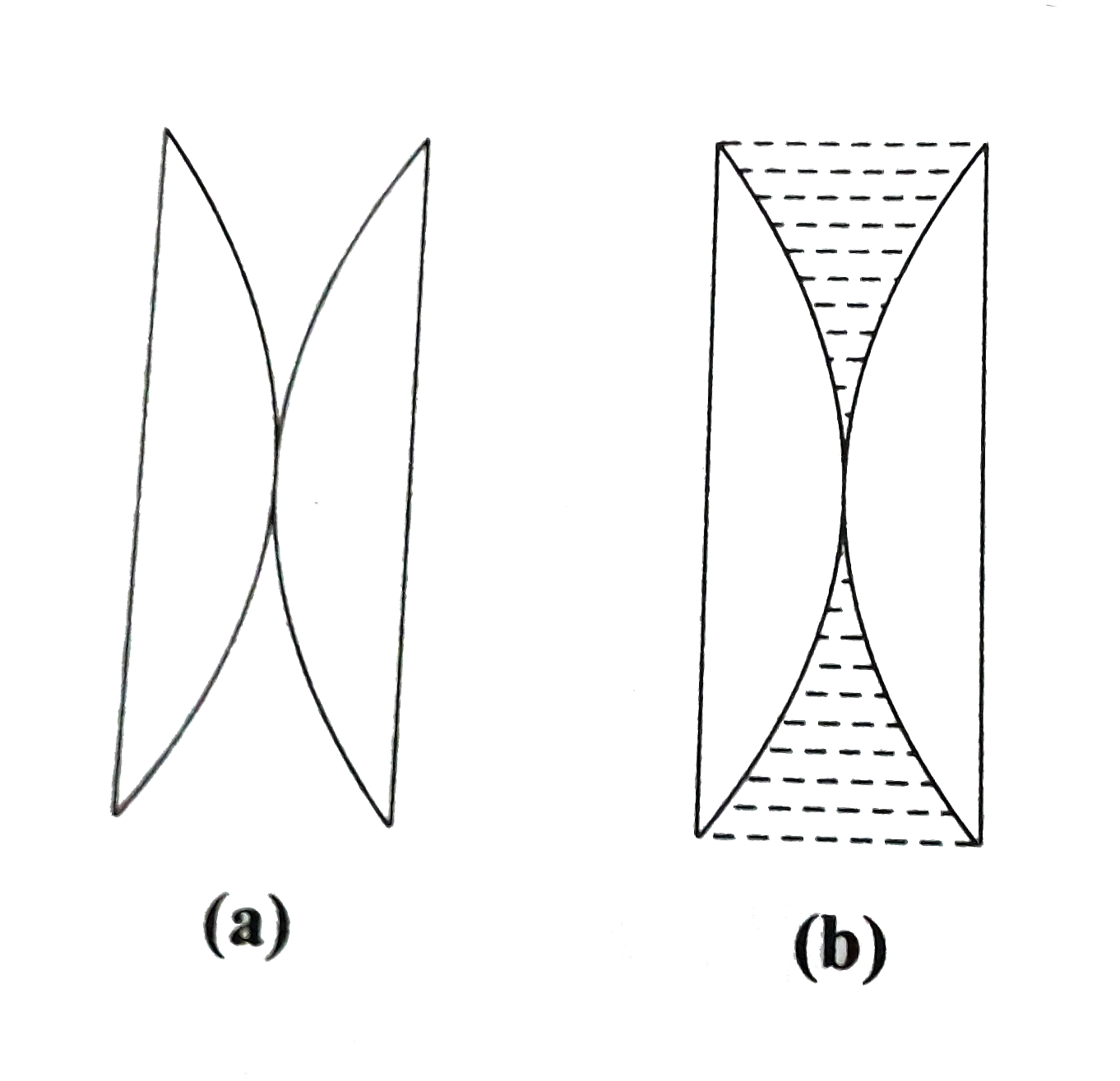
Figure(a) shows two plano-convex lenses in contact as shown. The combination has focal length 24cm. Figure (b) shows the same with a liquid introduced between them. If refractive index of glass of the lenses is 1.50 and that of the liquid is 1.60, the focal length of system in figure (b) will be
$Figure(a) shows two plano-convex lenses in contact as shown. The combination has focal length 24cm. Figure (b) shows the same with a liquid introduced between them. If refractive index of glass of the lenses is 1.50 and that of the liquid is 1.60, the focal length of system in figure (b) will be$

A

$- 120 c m$

B

120cm

C

$- 24 c m$

D

$24 c m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:a.

Since the refractive index of the liquid is grater than the refractive index of glass, therefore the focal length of the system has to be negative. So, options (b) and (d) are excluded.
Now, $\frac{1}{24} = (1.5 - 1) \frac{2}{R}$
or $R = 24 c m$
Again, for liquid concave lens,
$\frac{1}{f} = - (1.6 - 1) (\frac{2}{24})$
$\frac{1}{f} = - \frac{3}{5} \times \frac{1}{12}$
or $\frac{1}{f} = - \frac{1}{12}$ or $f = - 20 c m$
Now, $\frac{1}{F} = \frac{1}{24} - \frac{1}{20} = \frac{5 - 6}{120}$
or $F = - 120 c m$

|

Updated on:21/07/2023

Class 12 PHYSICS RAY OPTICS AND OPTICAL INSTRUMENTS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two thin lenses of focal lengths $f_{1} and f_{2}$ are placed in contact. The focal length of the composite lens will be
A $\frac{f_{1} + f_{2}}{2}$
B $\frac{f_{1} + f_{2}}{f_{1} f_{2}}$
C $\sqrt{f_{1} f_{1}}$
D $\frac{f_{1} f_{2}}{f_{1} + f_{2}}$
Question 2 - Select One
Shown in the figure here is a convergent lens placed inside a cell filled with a liquid. The lens has focal length $+ 20 c m$ when in air and its material has refractive index 1.50. If the liquid has refractive index 1.60, the focal length of the system is

A $+ 80 c m$
B $- 80 c m$
C $- 24 c m$
D $- 100 c m$
Question 3 - Select One
A plano-convex lens has refractive index 1.6 and radius of curvature 60 cm. What is the focal length of the lens?
A50 cm
B100 cm
C200 cm
D150 cm

CENGAGE PHYSICS-GEOMETRICAL OPTICS-Single Correct

Home
Ask Doubt
Profile