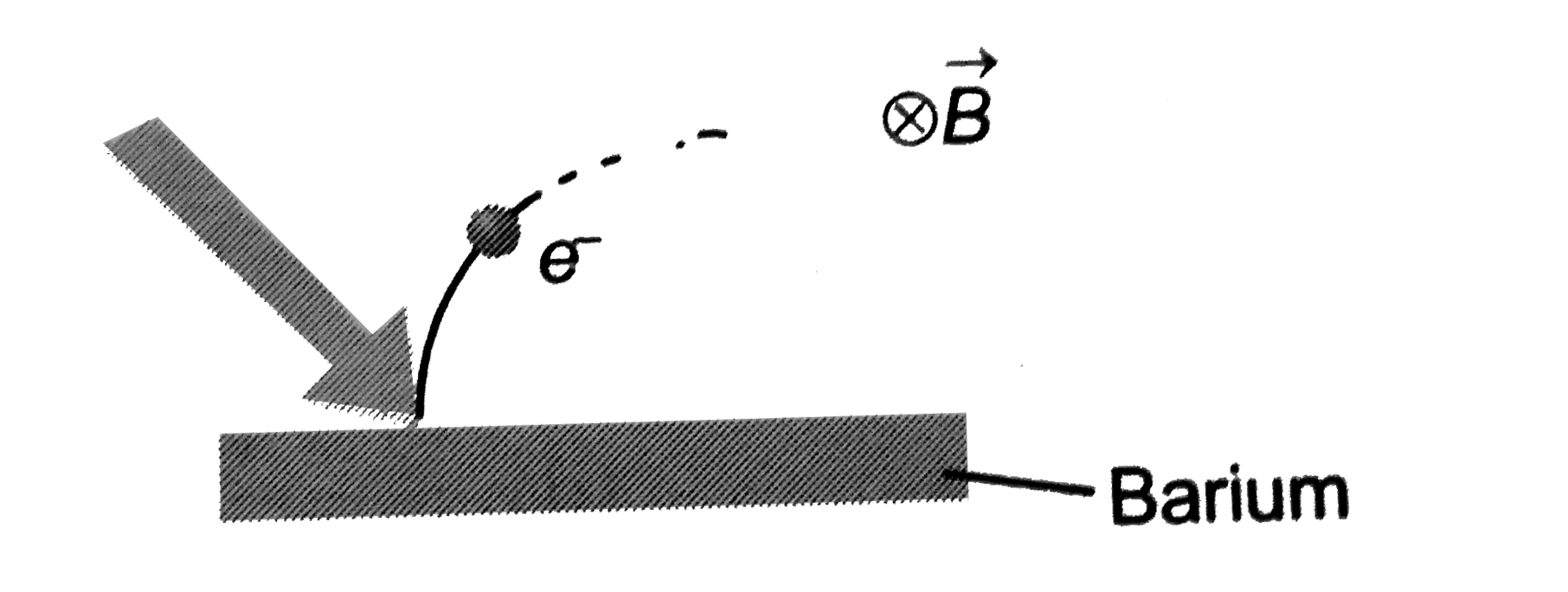
When barium is irradiated by a light of λ=4000A all the photoelectrons emitted are bent in a circle of radius 50 cm by a magnetic field of flux density 5.26×10−6 T acting perpendicular to plane of emission of photoelectron. Then,
the kinetic energy of fastest photoelectrin is 0.6 eV
work function of the metal is 2.5 eV
the maximum velocity of photoelectron is 0.46×106ms−1
the stopping potential for photoelectric effect is 0.6 V
The correct Answer is:A, B, C, D
To solve the problem step by step, we will follow the outlined procedure in the video transcript.
Step 1: Calculate the Maximum Velocity of the Photoelectron
The formula to find the velocity v of the photoelectron in a magnetic field is given by:
v=qBrm
Where:
- q = charge of the electron = 1.6×10−19C
- B = magnetic flux density = 5.26×10−6T
- r = radius of the circular path = 0.50m
- m = mass of the electron = 9.1×10−31kg
Substituting the values into the formula:
v=(1.6×10−19C)(5.26×10−6T)(0.50m)9.1×10−31kg
Calculating the numerator:
(1.6×10−19)(5.26×10−6)(0.50)=4.208×10−25
Now calculating the velocity:
v=4.208×10−259.1×10−31≈0.462×106m/s≈0.46×106m/s
Step 2: Calculate the Kinetic Energy of the Photoelectron
The kinetic energy K of the photoelectron can be calculated using the formula:
K=12mv2
Substituting the values:
K=12(9.1×10−31kg)(0.46×106m/s)2
Calculating v2:
(0.46×106)2=0.2116×1012m2/s2
Now substituting back into the kinetic energy formula:
K=12(9.1×10−31)(0.2116×1012)≈9.63×10−19J
To convert this to electron volts (1 eV = 1.6×10−19J):
K≈9.63×10−191.6×10−19≈0.602eV≈0.6eV
Step 3: Calculate the Stopping Potential
The stopping potential V0 can be calculated using the relationship:
eV0=K
Where e is the charge of the electron. Therefore,
V0=Ke=0.6V
Step 4: Calculate the Work Function
The work function ϕ can be calculated using the equation:
ϕ=E−K
Where E is the energy of the incident photons. The energy of the photons can be calculated using:
E=hcλ
Where:
- h=6.63×10−34J s (Planck's constant)
- c=3×108m/s (speed of light)
- λ=4000Å=4000×10−10m
Calculating E:
E=(6.63×10−34)(3×108)4000×10−10≈4.97×10−19J
Converting this to electron volts:
E≈4.97×10−191.6×10−19≈3.106eV
Now calculating the work function:
ϕ=E−K≈3.106eV−0.6eV≈2.506eV≈2.5eV
Summary of Results
1. Maximum Velocity of Photoelectron: 0.46×106m/s
2. Kinetic Energy: 0.6eV
3. Stopping Potential: 0.6V
4. Work Function: 2.5eV
- q = charge of the electron = 1.6×10−19C
- B = magnetic flux density = 5.26×10−6T
- r = radius of the circular path = 0.50m
- m = mass of the electron = 9.1×10−31kg
- h=6.63×10−34J s (Planck's constant)
- c=3×108m/s (speed of light)
- λ=4000Å=4000×10−10m
1. Maximum Velocity of Photoelectron: 0.46×106m/s
2. Kinetic Energy: 0.6eV
3. Stopping Potential: 0.6V
4. Work Function: 2.5eV
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Linked Comprehension44videos
PHOTOELECTRIC EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Integer9videos
PHOTOELECTRIC EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Single Correct130videos
PHOTOELECTRIC EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - NUCLEIEXERCISE - QUESTION BANK59videos
NUCLEI
- BOOK - CENGAGE PHYSICSCHAPTER - RAY OPTICSEXERCISE - DPP 1.612videos
RAY OPTICS
Similar Questions
When 0.50 Å X-ray strike a material , the photoelectron from the K shell are observed to move in a circle of radius 2.3nm in a magnetic field of 2×10−2 tesle acting perpendicular to direct of emission of photoelectron . What is the binding elergy of k−shell electron?
View SolutionLight of wavelength 4000Å is incident on barium. Photoelectrons emitted describe a circle of radius 50 cm by a magnetic field of flux density 5.26×10−6tesla. What is the work of barium in eV? Given h=6.6×10−34Js,e=1.6×10−19C,me=9.1×10−31kg.
View Solution
Knowledge Check
- Question 1 - Select One
When 0.50 Å X-ray strike a material , the photoelectron from the K shell are observed to move in a circle of radius 2.3nm in a magnetic field of 2×10−2 tesle acting perpendicular to direct of emission of photoelectron . What is the binding elergy of k−shell electron?
A3.5 keVB6.2 keVC2.9 keVD5.5 keV - Question 2 - Select One
A cathode ray beam is bent in a circle of radius 2 cm by a magnetic induction 4.5×10−3weber/m2 ,The velocity of electron is
A3.43×107m/sB5.37×107m/sC1.23×107m/sD1.58×107m/s - Question 3 - Select One
When 24.8keV X- rays strike a material, the photoelectrons emitted from K shell are observed to move in a circle of radius 23 mm in a magnetic field of 2×10−2T. The binding energy of K shell electrons is
A6.2 keVB5.4 keVC7.4 keVD8.6 keV
The light of wavelength 4,125Å is incident on a metal surface of work function ϕ The emitted photoelectrons describe a circle of radius 200 cm under the effect of a magnetic field of flux density 1√7×10−5 tesla. If charge to mass ratio for electron is 1.7×1011 , find the value of ϕ.
0123456789View SolutionA current - carrying circular coil of 100 turns and radius 5.0 cm produces a magnetic field of (6.0×10−5) T at its centre. Find the value of the current.
View SolutionCalculate torque acting on a bar magnet of length 20 cm magnetic dipole moment 4×10−6Am2 , which is placed in earth's magnetic field of flux density 2×10−5T , perpendicular to the field .
View SolutionLight of wavelength 2475Å is incident on barium. Photoelectrons emitted describe a circle of radius 100cm by a magnetic field of flux density 1√17×10−5Tesla.
Work function of the barium is (Given em=1.7×1011)
 View Solution
View SolutionRadiation of wavelength 120 nm ejects photoelectrons from a plate whose work function is 3.0 eV. If a magnetic field of flux density 4.0×10−5 T is applied parallel to the plate, then the radius of the path followed by electrons ejected normally from the plate with maximum energy will be
View Solution

