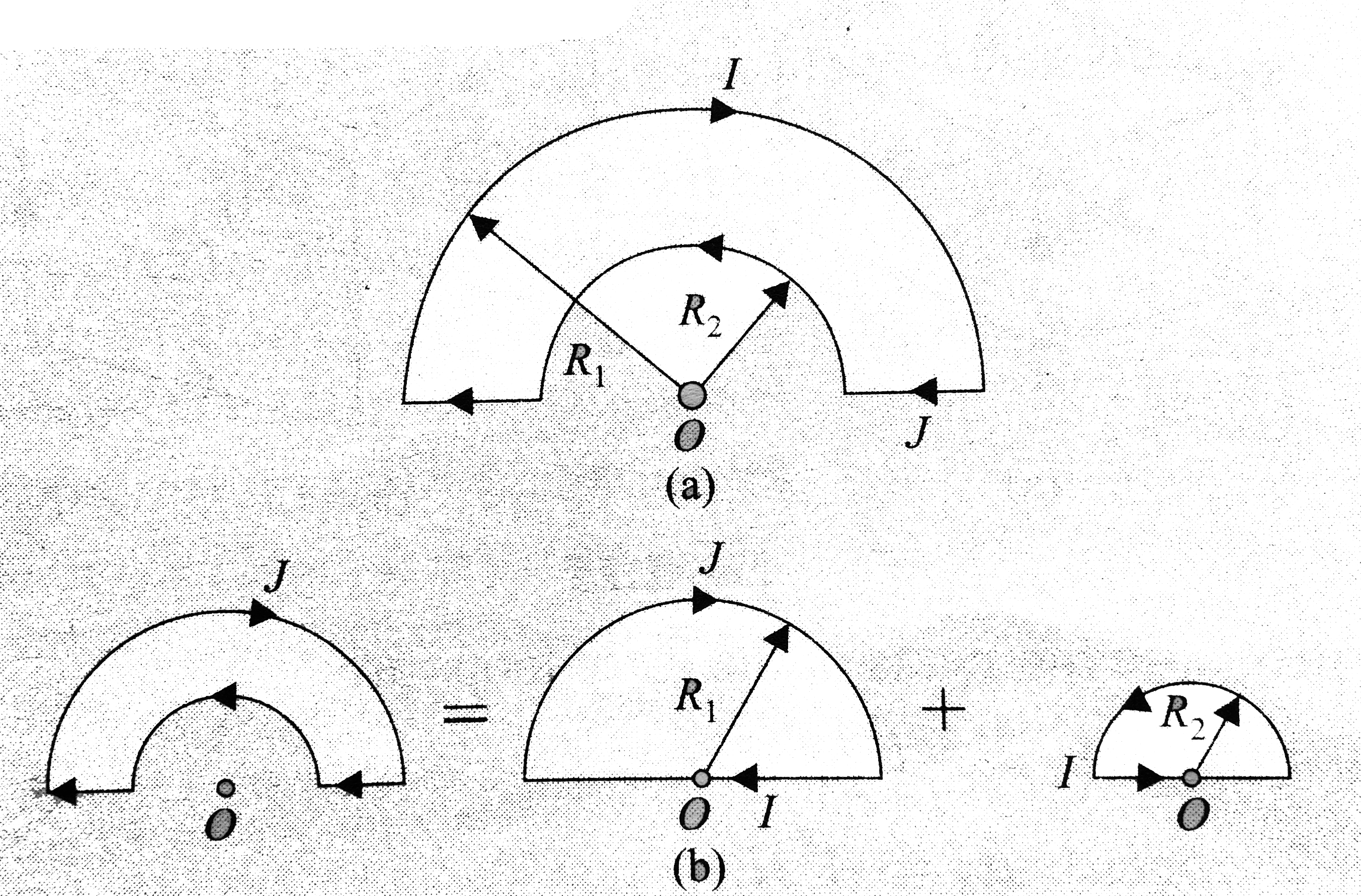
Compute the magnetic dipole moment of the loop shown in Fig. 1.69
Video Solution
Text Solution
The given loop may be considered as the superposition of
the two loops, as shown in the figure.
The resultant dipole moment is M=πR21I2−πR22I2
or M=πI2(R21−R22) (inward)
|
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Solved Example
25 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.1
22 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
Knowledge Check
Magnetic dipole moment is a
AScalar quantityBVector quantityCConstant quantityDNone of theseThe magnetic dipole moment has the dimension of
Acurrent x lengthBcharge x time x lengthCcurrent x areaDcurrentareaMagnetic dipole moment of rectangular loop is
Ainversely proportional to current in loopBinversely proportional to area of loopCparallel to plane of loop and proportional to area of loopDperpendicular to plane of loop and proportional to area of loop
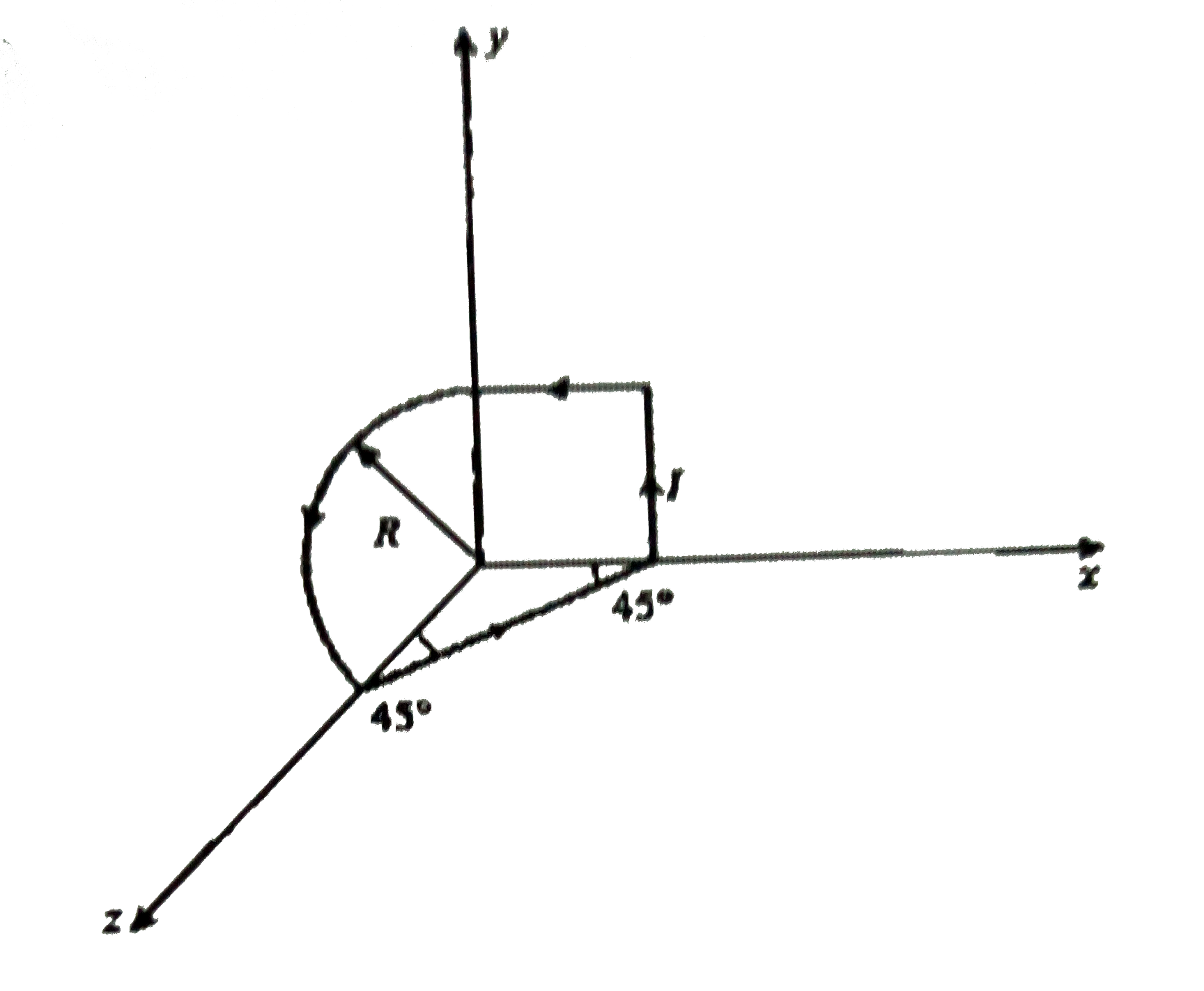
Find the magnetic moment of the current carrying loop shown in figure.
View SolutionThe magnetic dipole moment of current loop is independent of
View SolutionThe magnetic dipole moment of a current loop is independent of
View SolutionMagnetic dipole moment of revolving electron is
View SolutionThe magnetic moment of the loop shown in the adjacent figure is :( length of each side is a )

View Solution
