A stsnding wave y=2Asinkxcosωt is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B. Now answer the following question:
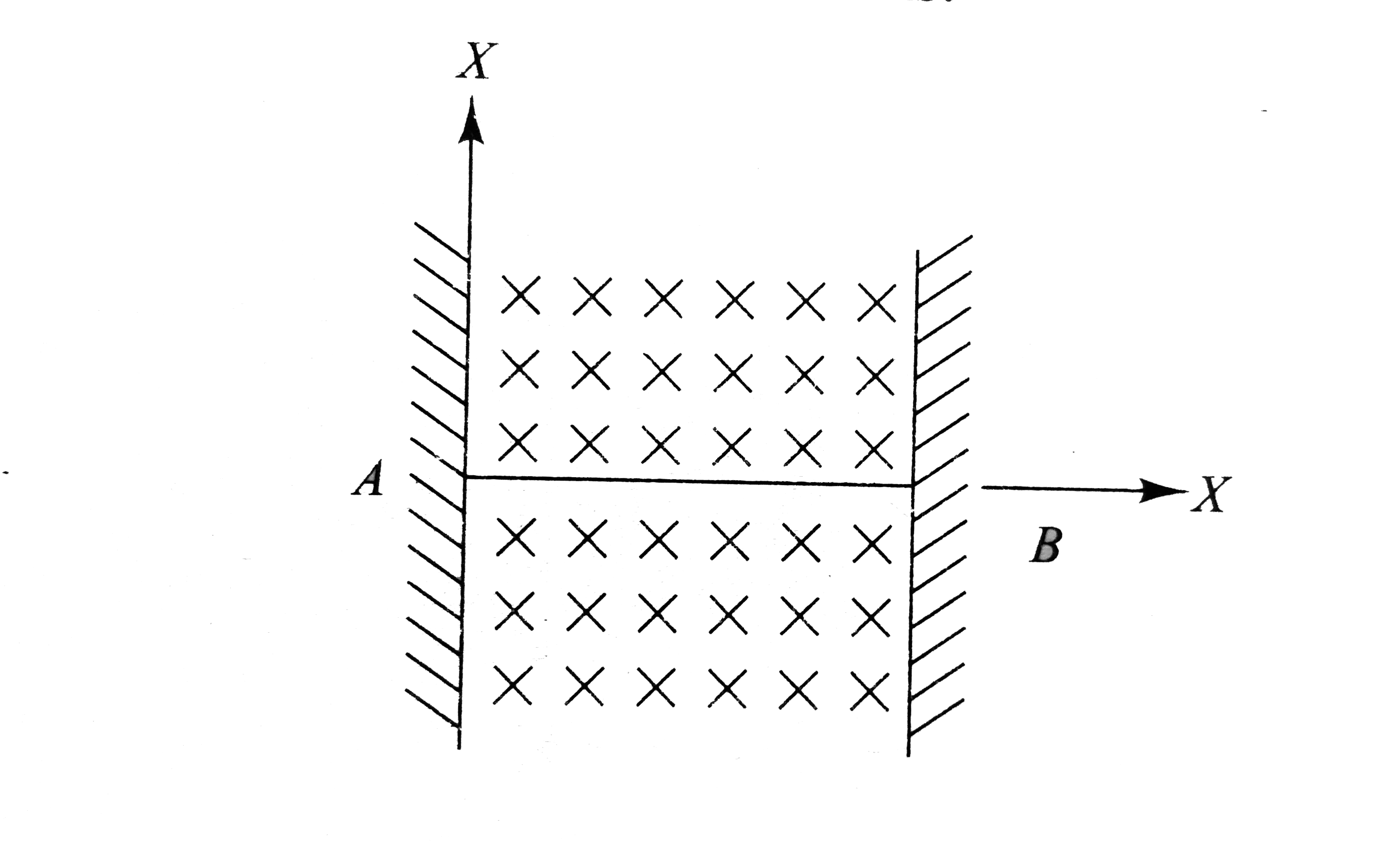
In which of the following modes the emf induced in AB is always zero?
(a) fundamental modes
(b) second harmonic
( c) ssecond overtone
(d) fourth overtone
The correct Answer is:B
(b) y=2Asinkxcosωt
v=dydt=−2Asinkxωsinωt
vmax=−2Aωsinkx,k=3πAB
e=∫l=AB0bvmaxdx=−2aωB∫AB0sinkxdx=+2ωABk[cos(3π)ABAB−cosθ]=−4(AB)ωk
ωt=π2
t=π2ω
For second harmonic k=2πAB
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Integer9videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Archives Fill In The Blanks3videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Asserton - Reasoning8videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGENTIC INDUCTIONEXERCISE - QUESTION BANK40videos
ELECTROMAGENTIC INDUCTION
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.315videos
ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYS
Similar Questions
In Fig. ABCD is a fixed smooth conducting frame in horizontal plane. T is bulb of power 100W,P is a smooth pulley and OQ is a conducting rod. Neglect the self-inductance of the loop and resistance of any part other than the bulb. The mass M is moving down with constant velocity 10ms−1. Bulb lights at its rated power due to induced emf in the loop due to earth's magnetic field. Find the mass M (in kg) of teh block. (g = 10 ms^(2))
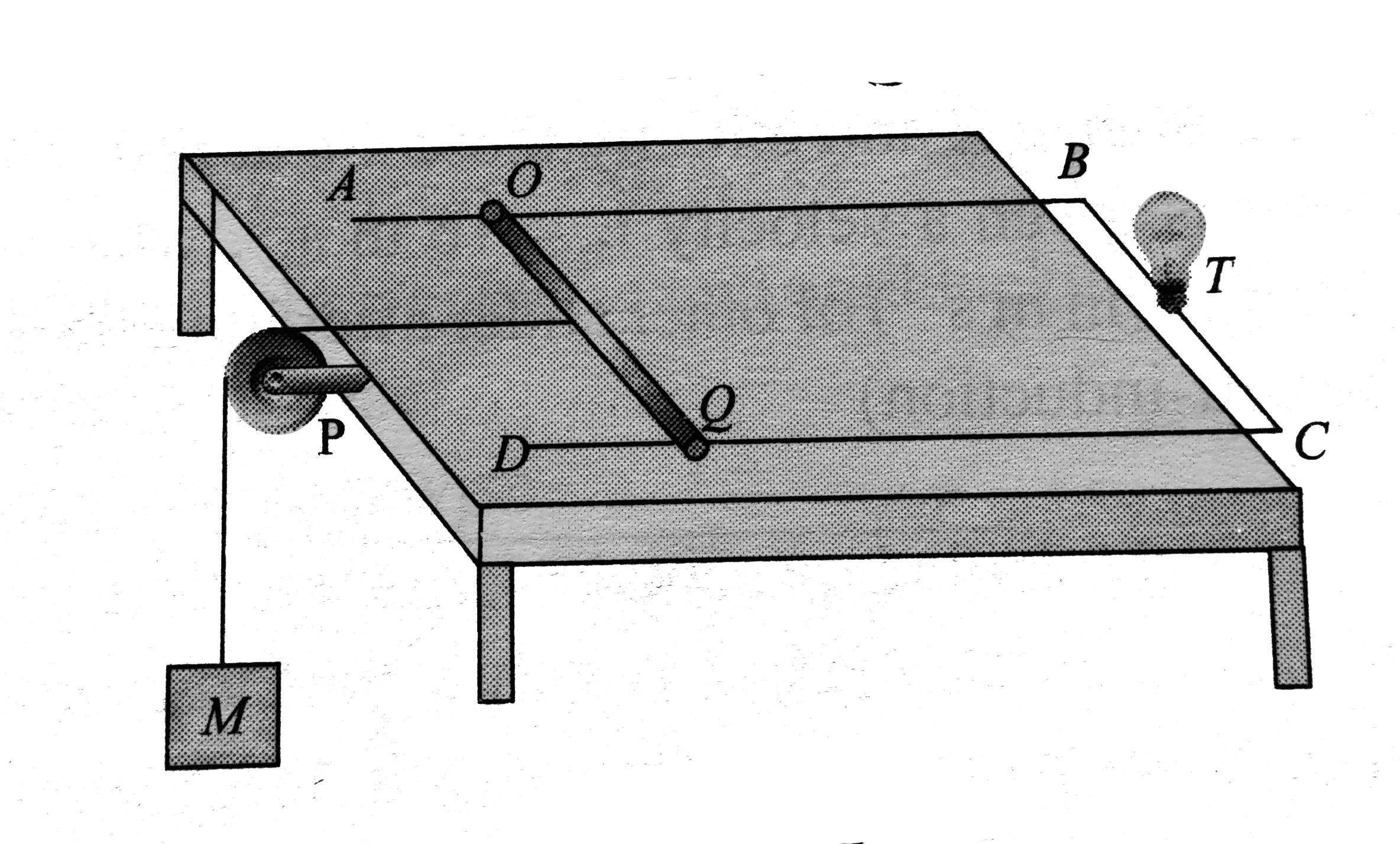 03:49View Solution
03:49View SolutionIn a straight conducting wire, a constant current is flowing from left to right due to a source of emf. When the source is switched off, the direction of the induced current in the wire will.........
02:15View SolutionStatement I: An emf is induced ina long solenoid by a bar magnet that moves while totally inside the solenoid along axis of the solenoid.
Statement II: as the magnet moves inside the solenoid the flux through turns of the solenoid changes.04:25View SolutionA flat, circular coil of radius 10cm has 100 turns of wire. Auniform magnetic field exists in a direction perpendicular to the plane of the coil. This ficld is increasing at the rate of 0.1Ts. Calculate the magnitude of emf induced (in volt) in the coil.
05:16View SolutionThe figure shows different graphs between stopping potential (V0) and frequency (v) for photosensitive surface of cesium , potassium , sodium and lithium. The plots are parallel. Correct ranking of the targets according to their work function greatest forst will be
 03:54View Solution
03:54View SolutionA wire is stretched and fixed at two ends. Transverse stationary waves are formed in it. It oscillates in its third overtone mode. The equation of stationary wave is y= A sin kx cos omegat Choose the correct options.
01:12View SolutionWhich of the following equations can form stationary waves? (i) y= A sin (omegat - kx) (ii) y= A cos (omegat - kx) (iii) y= A sin (omegat + kx) (iv) y= A cos (omegat - kx) .
02:33View SolutionA standing wave y = 2A sin kx cos omegat is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B . Now answer the following question: The wire is found to vibrate in the third harmonic. The maximum emf induced is
03:08View SolutionA stsnding wave y = 2A sin kx cos omegat is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B . Now answer the following question: In the above qestion, the time when the emf becomes maximum for the time is
02:14View SolutionA standing wave y=2A sinkx cos omegat is setup in the wire AB fixed at both ends by two vertical walls (see the figure). the region between the wall contains a constant magnetic field B . Now answer the following questions. In the above question, the time when the emf becomes maximum for the first time is
02:38View SolutionTwo SHM's are represented by y = a sin (omegat - kx) and y = b cos (omegat - kx) . The phase difference between the two is :
02:56View SolutionThe equation of a standing wave in a string fixed at both its ends is given as y=2A sin kx cos ωt. The amplitude and frequency of a particle vibrating at the point of string midway between a node and an antinode is
View Solutionदो तरंगो y=acos(ωt+kx) तथा y=asin(ωt+kx) में कलांतर बताइये।
08:26View SolutionTwo sound waves are y = a sin (omegat - kx), y = a cos (omega - kx) . Phase difference between two waves is
03:27View SolutionA wire is stretched and fixed at two ends. Transverse stationary waves are formed in it. It oscillates in its third overtone mode. The equation of stationary wave is
y=Asinkxcosωt
Choose the correct options.02:52View SolutionWhich of the following equations can form stationary waves? (i) y= A sin (omegat - kx) (ii) y= A cos (omegat - kx) (iii) y= A sin (omegat + kx) (iv) y= A cos (omegat - kx) .
02:48View Solution

