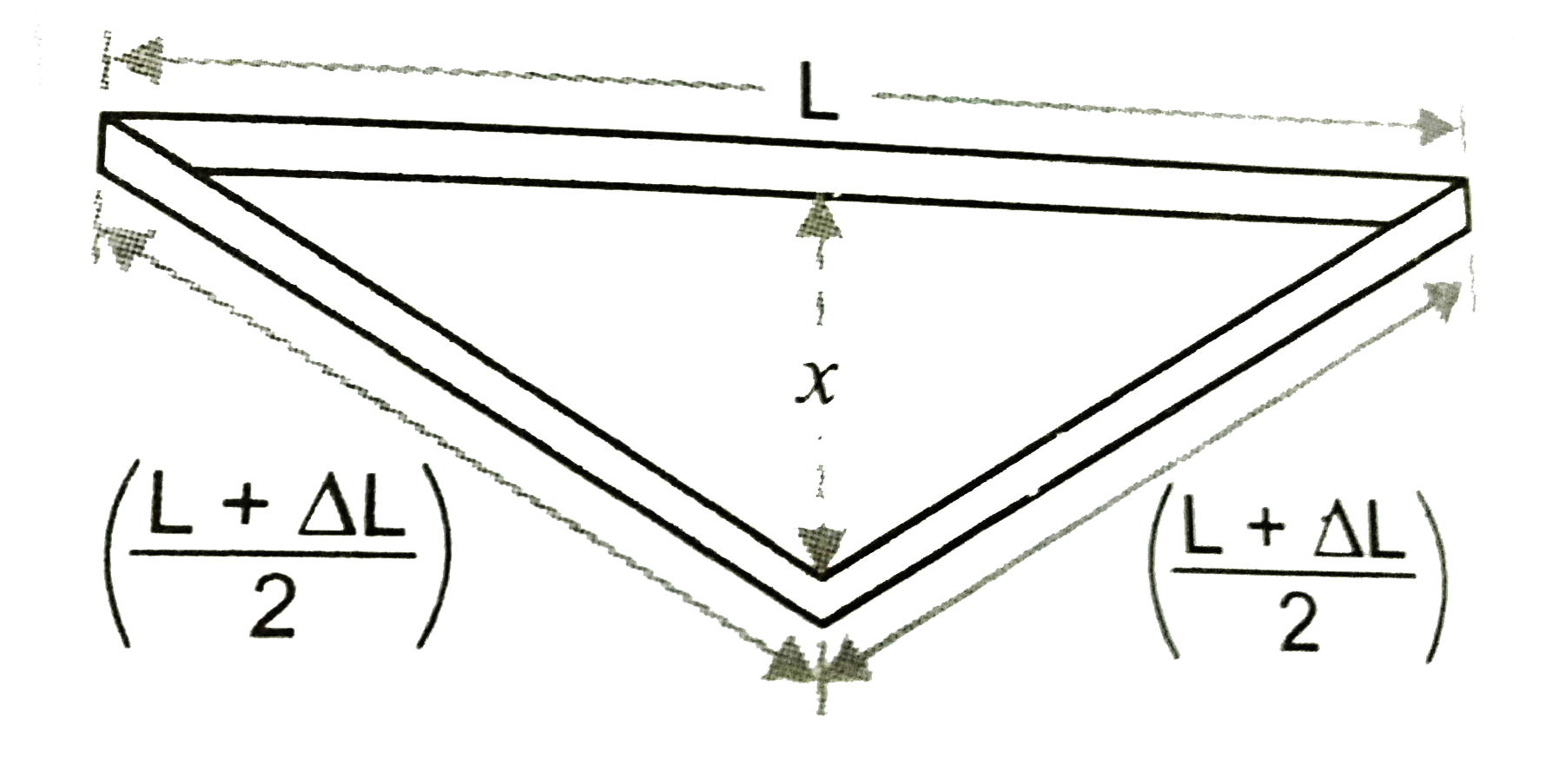
A steel rail 30 m long is firmly attached to the roadbed only at its ends. The sun raises the temperature of the rail by $5^{\circ} C$ , causing the rail to buckle. Assuming that the buckled rail consists of two straight parts meeting in the centre, calculate how much the centre of the rail rises. coefficient of linear expansion of steel is $12 \times 10^{- 6} / K$ .

Video Solution

Text Solution

Verified by Experts

As indicated in Fig. we let the initial length be 2 s and the final total length be $2 (s + Δ s)$
The height of the centre of the buckled rail is denoted by y. Assuming that the standard coefficient $α$ of linear expansion can be used (in spite of the fact that the ends are anchored), we have $Δ s = α s Δ T$ .
By the Pythagorean theorem
$y = \sqrt{{(s + Δ s)}^{2} - s^{2}} = \sqrt{2 s Δ s + {(Δ s)}^{2}}$
$\sqrt{2 α Δ T + {(α Δ T)}^{2}}$
With $s = 15.0 m$ , $α 12 \times \frac{10_{6}}{K}$ , and $Δ T = 50 K$ , we obtain
$y = (15.0 m) \sqrt{(12 \times 10^{- 4}) + {(6 \times 10^{- 4})}^{2}} = 0.52 m$

Updated on:21/07/2023