A cube and a sphere of equal edge and radius, made of the same substance are allowed to cool under identical conditions. Determine which of the two will cool at a faster rate.
From equation, the rate of cooling of a body is given by
−dTdt=AeσρVs(T4−T40)
since, substance is same for both bodies, so
e/ρs=constant.
Finally, thay are allowed to cool under identical conditions so (T4−T40)= constant.
−dTdt∝AV
Let the edge of the cube or radius of the sphere be a, then for cube, A=6a2 and V=a3 so AV=6a
For the sphere, A=4πa2 and V=(4/3)πa3, so A/V=3/a
Evidently, the ratio A/V is more for cube, so the cube cools at a faster rate. Not the special technique used in this problem.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Subjective25videos
CALORIMETRY
- BOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Single Correct148videos
CALORIMETRY
- BOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Exercise 1.222videos
CALORIMETRY
- BOOK - CENGAGE PHYSICSCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.620videos
BASIC MATHEMATICS
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE1videos
CENTRE OF MASS
Similar Questions
A cube, sphere and a cylinder made of same material shown in figure are allowed to cool under identical conditions. Determine which of these will cool at a faster rate?
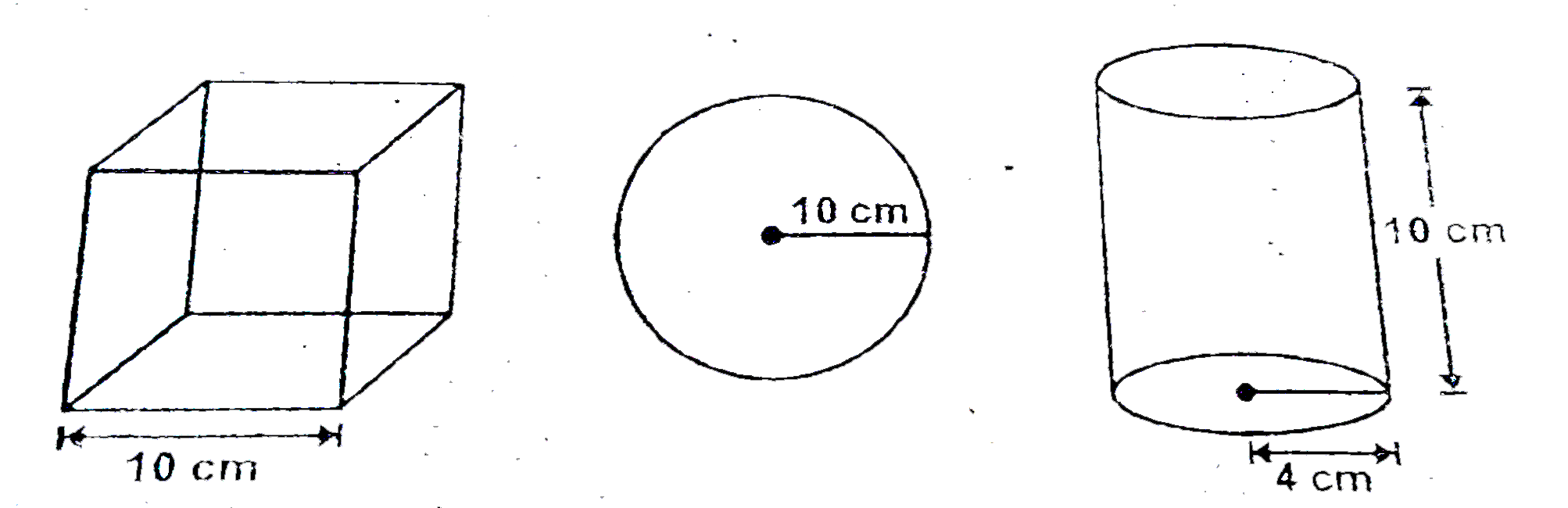 .View Solution
.View SolutionTwo solid spheres of radii 5cm and 3cm are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. The ratio of rate of fall of temperature is
View Solution
Knowledge Check
- Question 1 - Select One
Two solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
AR1R2BR2R1CR21R22DR22R21 - Question 2 - Select One
Two solid spheres of radii R1 and R2 are made of same material and have similar surface. The spheres are raised to the same temperature and then allowed to cool under identical conditions. Assuming spheres to be perfect conductors of heat, their initial ratio of rates of loss of heat is:
AR21R22BR1R2CR2R1DR22R21 - Question 3 - Select One or More
Which of the following gases will have the same rate of effusion under identical conditions ?
AC2H4BC3H8CCO2DN2O
Two solid spheres, one of aluminium and the other of copper, of twice the radius are heated to the same temperature and are allowed to cool under the identical conditions. Given that specific heat of aluminium is 900J/kg K and that ofcopper is 390J/kg K. Specific gravity of aluminium and copper are 2.7 and 8.9 respectively.
initial rates of fall of temperature, and
the initial rates of loss of heatView SolutionA solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperature. Assume that the emissivity of both the spheres is the same. Find the ratio of
(a) the rate of heat from the aluminium sphere to the rate of heat loss from the copper sphere and
(b) the rate of fall of temperature of the the aluminium sphere to the rate of fall temperature of teh copper sphere. The specific heat capacity of aluminium =900J/kg−∘C and that of copper =390J/kg−∘C. The density of copper is 3.4 times the density of aluminium.View SolutionA solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperatures. Assume that the emissivity of both the spheres is the same. Find ratio of (a) the rate of heat loss from the aluminium sphere to the rate of all of temperature of the copper sphere. The specific heat capacity of aluminium =900Jkg−1^(@)C^(-1).andtˆofcopper=390Jkg^(-1)^(∘)C−1 . The density of copper =3.4 times the correct wattage.
View SolutionA solid copper cube and sphere, both of same mass & emissivity are heated to same initial temperature and kept under identical conditions. What is the ratio of their initial rate of fall of temperature ?
View SolutionWhen a sphere, a cube and a thin plate are heated to same temperature and then allowed to cool, the first to cool is
View Solution

