Consider a cylindrical container of cross-section area A length h and having coefficient of linear expansion $α_{c}$ . The container is filled by liquid of real expansion coefficient $γ_{L}$ up to height $h_{1}$ . When temperature of the system is increased by $Δ θ$ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between $γ_{L}$ and $α_{c}$ for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by $Δ θ$ , then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.

Video Solution

Text Solution

Verified by Experts

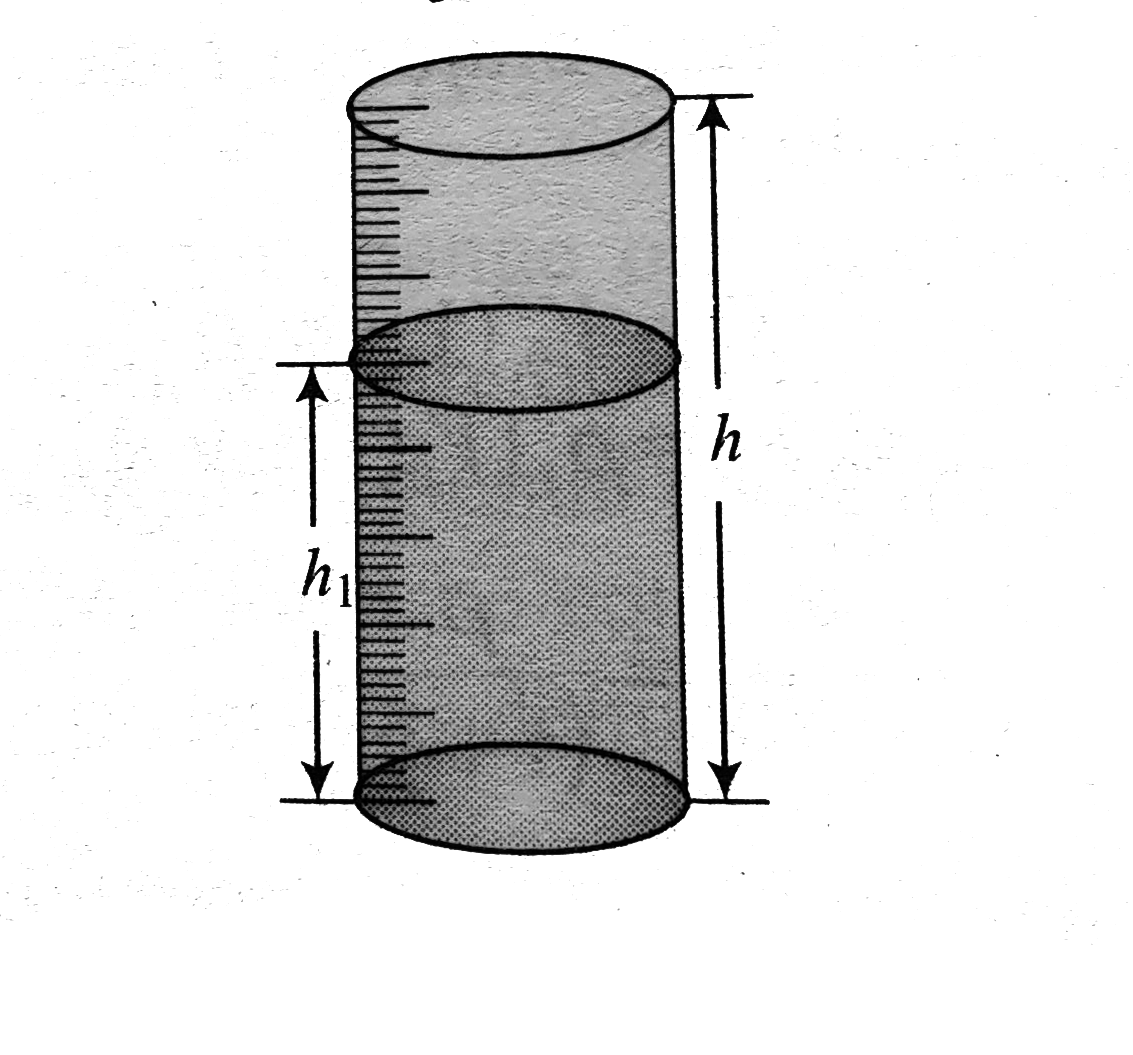
On increasing the temperature, the height area of cross section and volume of the cylinder will increase.
(a). New height $= h_{f} = h {1 + α Δ θ}$
New area of cross section $A_{f} = A {1 + 2 α_{c} Δ θ}$
New volume of container $V_{f} = A h {1 + 3 α (c) Δ θ}$
New volume of liquid $V_{ω} = V_{0} (1 + γ_{L} Δ θ)$
$V_{ω} = A h_{1} (1 + γ_{L} Δ θ)$
(b). The height of liquid level when expansion of container is neglected
$h_{f} = \frac{V_{ω}}{A} = \frac{A h_{1} (1 + Y_{L} Δ θ)}{A}$
$\Rightarrow h_{f} = h_{1} {1 + γ_{L} Δ θ}$
(c). The initial volume of container above the liquid.
$Δ V_{1} = A h - A h_{1}$
final volume of container above the liquid
$Δ V_{2} = A h (1 + 3 α_{c} Δ θ) - A h_{1} (1 + γ_{L} Δ θ)$
If volume of above container increases $Δ V_{2} > Δ V_{1}$
$[A h (1 + 3 α_{c} Δ θ) - A h_{1} (1 + γ_{L} Δ θ)] > [A h - A h_{1}]$
Which gives $3 h α_{c} > h_{1} γ_{L}$
Similarly, we can prove if the volume above container decreases, then $3 h α_{c} < h_{1} γ_{L}$ and for no change in volume 3 h $α_{c} = h_{1} γ_{L}$
(d). The area of container will increase, the area of container at this temperature will be
$A_{f} = A {1 + 2 α_{c} Δ θ}$
As liquid does not expand the volume of the liquid wll be as initial volume $= A h_{1}$
Hence height of the liquid column will be
$h_{1}$ )= $\frac{A h_{1}}{A_{f}} = \frac{A h_{1}}{A (1 + 2 α_{c} Δ θ)} \Rightarrow h_{1}$ )= $H_{1} (1 - 2 α_{c} Δ θ)$
. In this case we are neglecting the expansion of container. The volume of liquid at this temperature $< b r >$ $V_{ω}$ )) $=$ Ah_(1)(1+gamma_(L)Deltatheta)
$H e n c e h e i g h t o f t h e l i q u c o n t a \in e r i s < b r >$ h_(f) $=$ (V_(omega))/(A) $=$ (Ah_(1)(1+gamma_(L)Deltatheta))/(A) $\Rightarrow$ h_(f) $=$ h_(1)(1+gamma_(L)Deltatheta))/(A) $\Rightarrow$ h_(f) $=$ h_(1)(1+gamma_(L)Deltatheta)

Updated on:21/07/2023

Coefficient of linear expansion $α$ an coefficient of volume expansio $γ$ of solid are related as
A $γ = 3 α$
B $α = 3 γ$
C $γ = 2 α$
D $α = γ$
A long cylindrical metal vessel of volume $V$ and coefficient of linear expansion $α$ contains a liquid. The level of liquid has not changed on heating. The coefficient of real expansion of the liquid is.
A $\frac{V - α}{V}$
B $\frac{V + α}{V}$
C $\frac{V}{V - α}$
D $3 α$
On heating a liquid having coefficient of volume expension $α$ in a container having coefficient of linear expansion $α / 2,$ the level of the liquid in the container would
Arise
Bfall
Cremains almost stationary
DCannot be bredicted