Shows the shape of a progressive wave at time $t = 0$ . after a time $t = (1) / (80)$ , the particle at the origin has its maximum negative displacement.if the wave speed is $80$ units maximum negative displacement. If the wave speed is $80$ units, then find the equation of the progressive wave. .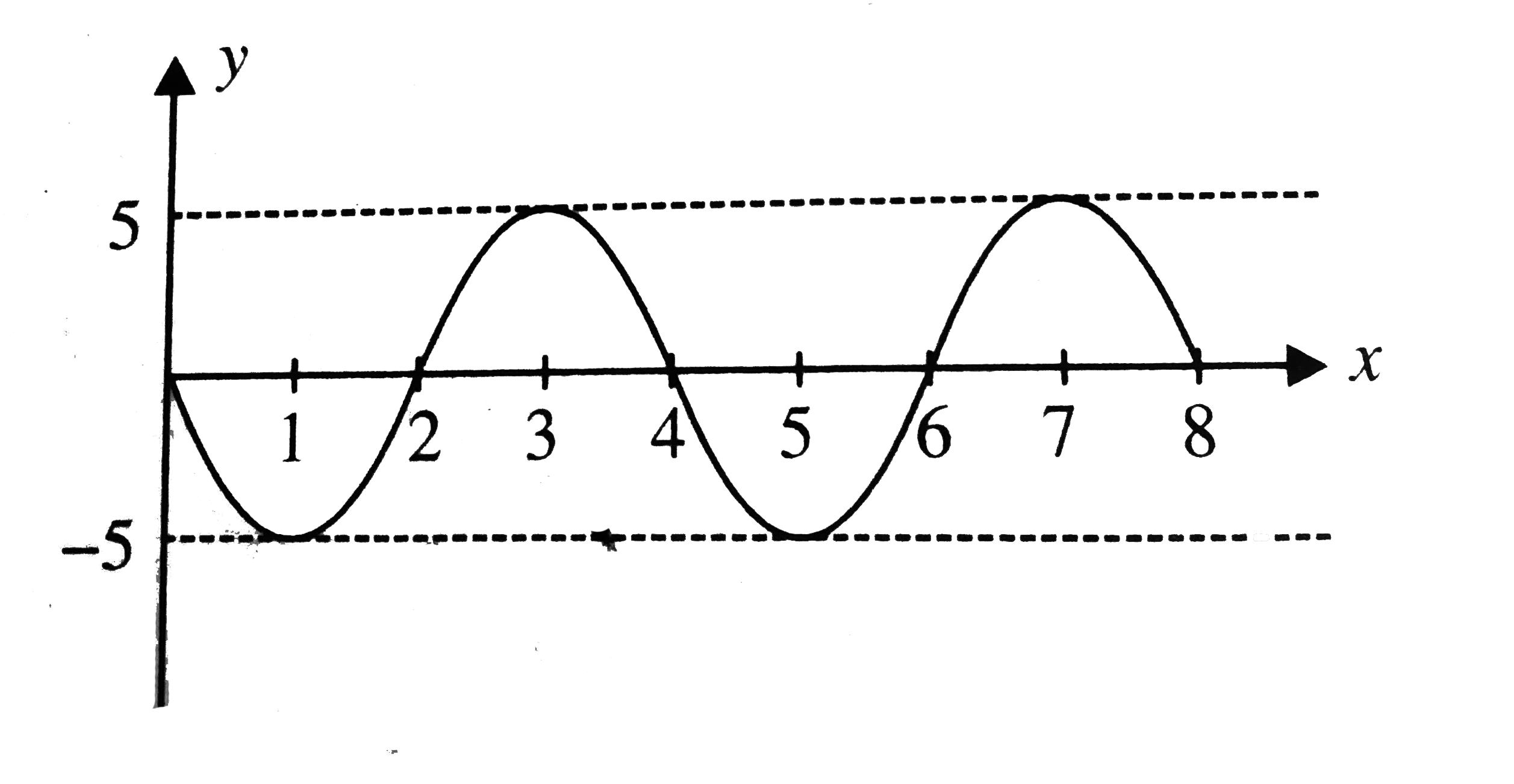

Video Solution

Text Solution

Verified by Experts

The wavelength $λ = 4$
we know, $T = \frac{λ}{v} = \frac{4}{80} = \frac{1}{20}$
Since after $t = \frac{1}{80} = \frac{T}{4}$ ,the particle at the origin is at its maximum negative, the entire wave pattern would have shifted by a distance of 1 unit $(λ / 4)$ to the left.
The wave moves in the negative $x -$ axis direction, from this we get $y = A sin [2 π (\frac{t}{t} + \frac{x}{λ}) + ϕ]$
we have initially $y = 0$ for $t = 0$ and $x = 0$
$ϕ = 0, π, 2 π$ etc.
Also, $y = - A,$ when $t = T / 4$ and $x = 0$
$∴ - A = A sin [2 π (\frac{1}{4}) + ϕ]$
$\Rightarrow \frac{π}{2} + ϕ = \frac{3 π}{2}, \frac{7 π}{2}$ etc.
or $ϕ = π, 3 π$ ,etc.
From Eqs. (i) and (ii), $ϕ = π$
Hence $y = 5 sin [2 π (\frac{t}{1 / 20} + \frac{x}{(4)}) + π]$

Updated on:21/07/2023