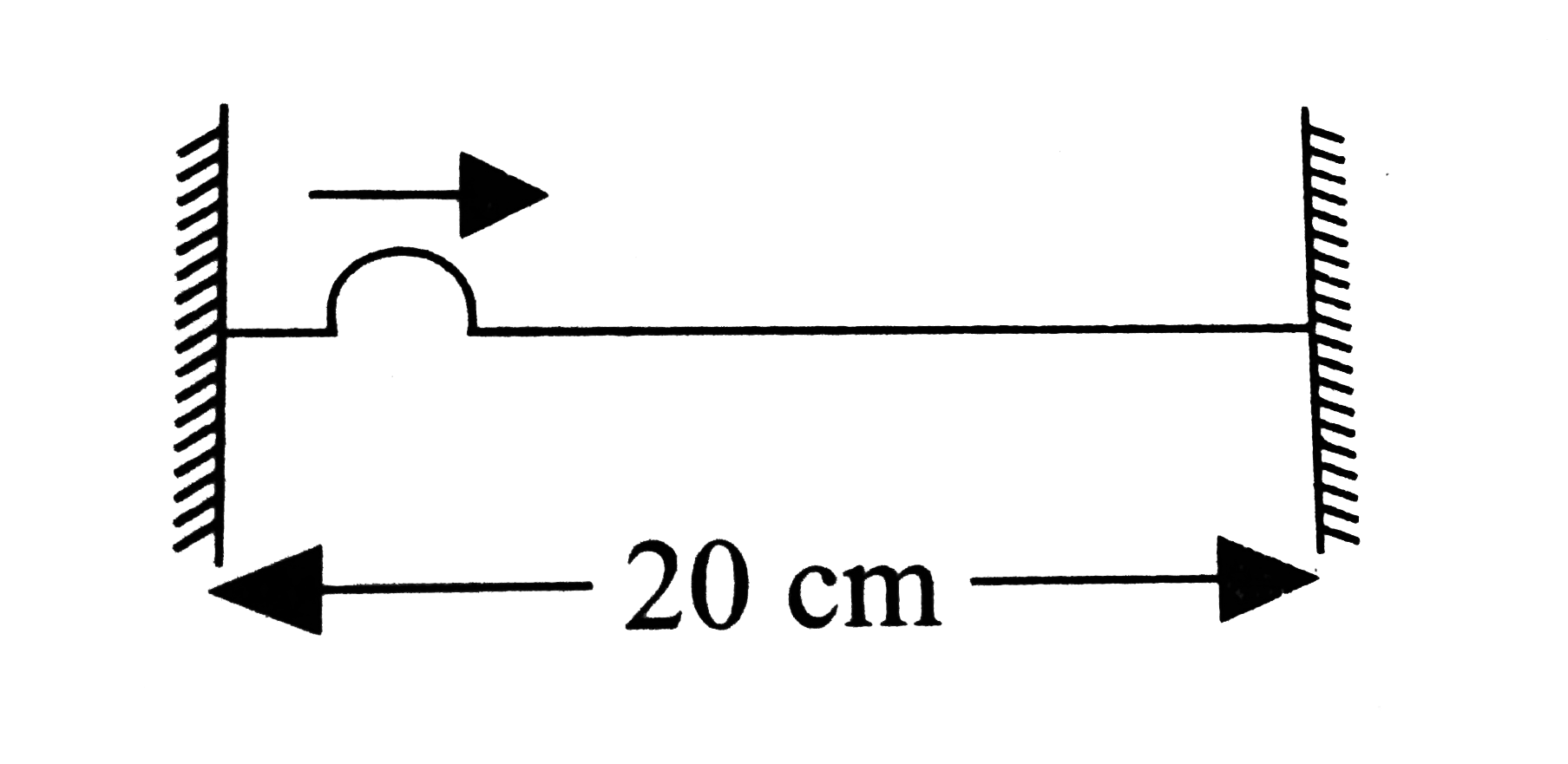
A taut string having tension 100 N and linear mass density 0.25kg/m is used inside a cart to generate a wave pulse starting at the left end, as shown. What should be the velocity of the cart so that pulse remains stationary w.r.t. ground.
The expression v=√Tμ gives the velocity of the wave in the string. Here the string is moving with cart.
velocity of pulse v=√T/μ=20m/s
This velocity is w.r.t. car.
Velocity of the pulse can be given as
Now →vP=→vPC+→v(CG)
0=20i+→vCG
→vCG=−20im/s
Hence, the cart should move with velocity 20m/s in the direction opposite to the pulse.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Example
9 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Exercise 5.1
9 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A taut string having tension 50 N and linear mass density 0.25 kg m−1 is used inside a cart to generate a wave pulse starting at the left end. What is the velocity of cart so that the pulse remains stationary with respect to the ground? (Consider the numerical value to be Vˆims−1, then find V) ( Take, √2=1.414.)
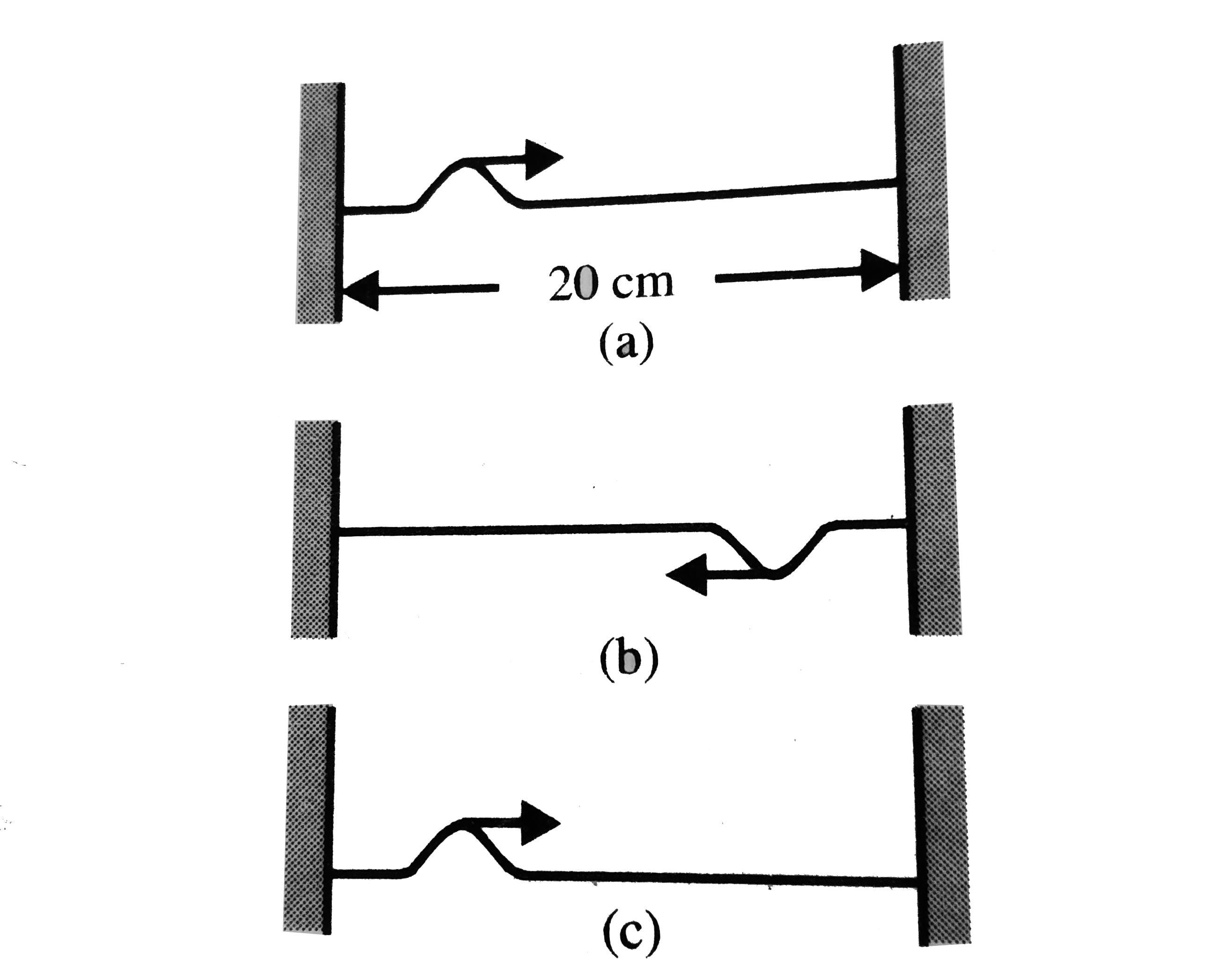
View SolutionA string of length 20cm and linear mass density 0.40g/cm is fixed at both ends and is kept under a tension of 16N.A wave pulse is produced at t=0 near an end as shown in figure which travels towards the other end.
when will the string have the shape shown in the figure again? (in ×10−2s)
View Solution
Knowledge Check
The tension in a string with a linear density of 0.0010 kg/m iş 0.40 N. A 100 Hz sinusoidal wave on this string has a wavelength of
A0.05cmB2.0cmC5.0cmD20cmFigure shown a clamped metal string of length 30cm and linear mass density 0.1kg/m. Which is taut at a tension of 40N. A small rider ( piece of paper ) is placed on string at point P as shown. An external vibrating tuning fork is brought near this string and oscillations of rider are carefully observed.
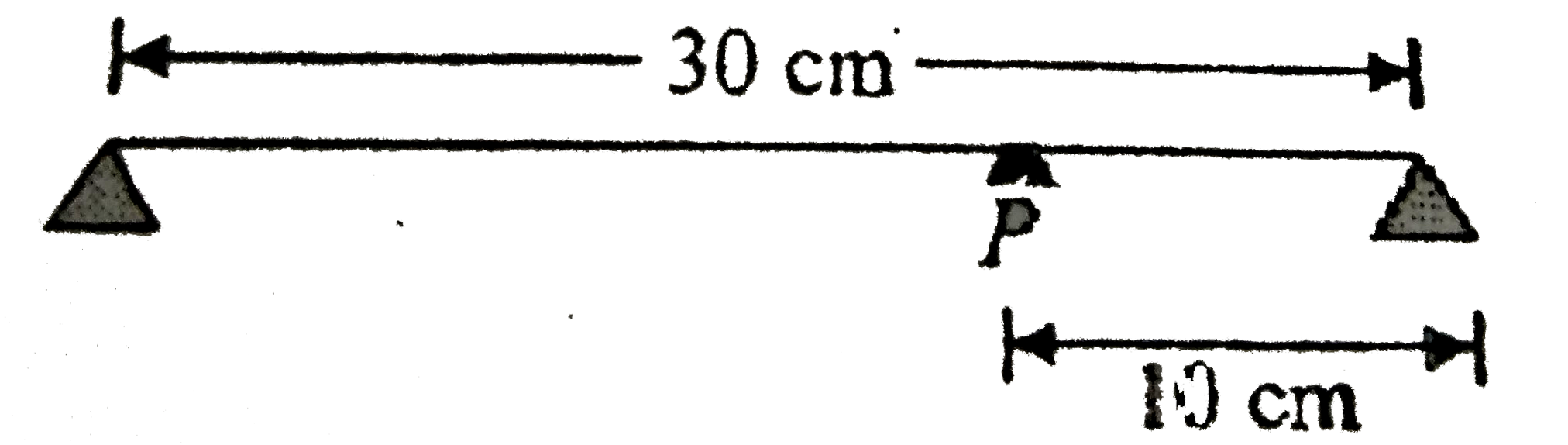
At which of the following frequencies the point P on string will have maximum oscillation amplitude among all points on string :A2003HzB100HzC200HzDNone of theseFigure shown a clamped metal string of length 30cm and linear mass density 0.1kg/m. Which is taut at a tension of 40N. A small rider ( piece of paper ) is placed on string at point P as shown. An external vibrating tuning fork is brought near this string and oscillations of rider are carefully observed.
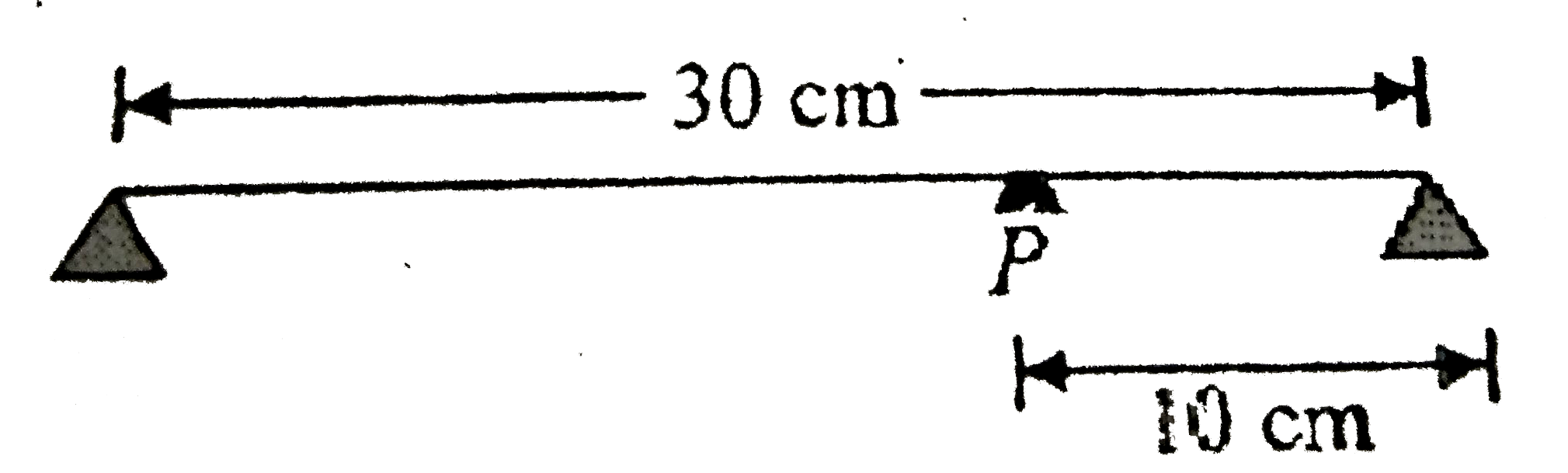
Now if the tensio in the string is made 160N, at which of the following frequencies of turning fork , rider will not vibrate at allA1003HzB50HzC200HzDNone of these
A string of length 20 cm and linear mass density 0.40gcm−1 is fixed at both ends and is kept under a tension of 16 N. A wave pulse is produced at t = 0 near an end as shown in figure, which travels towards the other end. (a) When will the string have the shape shown in the figure again ? (b) Sketch the shape of the string at a time half of that found in part (a).

View SolutionA string of length 20cm and linear mass density 0.40g/cm is fixed at both ends and is kept under a tension of 16N . A wave pulse is produced at t=0 near an ends as shown in Fig. 7.17 (b) , which travels towards the other end . When will the string have the shape shown in the Fig . ( c).
View SolutionA transverse harmonic wave is propagating along a taut string. Tension in the string is 50 N and its linear mass density is 0.02kgm–1 The string is driven by a 80Hz oscillator tied to one end oscillating with an amplitude of 1mm. The other end of the string is terminated so that all the wave energy is absorbed and there is no reflection
(a) Calculate the power of the oscillator.
(b) The tension in the string is quadrupled. What is new amplitude of the wave if the power of the oscillator remains same?
(c) Calculate the average energy of the wave on a 1.0m long segment of the string.
View SolutionA 4.0kg block is suspended from the celling of an elevator through a string having a linear mass density of 1.6×10−2kg/m. Find (a) speed (with respect to the string) with which pulse can proceed on the string it he elevator accelerates up at the rate of 6m/s. (b) in part (a) at some instant speed of lift is 40m/s in upward direction, then speed of the wave pulse with respect to ground at that instant is (Take =10m/s2)
View SolutionTwo smooth blocks of same mass are connected by an inextensible and massless string which is passing over a smooth pulley are kept in a lift is going down with
acceleration 'a' as shown in the fig What should be the value of a (in m/s2) so that acceleration of block A w.r.t. ground will be minimum? (g=10m/s2)
View Solution
