A rope of total mass m and length L is suspended vertically. Show that a transverse pulse travels the length of the rope in a time interval Δt=2√L/g. Sugestion: first find an expression for the wave speed at any point a distance x from the lower end by considering the rope's tension as rejulting from the weight of the segment below that point.
The tention will increase as elevation x increase, because the upper part of the hanging rope must support the weight of the lower part. Then the wave speed increase with height.
we will need to do an integral based on v=dx/dt to find the travel time of the variable-speed pulse.
we define x=0 at the bottom of the rope and x=L at the top of the rope. the tension in the rope at any point is the weight of the rope.below the point.we can thus write the tension unit length of the rope, which we assume uniform. the speed of the wave pulse at each point along the rope's length is, therefore,
but at each point, the wave propagates at the rate of
v=dxdt
so we substitute for v and generate the differential equation equation:
dxdt=√gxordt=dx√gx
Integrat∈g⊥hss,<br>Deltat=(1)/sqrt(g)int_(0)^(L)(dx)/sqrt(x)=[2sqrtx]_(0)^(L)/sqrt(g)=2sqrtL/g
what you have learned in mathematic class is another operation that can be done to both sides of an equation: integrating from one physical point to another. here the integral extends in time and space from the situation with the opulse reaching the top.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Example9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Exercise 5.19videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A uniform rope of mass m and length L is attached to the ceiling of a lift. The other end of the rope is attached to a block of mass M. The lift is moving up with a constant acceleration a0. Find the tension in the rope at a distance x from the ceiling as shown in the diagram.
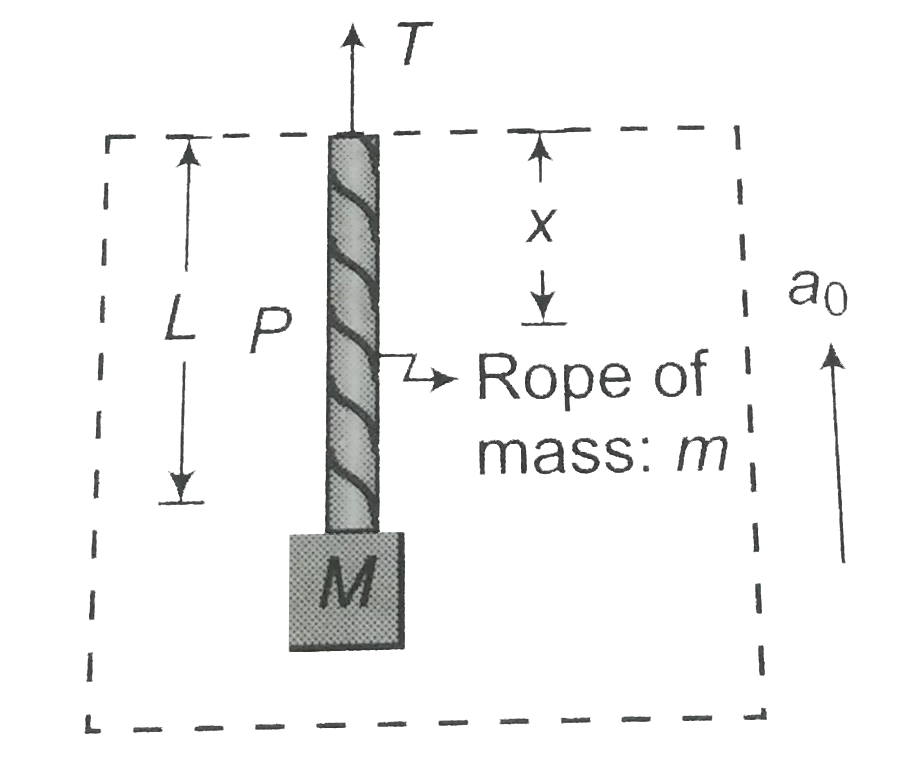 View Solution
View SolutionA uniform rope of mass m and length L hangs from a celling. (a) Show that the speed of a transverse wave on the rope is a function of y, the distance from the lower end, and is given by t=2√L/g.
View Solution
Knowledge Check
- Question 1 - Select One
A rope of length L and mass m hangs freely from the ceiling. The velocity of transverse wave as a function of position x from the bottom is proportional to
ATBT(√2−1√2)CT√2DT2 - Question 2 - Select One
A uniform rod of length L and weight W is suspended horizontally by two vertical ropes as shown. The first rope is attached to the left end of the rod while the second is attached to the left end of the rod while the second rope is attached a distance L/4 from the right end. The tension in the second rope is.
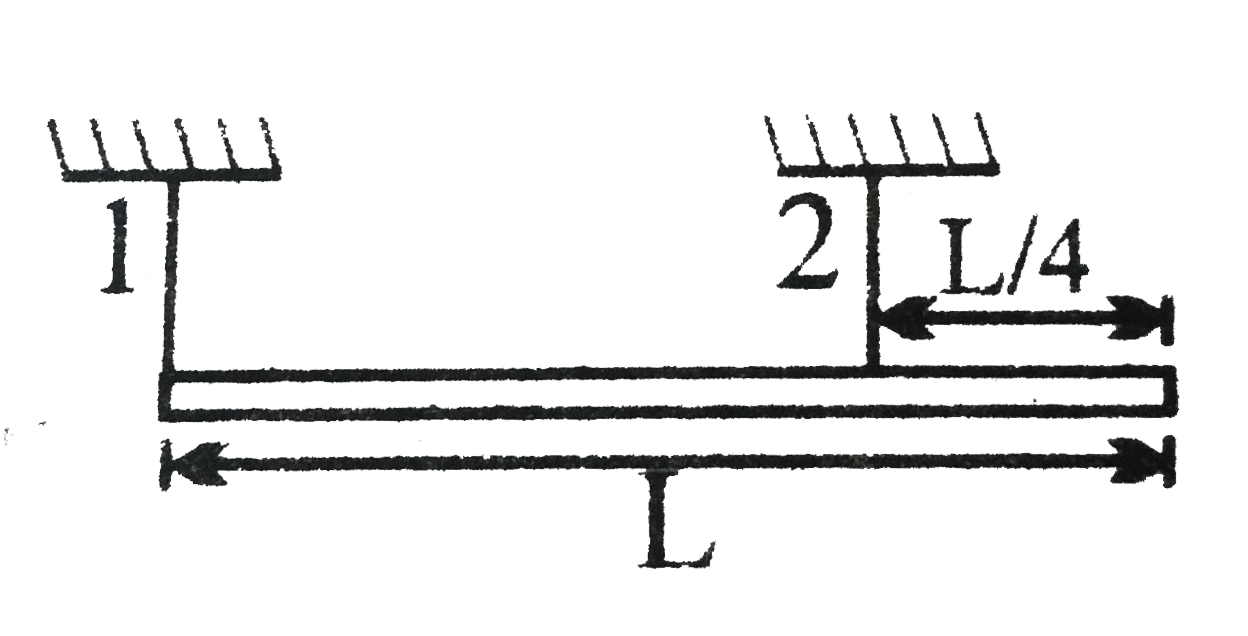 .AW2BW4CW3D2W3
.AW2BW4CW3D2W3 - Question 3 - Select One
A straight rope of length 'L' is kept on a frictionless horizontal surface and a force 'F' is applied to one end of the rope in the direction of its length and away from that end. The tension in the rope at a distance 'l' from that end is
AFlBLFlC(1−lL)FD(1+lL)F
A straight rope of length 'L' is kept on a frictionless horizontal surface and a force 'F' is applied to one end of the rope in the direction of its length and away from that end. The tension in the rope at a distance 'I from that end is 9
View SolutionA uniform rope of mass m and length L hangs from a ceiling. (a) Show that the speed of a transverse wave on the rope is a function of y, the distance from the lower end, and is given by v=√gy. (b) Show that the time a transverse wave takes to travel the length of the rope is given by t=2√L/g.
View SolutionA uniform rope of mass 0.1kg and length 2.45m hangs from a ceiling.
(a) Find the speed of transverse wave in the rope at a point 0.5m distant from the lower end.
(b) Calculate the time taken by a transverse wave to travel the full length of the rope.
 View Solution
View SolutionA rope of length L and mass m hangs freely from the ceiling. The velocity of transverse wave as a function of position x-along the rope is proportional to
View SolutionA rope of length L and mass m hangs freely from the ceiling. The velocity of transverse wave as a function of position x from the bottom is proportional to
View Solution

