Statement I : A standing wave pattern is formed in a string . The power transfer through a point ( other than node and antinode) is zero always.
Statement II : At antinode is perpendicular to this velocity .
Statement I is true , Statement II is true , Statement II is a correct explanation for Statement I.
Statement I is true , Statement II is true , Statement II is NOT a correct explanation for Statement I.
Statement I is true , Statement II is false.
Statement I : is false , Statement II is true.
The correct Answer is:D
At node v=0 , at antinode tension perpendicular to velocity therefore , at these points power =0,(P=F.V) At other points P≠0.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension55videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Integer9videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple26videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - 241videos
THERMODYNAMICS
Similar Questions
A standing wave pattern is formed on a string.One of the waves is given by equation y1=acos(ωt−kx+π3) ,then the equation of the other wave such that x=0 a node is formed is-
View SolutionFigure shows the standing waves pattern in a string at t = 0. Find out the equation of the standing wave where the amplitude of antinode is 2A.
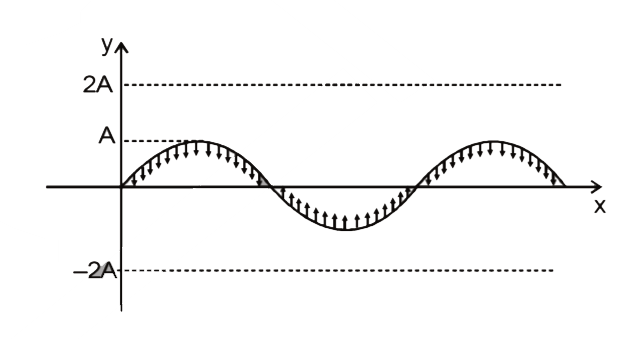 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
S1 : A standing wave pattern if formed in a string. The power transfer through a point (other than node and antinode) is zero always
S2: if the equation of transverse wave is y=sin2π[t0.04−x40], where distance is in cm. time in second, then the wavelength will be 40 cm.
S3: if the length of the vibrating string is kept constant, then frequency of the string will be directly proportional to √TAFTTBTTFCTFTDFFF - Question 2 - Select One
Statement I : In standing waves on a string , the medium particles , i.e., different striung elements remain at rest .
Statement II : In standing waves all the medium particles attain maximum velocity twice in one cycle.AStatement I is true , Statement II is true , Statement II is a correct explanation for Statement I.BStatement I is true , Statement II is true , Statement II is NOT a correct explanation for Statement I.CStatement I is true , Statement II is false.DStatement I : is false , Statement II is true. - Question 3 - Select One
Statement-1 : in standing wave average rate of transfer of energy at any cross-section is zero.
because
Statement-2 : instantaneous rate of energy transfer at node is equal to zero.AStatement -1 is True, statement -2 is True, Statement -2 is a correct explanation for Statement -1.BStatement -1 is True, Statement -2 is True , Statement-2 is Not a correct explanation for statement-9CStatement -1 is True, statement -2 is FalseDStatement -1 is False, Statement -2 is True.
Statement-I : When velocity of a particle is zero then acceleration of particle is zero.
Statement-II : Acceleration is equal to rate of change of velocity.View SolutionStatement I : In a standing wave on a string , the spacing between nodes is Δx. If the tension in string is increased wave same as before , then the separation between nearest node and antinode will be Δx.
Statement II : Spacing between nodes ( consecutive) in the standing wave is equal to half of the wavelength of component waves.View SolutionIn a stationary wave that forms as a result of reflection of wave from an obstacle, the ratio of this amplitude at an antinode to the amplitude at node is n. The fraction of energy reflected is
View SolutionStatement I : The transverse wave is traveling along a string in the positive x-axis is shown. Points (A & P1) moving downward and point (C &P2) moving upward.
Statement II : In a wave propagating in positive x direction, the points with +ve slope move downward and vice versa.
 View Solution
View SolutionWhen a string is vibrating in a standing wave pattern , the power transmitted across an antinode , compared to the power transmitted across a node , is
View Solution

