In the arrangement shown in Fig. 7.100 , mass can be hung from a string with a linear mass density of 2×10−3kg/m that passes over a light pulley . The string is connected to a vibrator of frequency 700Hz and the length of the string between the vibrator and the pulley is 1m.

If the standing waves are observed , the largest mass to be hung is
16kg
25kg
32kg
400kg
The correct Answer is:D
For largest mass , p=1
n=p2L√Tμ
700=p2L√Tμ
m=2×10−3kg/m,L=1m
T=[(700)2×4×1×2×10−3]=3920N
Largest mass to be hang , Mmax=3920/9.8=400kg
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICSChapter:SUPERPOSITION AND STANDING WAVESExercise:Integer
Explore 9 Videos
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICSChapter:SUPERPOSITION AND STANDING WAVESExercise:Multiple Correct Answers Type
Explore 5 Videos
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICSChapter:SUPERPOSITION AND STANDING WAVESExercise:Assertion - Reasoning
Explore 6 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICSChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
- THERMODYNAMICSBook:CENGAGE PHYSICSChapter:THERMODYNAMICSExercise:24
Explore 1 Video
Similar Questions
In the given sonometer arrangentent, a mass M can be hung from a string, that passes over a light pulley. The string connected to a vibrator having constant frequency. When the value of M is either 16kg or 25kg standing waves are observed, however, no standing waves are observed with any mass between these values. The largest mass for which standing waves could be observed is n×102kg and frequendy of vibrator is f(Hz) then find the numerical value of n×f (mass per unit length for string is 10−3 kg / m and length L=2 m ).
'(##CEN_KSR_PHY_JEE_C17_E01_037_Q13##) A block string system is shown in the figure. Mass of block A,B and C is 5 kg, 2 k , and 2kg respectively. Mass of rod is given as 1kg and its length is 1m. A wave transverse is transmitted in the rod in between the block B and C once in forward and once backward direction. Time for string wave to reach one end to other end in forward direction is 2/(√z)(√x−√y) sec. Find (x+y+z)
'(##CEN_KSR_PHY_JEE_C17_E01_036_Q12##)'
View SolutionThe linear mass density of the string shown in the figure is μ=1g/m. One end (A) of the string is tied to a prong of a tuning fork and the other end carries a block of mass M. The length of the string between the tuning fork and the pulley is L=2.0m. When the tuning fork vibrates, the string resonates with it when mass M is either 16 kg or 25 kg. However, standing waves are not observed for any other value of M lying between 16 kg and 25 kg. Assume that end A of the string is practically at rest and calculate the frequency of the fork.

View Solution
Knowledge Check
In the arrangement shown in Fig. 7.100 , mass can be hung from a string with a linear mass density of 2×10−3kg/m that passes over a light pulley . The string is connected to a vibrator of frequency 700Hz and the length of the string between the vibrator and the pulley is 1m.

If the mass suspended is 16kg , then the number of loops formed in the string isA1B3C5D8In the arrangement shown in Fig. 7.100 , mass can be hung from a string with a linear mass density of 2×10−3kg/m that passes over a light pulley . The string is connected to a vibrator of frequency 700Hz and the length of the string between the vibrator and the pulley is 1m.

The string is set into vibrations and represented by the equation y=6sin(πx10)cmcos(14×103πt) where x ane y are in cm , and t in s, the maximum displacement at x=5m from the vibrator isA6cmB3cmC5cmD2cmA string of length 0.5m and linear density 10−4 kg/m is stretched under a tension of 100 N. If the frequency of the vibration of the string is 4000 Hz, how many loops are formed on the string?
A1B4C2D6
The mass suspended from the stretched string of a sonometer is 2 kg and the frequency of the tuning fork used is 100 Hz. If the length of the string between the wedges is 50 cm,
find the linear mass density of the string (Taking g = 10ms−2).
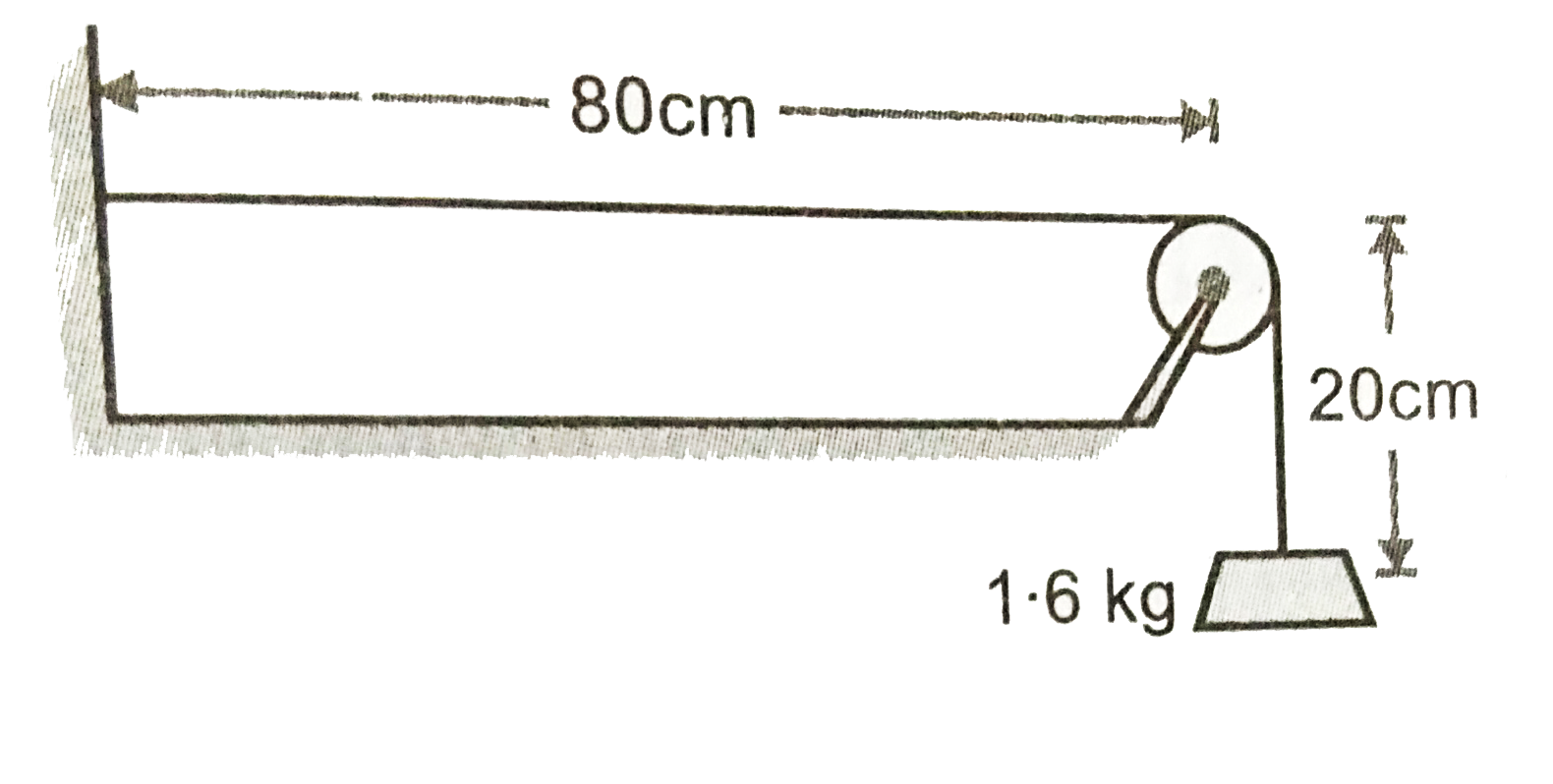
View SolutionA 100 cm long wire of mass 40 g supports a mass of 1.6 kg as shown in figure . Find the fundamental frequency of the portion of the string between the wall and the pulley. Take g=10m/s2.
View SolutionIn the arrangement shown in fig. m1=1kg,m2=2kg. Pulleys are massless and strings are light. For what value of M, the mass m1 moves with constant velocity.

View SolutionIn the figure shown, a particle of small mass m is joined to a very heavy mass M (M >> m) by a light string passing over a light pulley. The total downward force on the pulley by the string is :
View SolutionStanding wavs are produced in a stretched string of length 8 m. If the string vibrates in 4 loops and the wave velocity is 20 m/s, then the frequency of the string will be
View Solution