Suppose a, b, c are three distinct real numbers and is a real quadratic polynomial such that lt brgt
![Suppose a, b, c are three distinct real numbers and f(x) is a real quadratic polynomial such that lt brgt [[4a^(2) , 4a, 1],[4b^(2), 4b, 1 ],[4c^(2), 4c, 1]][[f(-1)],[f(1)],[f(2)]]= [[3a^(2),+3a],[3b^(2) , +3b],[3c^(2),+ 3c]].](https://d10lpgp6xz60nq.cloudfront.net/physics_images/ARH_SKG_ALG_C08_E05_072_Q01.png)
Text Solution
The correct Answer is:
On comparing, we get
and
It is clear that a, b, c are the roots of
then
Let
Now,
Graph of
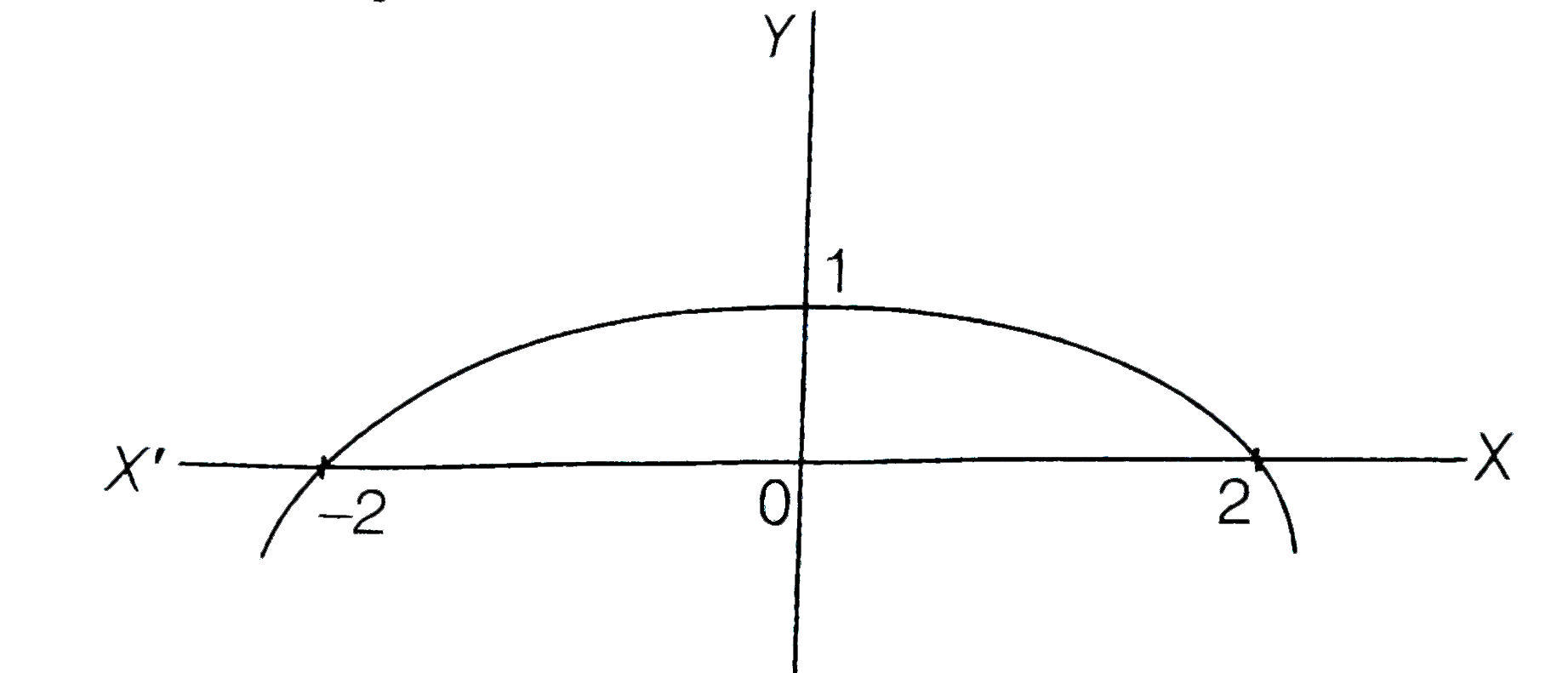
(A) x- coordinates of the point intersection of with
ther X- axis are - 2 and 2.
(B) Area
(C) Maximum value of is 1.
(D) Length of intercept on the X_ axis is 4.
|
Topper's Solved these Questions
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Statement I And Ii Type Questions)
10 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Subjective Type Questions)
14 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Single Integer Answer Type Questions)
10 Videos
- MATHEMATICAL INDUCTION BOOK - ARIHANT MATHSCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
31 Videos
Similar Questions
Knowledge Check
If a,b,c are positive real numbers and 2a+b+3c=1, then the maximum value of a4b2c2 is equal to
A13⋅48B19⋅47C19⋅48D127⋅48If a,b,c are non-zero real numbers, then the minimum value of the expression (a8+4a4+1)(b4+3b2+1)(c2+2c+2)a4b2 equals
A12B24C30D60Statement 1: F a,b,c are real positive numbers with abc =1 and ∀T =1 where
A=⎡⎢⎣abcbcacab⎤⎥⎦ then a3+b3+c3=4.
Statement 2: 1a+1b+1C=0.AStatement -1 is True ,Statement -2 is True ,Statement -2 is corect explanation for Statement -1BStatement-1 is True .Statement -2 is true Statement -2 is Not a correct explanation for Statement -1CStatement-1 is true Statement-2 is FalseDStatement-1 is False , Statement-2 is True
If x≠1 and f(x)=x+1x−1 is a real function, then f(f(f(2))) is (a) 1 (b) 2 (c) 3 (d) 4
View SolutionIf a,b,c are non-zero real numbers, then the minimum value of the expression (a8+4a4+1)(b4+3b2+1)(c2+2c+2)a4b2 equals
View SolutionIf A=[1234],B=[2345] and 4A−3B+C=O, then C=[213−1].
View Solution[ The number of real solutions of x^(2)-4|x|-2=0 is [ (a) 1, (b) 2 (c) 3, (d) 4]]
View SolutionLet f(x)=ax2+bx+c is a quadratic polynomial if 3a+√3b+c=1and4a+2b+c=0 then which ofthe following is/are not possible-
View Solution
