If ¯¯¯E and ¯¯¯F are the complementary events of E and F respectively and if 0<P(F)<1, then
P(¯¯¯EF)+P(¯¯¯EF)=1
P(EF)+P(E¯¯¯F)=1
P(¯¯¯EF)+P(E¯¯¯F)=1
P(E¯¯¯F)+P(¯¯¯E¯¯¯F)=1
The correct Answer is:(a,b)
Step by step video, text & image solution for If barE and barF are the complementary events of E and F respectively and if 0 < P(F)<1, then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Passage Based Questions)
27 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Single Integer Answer Type Questions)
10 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Single Option Correct Type Questions)
29 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
Similar Questions
Knowledge Check
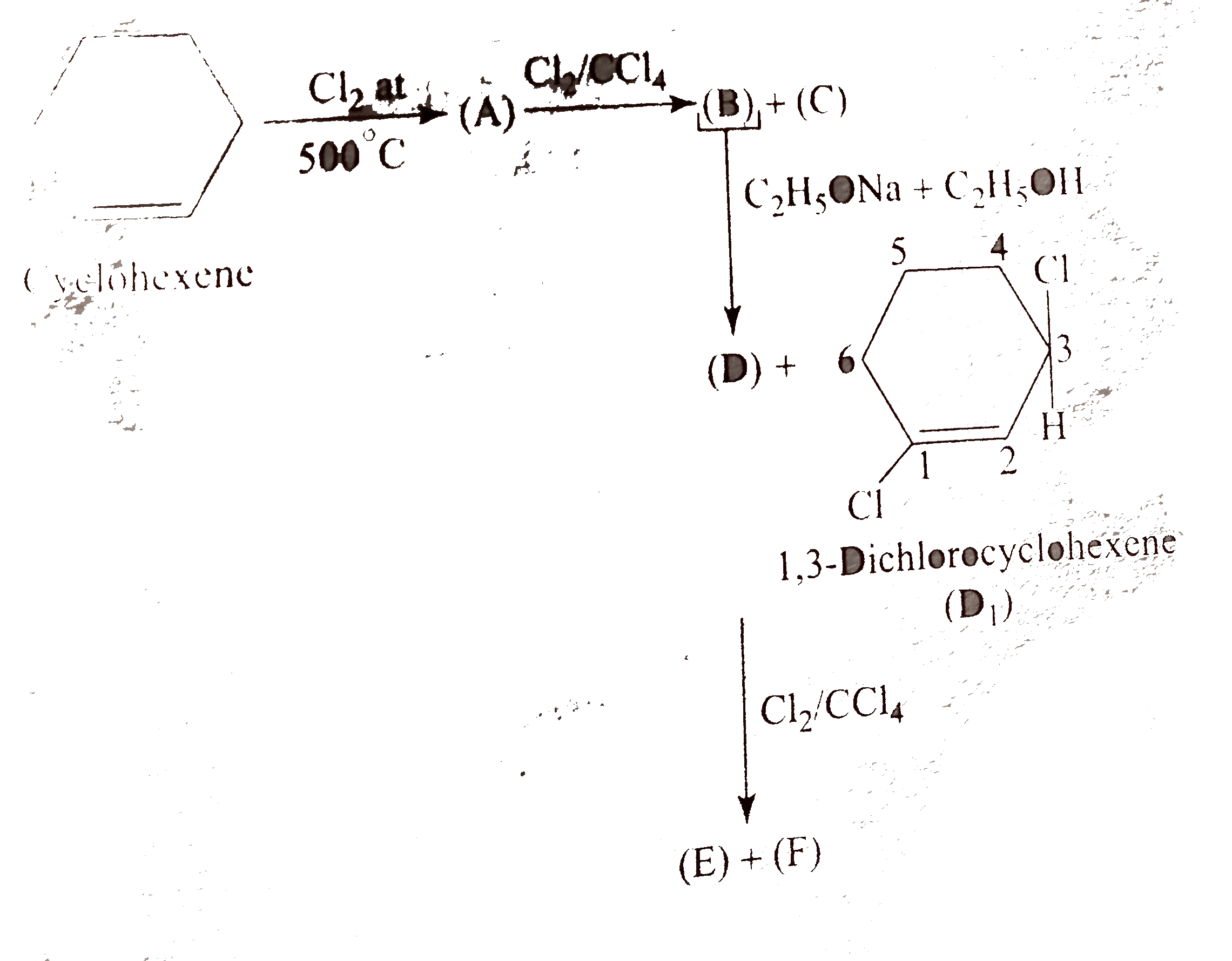
(E) and (F), respectively, are :A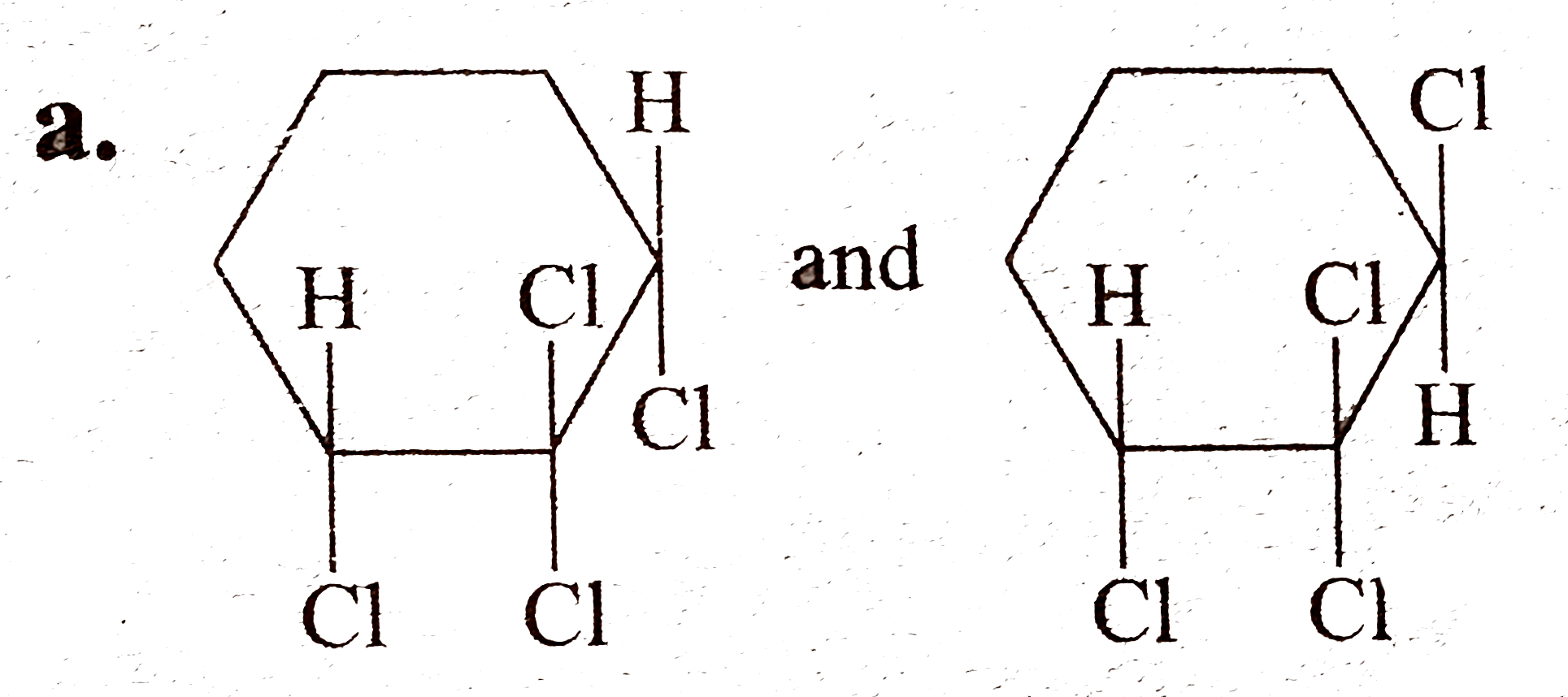 B
B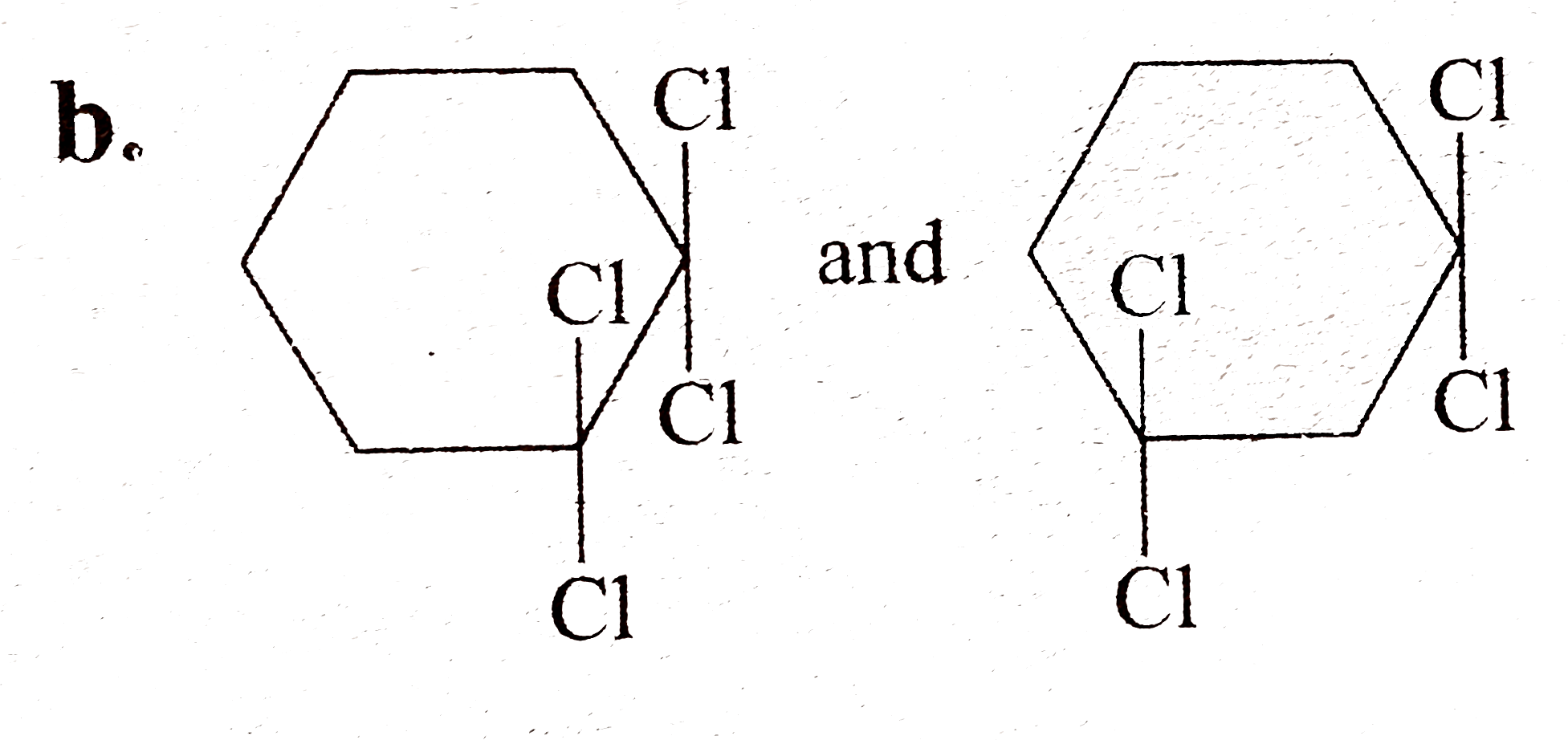 C
C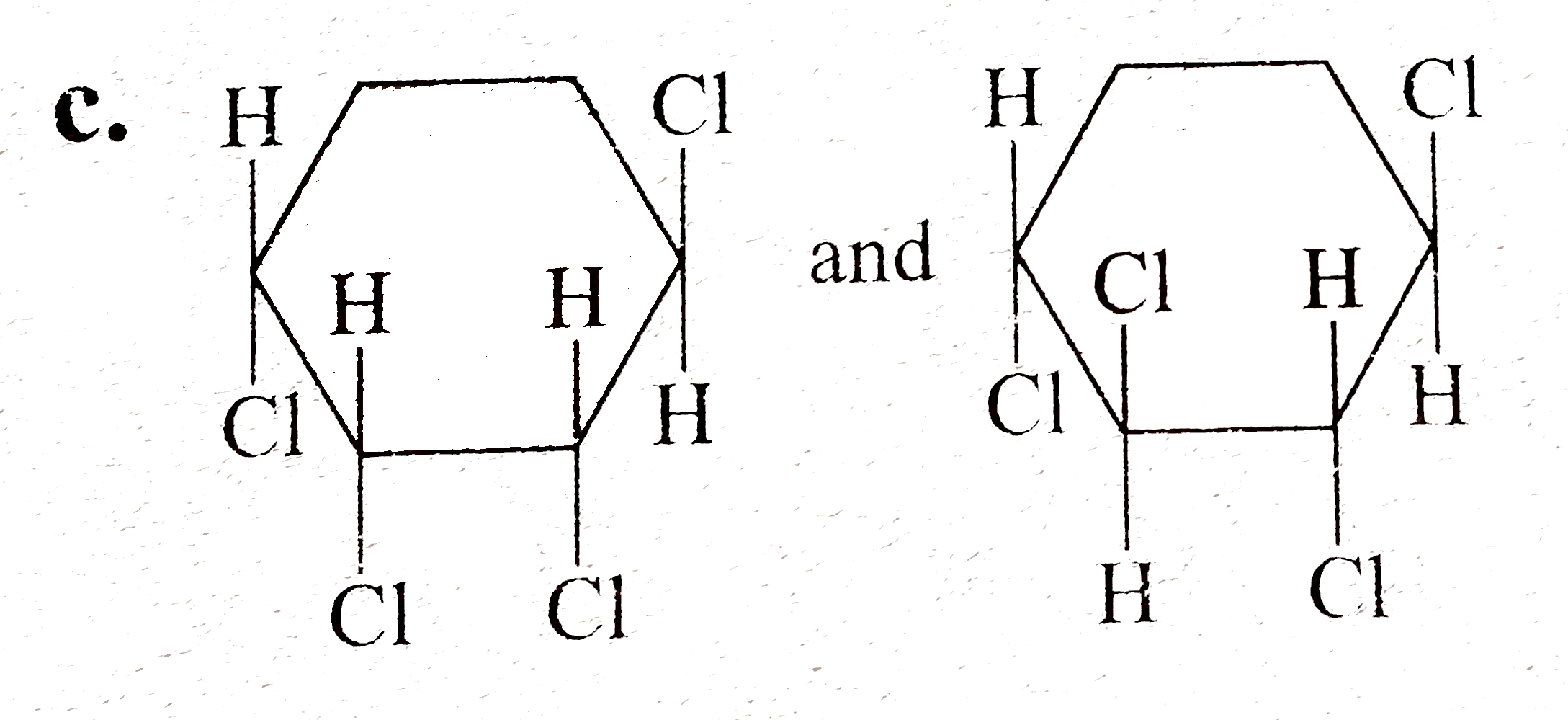 D
D
If F0 andFe are the focal lengths of the objective and eye-piece respectively for a Galilean telescope, its magnifying power is about
AF0+FeBF0×FeCF0/FeD12F0+FeIf A',B' are complementary events of two independent events A, B, then P(A∪B)=
A1−P(A)P(B')B1−P(A')P(B)C1−P(A')P(B')DP(A')+P(B')
If ¯¯¯E & ¯¯¯F are complementary events of events E&F respectively then P(EF)+P(¯¯¯EF)
View SolutionIf A and B are two complementary events, then what is the relation between P(A) and P(B).
View SolutionIf E and F are independent events with P(E)=p and P(F)=2p and P(exactly one of E,F) =56 then find the value of p
View SolutionIf E and F are two independent events then prove that E and F' are also independent events.
View SolutionUnits of van der Waal's constants a and bare respectively
View Solution
