Consider a circle , in which a point P is lying inside the circle such that ( as shown in figure ) .
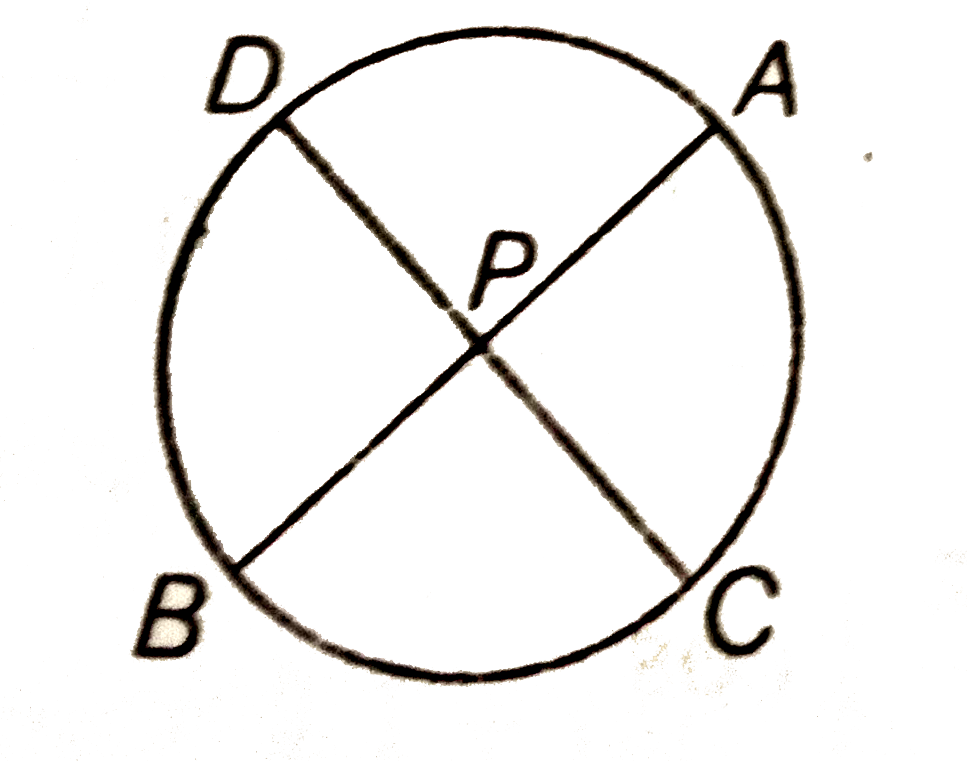
On the basis of above information , answer the questions
If then x is equal to
The correct Answer is:D
Step by step video & image solution for Consider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) . On the basis of above information , answer the questions If log_(PA) x=2 , log_(PB)x=3, log_(x) PC=4 , then log_(PD) x is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise For Session 1
15 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise For Session 2
8 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
44 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
19 Videos
Similar Questions
Consider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
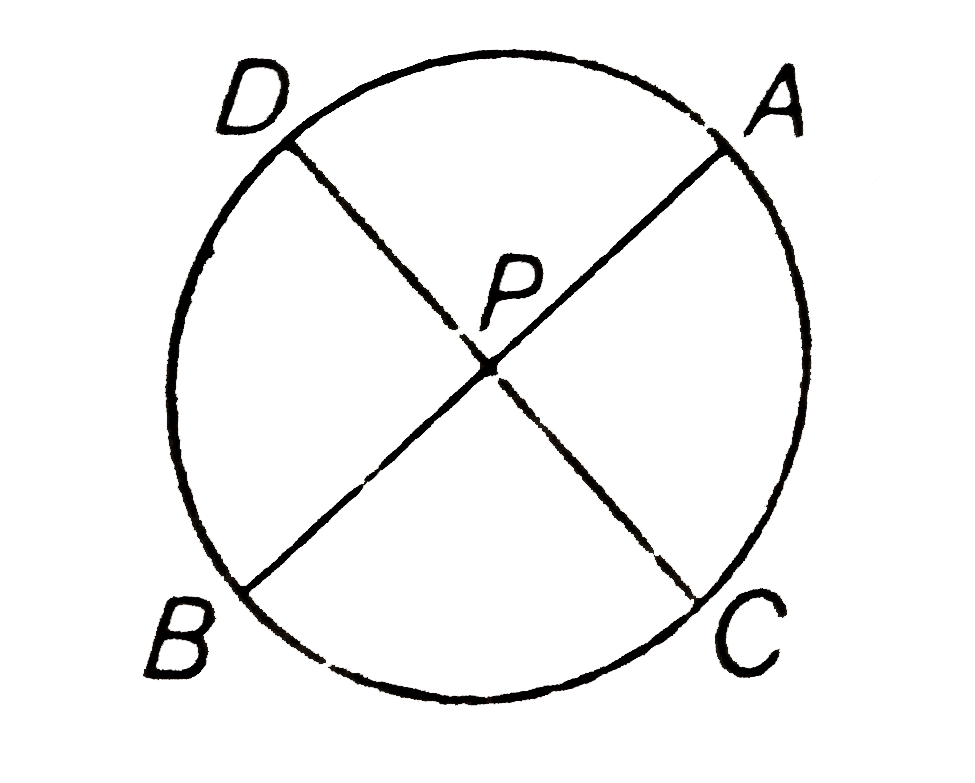
On the basis of above information , answer the questions
Let PA=4 , PB=3 cm and CD is diameter of the circle having the length 8. If PC>CD , then PCPD is equal to
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
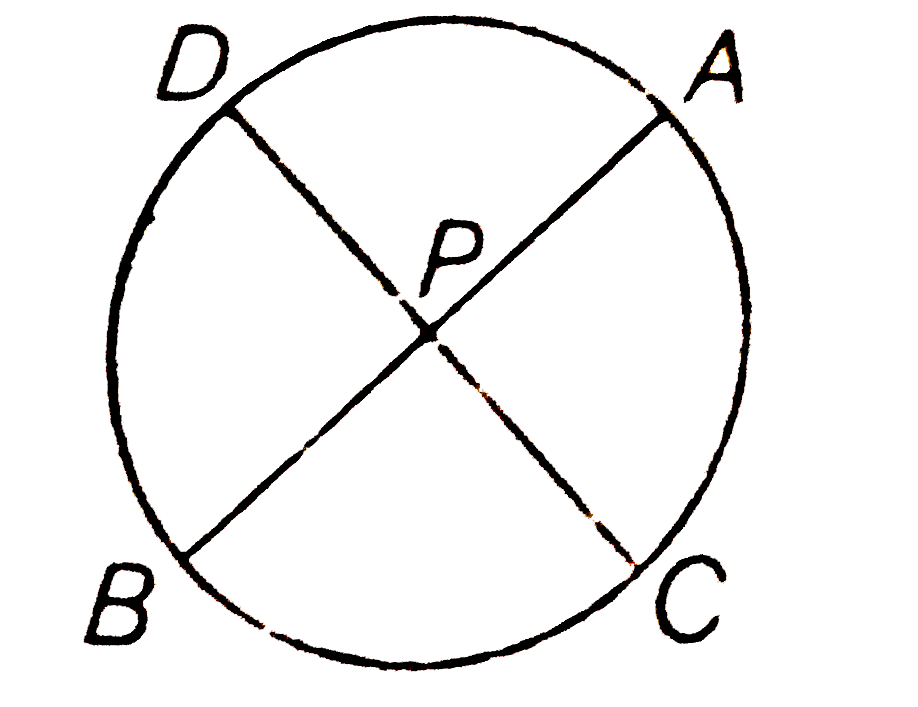
On the basis of above information , answer the questions
If PA=|cosθ+sinθ|andPB=|cosθ−sinθ|, then maximum value of (PC)(PD) , is equal to
View Solution
Knowledge Check
If log4(log2x)+log2(log4x)=2, then find logx4.
A2B12C1D0If log4x+log8x2+log16x3=232, then logx8=
A2B12C3D34If logxa,ax/2,logbx are in G.P. then x is equal to
Aloga(logba)Blog(loga)−log(logb)logaClogb(logab)Dnone of these
If 3log2x=4log2x−1 then x is equal to
View SolutionIf log2x×log3x=log2x+log3x, then find x .
View SolutionIf x>1andlog2x,log3x,logx16 are in GP, then what is x equal to ?
View Solution(log4x−2)⋅log4x=32(log4x−1)
View SolutionIf log32,log3(2x−5) and log3(2x−7/2) are in AP then x is equal to:
View Solution
