The area bounded between the parabolas x2=y4andx2=9y and the straight line y=2 is (1) 20√2 (2) 10√23 (3) 20√23 (4) 10√2
To find the area bounded between the parabolas x2=y4 and x2=9y, and the straight line y=2, we can follow these steps:
Step 1: Identify the curves
The equations of the curves are:
1. x2=y4 (which can be rewritten as y=4x2)
2. x2=9y (which can be rewritten as y=x29)
Step 2: Find the points of intersection
To find the points of intersection of the two parabolas, set them equal to each other:
4x2=x29
Multiplying through by 36 to eliminate the fraction:
144x2=x2
This simplifies to:
144x2−x2=0⟹143x2=0⟹x=0
Now, substituting x=0 into either equation to find y:
y=4(0)2=0andy=(0)29=0
So, one intersection point is (0,0).
Next, we need to find the intersection with the line y=2:
1. For y=2 in y=4x2:
2=4x2⟹x2=12⟹x=±1√2=±√22
2. For y=2 in y=x29:
2=x29⟹x2=18⟹x=±√18=±3√2
Step 3: Set up the integral
The area bounded by the curves and the line can be calculated using the integral:
Area=2∫20(x2−x1)dy
Where x2 is the right parabola x2=9y and x1 is the left parabola x2=y4.
From the equations:
- x2=3√y
- x1=√y4=√y2
Thus, the area becomes:
Area=2∫20(3√y−√y2)dy
=2∫20(6√y2−√y2)dy=2∫20(5√y2)dy
=5∫20√ydy
Step 4: Calculate the integral
The integral of √y is:
∫√ydy=23y3/2
Evaluating from 0 to 2:
=23(2)3/2−23(0)3/2=23(2√2)=4√23
Step 5: Final area calculation
Thus, the total area is:
Area=5⋅4√23=20√23
Conclusion
The area bounded between the parabolas and the line is:
20√23
The equations of the curves are:
1. x2=y4 (which can be rewritten as y=4x2)
2. x2=9y (which can be rewritten as y=x29)
To find the points of intersection of the two parabolas, set them equal to each other:
4x2=x29
Multiplying through by 36 to eliminate the fraction:
144x2=x2
This simplifies to:
144x2−x2=0⟹143x2=0⟹x=0
Now, substituting x=0 into either equation to find y:
y=4(0)2=0andy=(0)29=0
So, one intersection point is (0,0).
1. For y=2 in y=4x2:
2=4x2⟹x2=12⟹x=±1√2=±√22
2. For y=2 in y=x29:
2=x29⟹x2=18⟹x=±√18=±3√2
The area bounded by the curves and the line can be calculated using the integral:
Area=2∫20(x2−x1)dy
Where x2 is the right parabola x2=9y and x1 is the left parabola x2=y4.
- x2=3√y
- x1=√y4=√y2
Area=2∫20(3√y−√y2)dy
=2∫20(6√y2−√y2)dy=2∫20(5√y2)dy
=5∫20√ydy
The integral of √y is:
∫√ydy=23y3/2
Evaluating from 0 to 2:
=23(2)3/2−23(0)3/2=23(2√2)=4√23
Thus, the total area is:
Area=5⋅4√23=20√23
The area bounded between the parabolas and the line is:
20√23
Topper's Solved these Questions
Similar Questions
Knowledge Check
The area bounded between the parabola x2=y4 and x2=9y and the straight line y=2 is
A20√2B10√23C20√23D10√2The graph of straight line y=√3x+2√3 is :
A B
B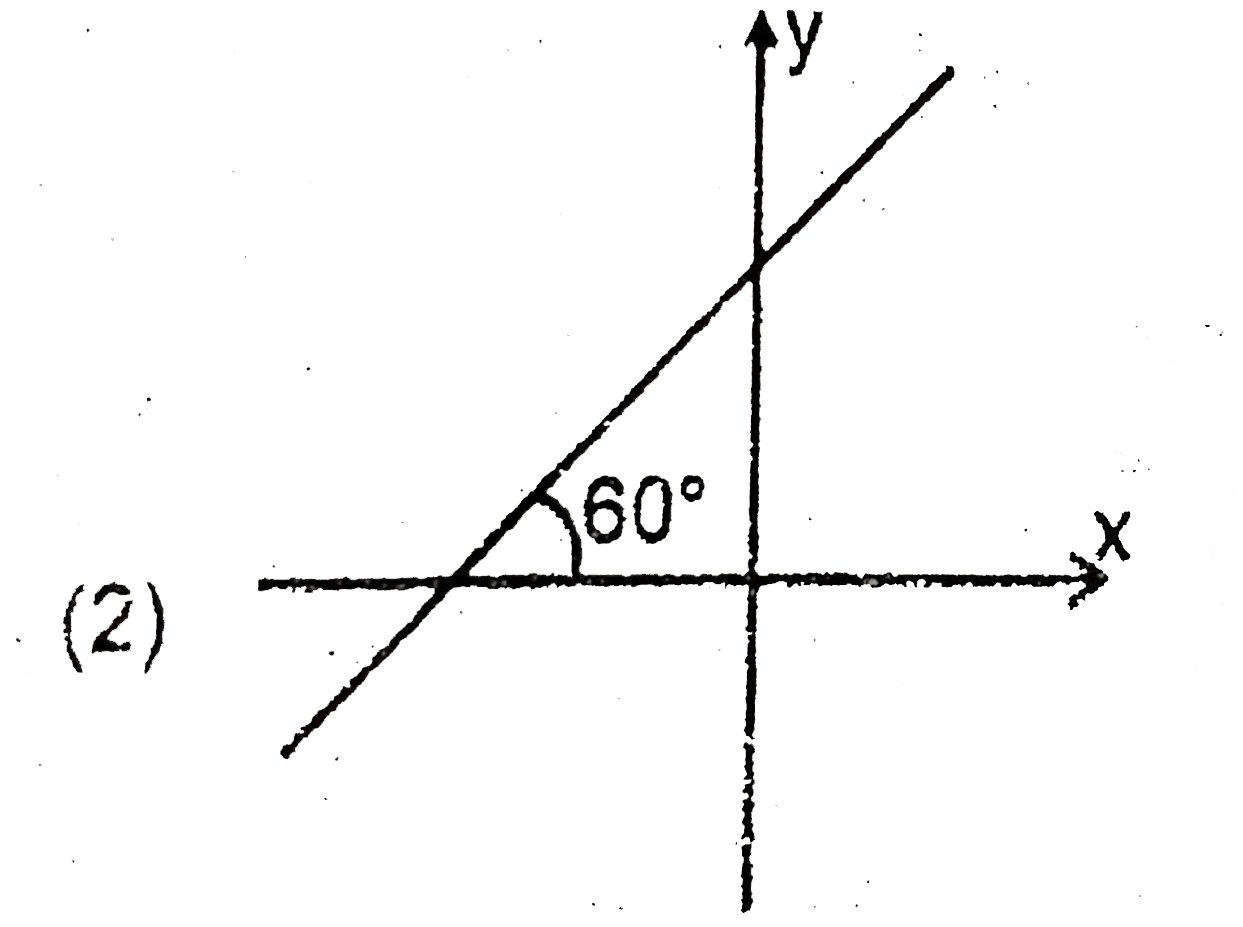 C
C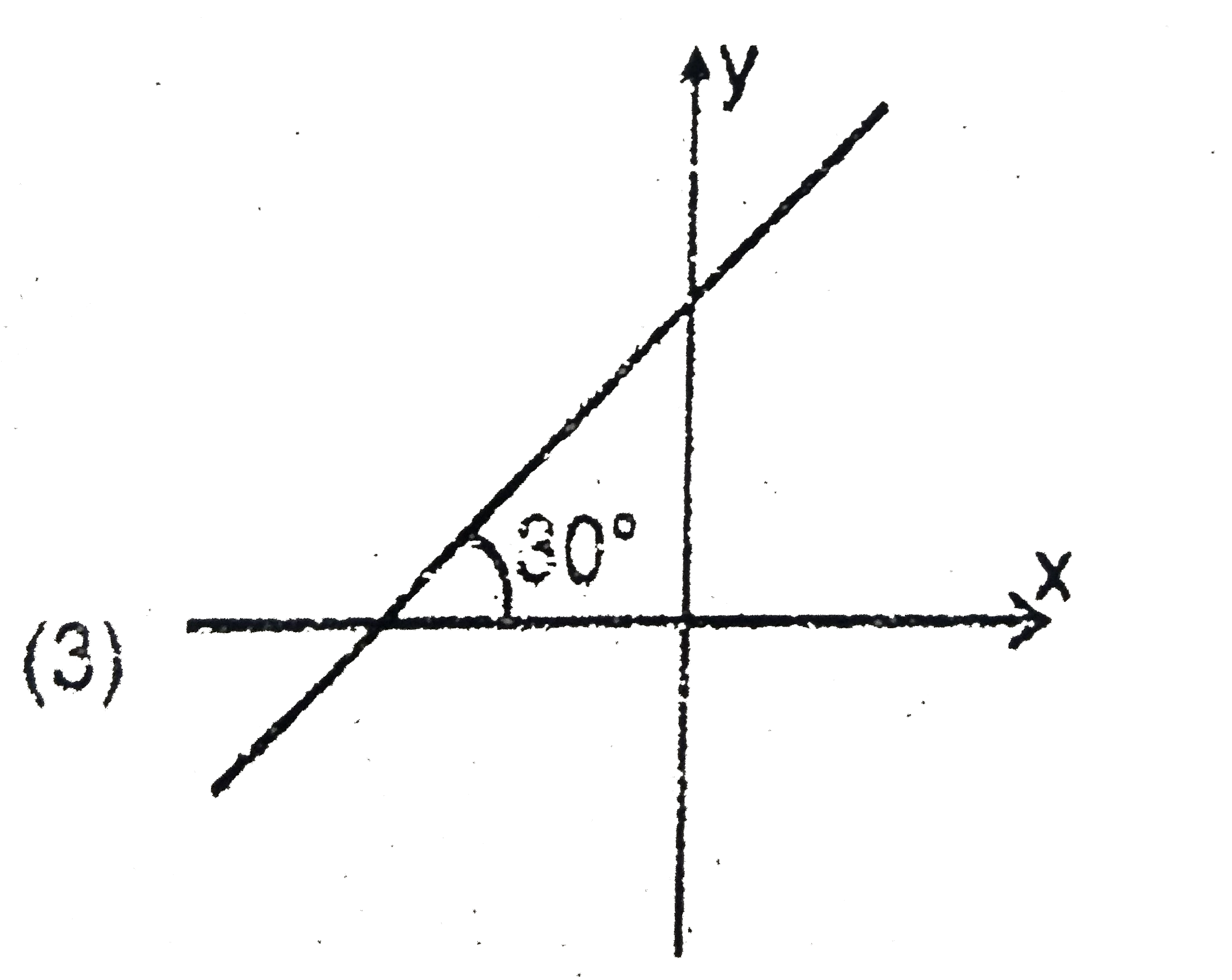 D
D
Area bounded between the curves y=√4−x2 and y2=3|x| in square units is
View SolutionThe area bounded by the curves x2+y2=4, |y|=√3x and y - axis, is
View SolutionThe area included between the parabolas y2=4ax(x+a)andy2=4b(b−x)is83√ab(a+b).True False
View Solution2√x+3√y=2 and 4√x−9√y=−1
View SolutionThe shortest distance between line y-x=1 and curve x=y2 is : (1) √34 (2) 3√28 (3) 83√2 (4) 4√3
View SolutionThe area bounded by the curve x2+y2=36and√|x|+√|y|=√6 is :
View Solution