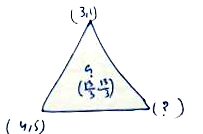
Co-ordinate of A is at 5 unit and if co-ordinate of B is -6 unit then find distance between AB.
Step by step video & image solution for Co-ordinate of A is at 5 unit and if co-ordinate of B is -6 unit then find distance between AB. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COVERAGE STANDARD QUESTION BOOK - UNIQUE PUBLICATIONCHAPTER - COVERAGE STANDARD QUESTION EXERCISE - 2 Mark Questions
73 Videos
- CO-ORDINATE GEOMETRYBOOK - UNIQUE PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Assignment-V
8 Videos
- FINANCIAL PLANNINGBOOK - UNIQUE PUBLICATIONCHAPTER - FINANCIAL PLANNINGEXERCISE - UNIQUE PRACTICE SESSION (4 MARK QUESTION )
1 Videos
Similar Questions
Knowledge Check
The co-ordination number of a metal in co-ordination compound is –
ASame as primary valencyBSum of primary and secondary valenciesCSame as secondary valencyDNone of the aboveThe co-ordinates of one end-point of a circle are (-3, 1) and the co-ordinates of the centre of the circle are (2, 4). The co-ordinates of the other end-point of the diameter are
A(−12,−32)B(−7,9)C(7,9)D(12,32)
In which quadrant are the following points. Whose x-co-ordinate is positive and y co- ordinate is negative. Both co-ordinates are negative.
View SolutionThe co-ordinate o point b on the numberline is -3 . Find the co-ordinates of the points which are at adistance of 6 units from B.
View SolutionFind the co-ordinates of the point at at a distance 6 units from the point (1,1) in the direction making an angle of 60∘ with the positive direction of the x− axis.
View SolutionFind the co-ordinates of the point at a distance of √14 from the mid-point of AB on the line joining the point A(1,2,3) and B(3,6,9).
View SolutionA straight line has slope 34 and it passes through the point A(−2,−5). Find the co-ordinates of a point B on this line where ¯¯¯¯¯¯AB=10 unit.
View SolutionFind the co-ordinates of the point at a distance of √5units from the point (1,2,3) on the line x+23=y+12=z−32.
View Solution