Assertion: Vector product of two vectors is an axial vector.
Reason: If →v= instantaneous Velocity, →r= radius vector and →ω= angular velocity, then →ω=→v×→r.
If both assertion and reason are true and reason is the correct explanation of assertion.
If both assertion and reason are true but reason is not the correct explanation of assertion
If assertion is true but reason is false.
If both aseertion and reason are false.
The correct Answer is:C
→v=→ω×→r
The expression →ω=→v×→r is wrong.
Topper's Solved these Questions
- VECTORSBook:A2ZChapter:VECTORSExercise:NEET Questions
Explore 12 Videos
- VECTORSBook:A2ZChapter:VECTORSExercise:Chapter Test
Explore 29 Videos
- VECTORSBook:A2ZChapter:VECTORSExercise:Problems Based On Mixed Concepts
Explore 32 Videos
- UNIT, DIMENSION AND ERROR ANALYSISBook:A2ZChapter:UNIT, DIMENSION AND ERROR ANALYSISExercise:Chapter Test
Explore 28 Videos
- WAVES AND ACOUSTICSBook:A2ZChapter:WAVES AND ACOUSTICSExercise:Chapter Test
Explore 30 Videos
Similar Questions
If →a is a non-zero vector and →a.→b=→a.→c,→a×→b=→a×→c, then
View SolutionThe linear velocity of a rotating body is given by →v=→ω×→r, where →ω is the angular velocity and →r is the radius vector. The angular velocity of a body is →ω=ˆi−2ˆj+2ˆk and the radius vector →r=4ˆj−3ˆk, then |→v| is
View Solution
Knowledge Check
The cross product of two vectors gives a vector perpendicular to the plane containing the vectors.
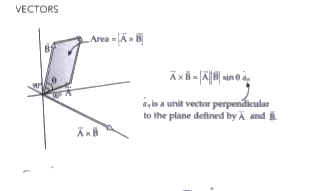
If →a and →b are non-zero vectors, then →a⋅(→a×→b)= _________A0B→0C→aD→bThe vector product of two vectors →Aand→B is zero. The scalar product of →Aand(→A+→B) will be
AzeroBA2CABDA2+ABThe linear velocity of a rotating body is given by →v=→ω×→r, where →ω is the angular velocity and →r is the radius vector. The angular velocity of a body is →ω=ˆi−2ˆj+2ˆk and the radius vector →r=4ˆj−3ˆk, then |→v| is-
A√29 unitsB√31 unitsC√37 unitsD√41 units
In nonuniform circular motion, the linear acceleration →a, the angular acceleration, →α, the angular velocity →v the radius vector →r and the angular velocity →ω at any instant are related by the vector equation,
View SolutionThe cross product of two vectors gives a vector perpendicular to the plane containing the vectors.
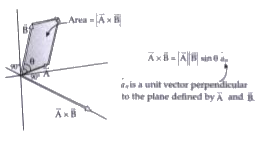
The angle between →a×→b and →b×→a is ___________.
View SolutionIf →u and →v are unit vectors and θ is the actue angle between them, then 2→u×3→v is a unit vector for
View SolutionThe resultant of two vectors →P and →Q is →R. If →Q is doubled then the new resultant vector is perpendicular to →P. Then magnitude of →R is :-
View SolutionIf for two vectors →A and →B,→A×→B=0, the vectors
View Solution