A body is allowed to fall from a height of 100m. If the time taken for the first 50m is t1 and for the remaining 50m is t2 then :
t1=t2
t1>t2
t1<t2
depends upon the mass.
The correct Answer is:B
To solve the problem, we need to find the relationship between the time taken to fall the first 50 meters (T1) and the time taken to fall the next 50 meters (T2) when a body falls from a height of 100 meters.
1. Understanding the Problem:
- The body falls from a height of 100 meters.
- The distance is divided into two parts: the first 50 meters and the second 50 meters.
- We need to find the relationship between T1 (time for the first 50 m) and T2 (time for the second 50 m).
2. Using the Equation of Motion:
- For free fall, the equation of motion is given by:
S=ut+12gt2
- Here, S is the distance fallen, u is the initial velocity (which is 0 for free fall), g is the acceleration due to gravity, and t is the time taken.
3. Calculating T1:
- For the first 50 meters:
50=0⋅T1+12gT12
- Simplifying this gives:
50=12gT12⟹T12=100g⟹T1=√100g=10√g
4. Calculating Total Time for 100 Meters:
- For the total distance of 100 meters:
100=0⋅T+12gT2
- This simplifies to:
100=12gT2⟹T2=200g⟹T=√200g=10√2√g
5. Finding T2:
- Since T is the total time for the fall, we can express T2 as:
T2=T−T1
- Substituting the values we calculated:
T2=10√2√g−10√g=10(√2−1)√g
6. Finding the Relationship between T1 and T2:
- Now we have:
T1=10√gandT2=10(√2−1)√g
- To find the relationship, we can compare T1 and T2:
T2T1=10(√2−1)10=√2−1
- Since √2≈1.414, we find:
√2−1≈0.414<1
- Therefore, T2<T1.
Conclusion:
Thus, we conclude that T1>T2.
- The body falls from a height of 100 meters.
- The distance is divided into two parts: the first 50 meters and the second 50 meters.
- We need to find the relationship between T1 (time for the first 50 m) and T2 (time for the second 50 m).
- For free fall, the equation of motion is given by:
S=ut+12gt2
- Here, S is the distance fallen, u is the initial velocity (which is 0 for free fall), g is the acceleration due to gravity, and t is the time taken.
- For the first 50 meters:
50=0⋅T1+12gT12
- Simplifying this gives:
50=12gT12⟹T12=100g⟹T1=√100g=10√g
- For the total distance of 100 meters:
100=0⋅T+12gT2
- This simplifies to:
100=12gT2⟹T2=200g⟹T=√200g=10√2√g
- Since T is the total time for the fall, we can express T2 as:
T2=T−T1
- Substituting the values we calculated:
T2=10√2√g−10√g=10(√2−1)√g
- Now we have:
T1=10√gandT2=10(√2−1)√g
- To find the relationship, we can compare T1 and T2:
T2T1=10(√2−1)10=√2−1
- Since √2≈1.414, we find:
√2−1≈0.414<1
- Therefore, T2<T1.
Thus, we conclude that T1>T2.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Relative Motion In One Dimension20videos
GENERAL KINEMATICS AND MOTION IN ONE DIMENSION
- BOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Understanding Motion Through Graphs40videos
GENERAL KINEMATICS AND MOTION IN ONE DIMENSION
- BOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Motion With Constant Acceleration30videos
GENERAL KINEMATICS AND MOTION IN ONE DIMENSION
- BOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Chapter Test29videos
FLUID MECHANICS
- BOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Chapter Test29videos
GRAVITATION
Similar Questions
[" I."A" body is allowed to fall from a "],[" height of "100m" .If the time taken for "],[" the first "50m" is "t_(1)" and for the "],[" remaining "50m" is "^(t_(2))" ."],[" The ratio of "t_(1)" and "t_(2)" is nearly "],[qquad [5:2],[3:1],[3:2]]
View SolutionA body is dropped from a height of 100m .Ratio of times taken to travel first 10m ,next 10m is
View Solution
Knowledge Check
- Question 1 - Select One
A body is allowed to fall from a height of 10m. If the time taken for the first 50m is t1 and for the remaining 50s,is t2.
The ratio t1 and t2. Is nearly .A5:2B3:1C3:2D5:3 - Question 2 - Select One
A body is allowed to fall from a height of 10m. If the time taken for the first 50m is t1 and for the remaining 50s,is t2.
The ratio of time to reach the ground and to reach first half of the distance is .A√3:1B√2:1C5:2D1:√3 - Question 3 - Select One
A body is allowed to fall from a height of 10m. If the time taken for the first 50m is t1 and for the remaining 50s,is t2.
Which is correct?At1=t2Bt1≤t2Ct1<t2Dt1.t2
A body falling from a height of 10m rebounds from the hard floor . It
View SolutionA body is falling from a height of 200 m. after it has fallen 100 m, the body will possess :
View SolutionA body has a free fall from a height of 20 m. After falling through a distance of 5 m, the body would
View SolutionA black body of temperature T is inside chamber of T0 temperature initially. Sun rays are allowed to fall from a hole in the top of chamber. If the temperature of black body (T) and chamber (T0) remains constant, then
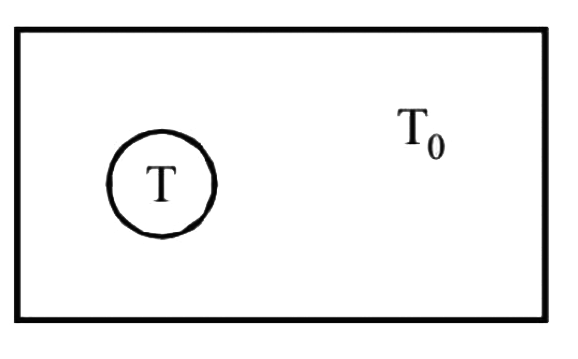 View Solution
View SolutionA body is falling from height 'h' it takes t1 time to rech the ground. The time taken to cover the first half of height is:-
View Solution

