In a rectangle ABCD,AB=21 and BC=1. Axes × and yy pass through centre of the rectangle. The moment of inertia is least about :
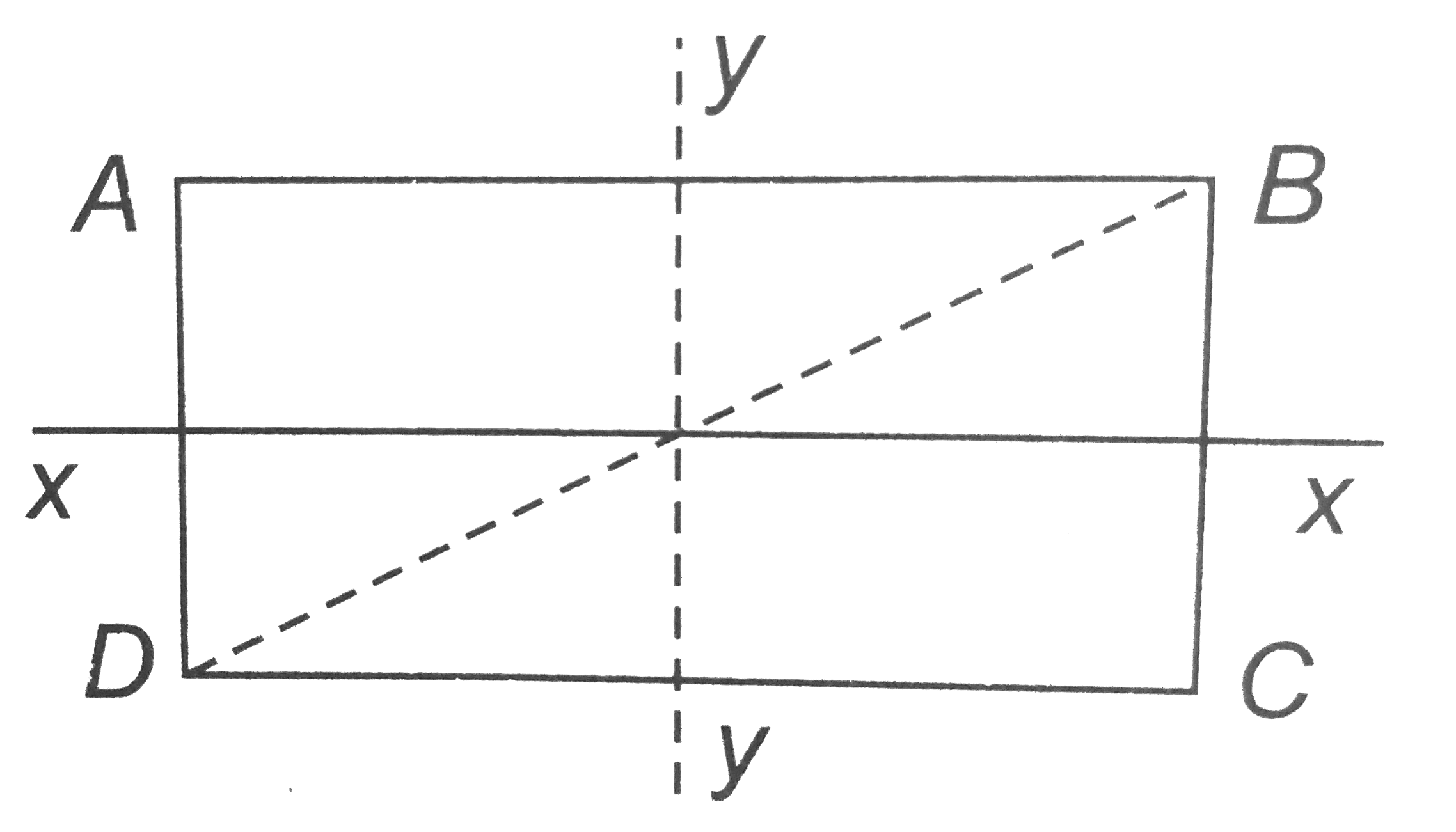 .
.
DB
BC
xx
yy
The correct Answer is:A
(a) Because distribution of mass is closest around the axis BD in this case.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Rotational Kinematics19videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Torque , Torque Equation And Equilibrium Of A Rigid Body29videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Displacement , Velocity And Acceleration Of Centre Of Mass30videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Chapter Test30videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
In a rectangle ABCD, AB= 15 cm ∠BAC=60∘ then BC is equal to
A15√3B15√32C15√3D152√3 - Question 2 - Select One
In a rectangle ABCD , AB = 15 cm and ∠BAC=60∘, then the length of side BC is
A15√2 cmB15 cmC15√5 cmD15√3 cm - Question 3 - Select One
In a rectangle ABCD(BC=2AB). The moment of inertia is maximum along axis through
 ABCBABCHFDEG
ABCBABCHFDEG
If I moment of inertia of a thin circular plate about an axis passing through tangent of plate in its plane. The moment of inertia of same circular plate about an axis perpendicular to its plane and passing through its centre is
View SolutionIn a rectangle ABCD(BC=2AB). The moment of inertia is minimum along axis through
 View Solution
View SolutionLet I be the moment of interia of a uniform square plate about an axis AB that passses through its centre and is parallel to two its sides. CD is a line in the plane of the plate that passes through the centre of the plate and makes an angle θ with AB. The moment of inertia of the plate about the axis CD is then equal to-
View SolutionLet I be the moment of interia of a uniform square plate about an axis AB that passses through its centre and is parallel to two its sides. CD is a line in the plane of the plate that passes through the centre of the plate and makes an angle θ with AB. The moment of inertia of the plate about the axis CD is then equal to-
View SolutionABCD is a rectangle with A(−1,2),B(3,7) and AB:BC=4:3. If P is the centre of the rectangle, then the distance of P from each corner is equal to
View Solution

