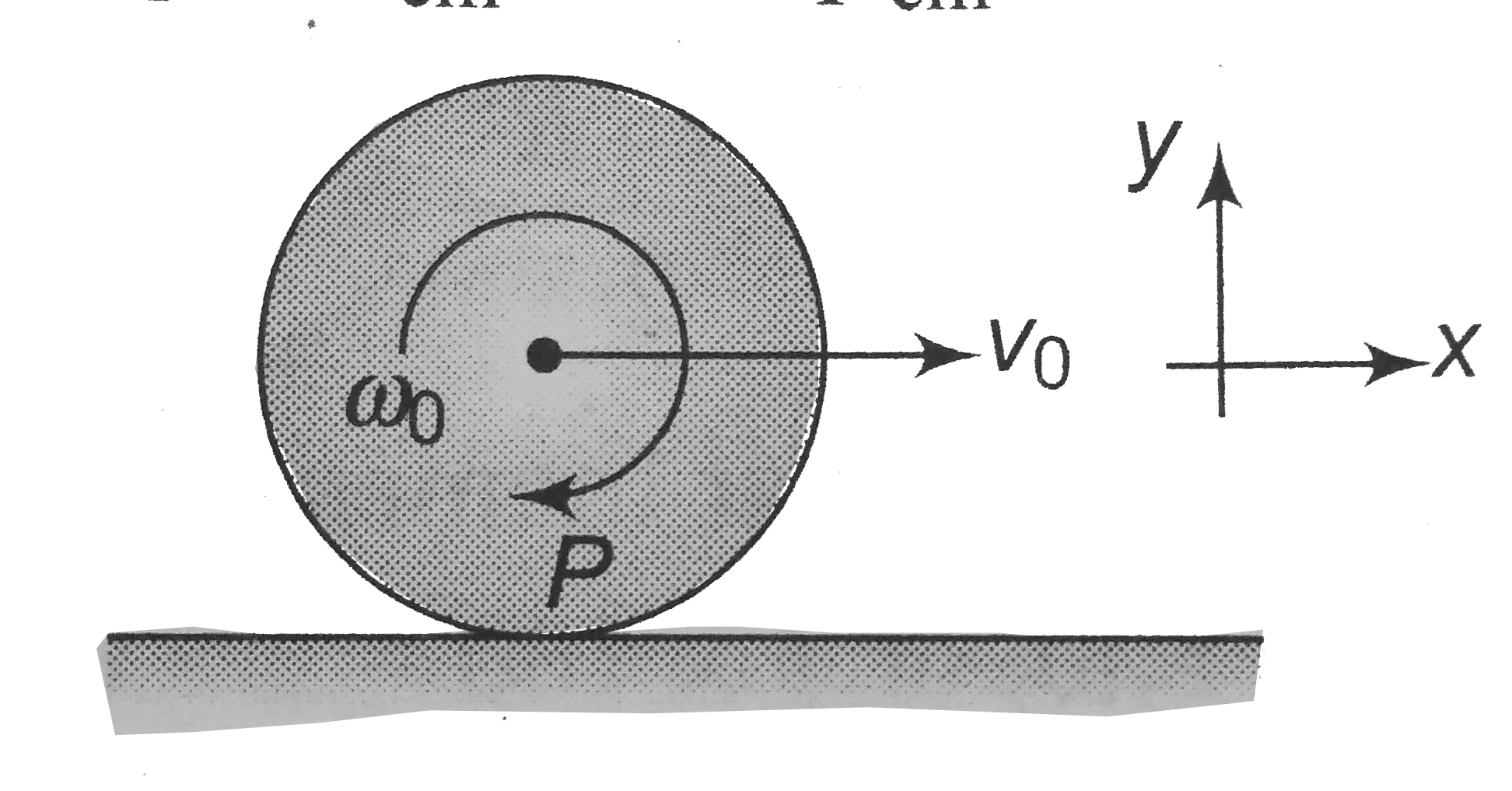
A sphere of mass $M$ rolls without slipping on rough surface with centre of mass has constant speed $v_{0}$ . If mass of the sphere is $m$ and its radius be R, then the angular momentum of the sphere about the point of contact is.

A

$\frac{4}{5} M v_{0} R (- ˆ k)$

B

$\frac{9}{5} M v_{0} R (- ˆ k)$

C

$\frac{8}{5} M v_{0} R (- ˆ k)$

D

$\frac{7}{5} M v_{0} R (- ˆ k)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

(d) Since ${\to L}_{p} = {\to L}_{c m} + \to r \times {\to p}_{c m}$
${\to L}_{p} = v = (10 - t) m s^{- 1}$
= $I_{c m} ω_{0} (- ˆ k) + M v_{0} R (- ˆ k)$
Since sphere is in pure rolling motion hence
$ω = v_{0} / R$
$\Rightarrow {\to L}_{p} = [\frac{2}{5} M R^{2} (\frac{v_{0}}{R}) + M v_{0} R (- ˆ k)] = \frac{7}{5} M v_{0} R (- ˆ k)$ .
.

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A solid sphere of mass m rolls without slipping on an inclined plane of inclination $θ$ . The linear acceleration of the sphere is
A $\frac{7}{5} g sin θ$
B $\frac{2}{7} g sin θ$
C $\frac{3}{7} g sin θ$
D $\frac{5}{7} g sin θ$
Question 2 - Select One
A solid sphere of mass $m$ and radius $R$ rolls without slipping on a horizontal surface such that $v_{c . m .} = v_{0}$ .
AThe kinetic energy of rotation is $\frac{1}{5} m v_{0}^{2}$ .
BThe total kinetic energy is $\frac{7}{10} m v_{0}^{2}$ .
CThe mechanical energy (assume the ground as reference) is $m g R + \frac{7}{10} m v_{0}^{2}$ .
DAll options are correct.
Question 3 - Select One

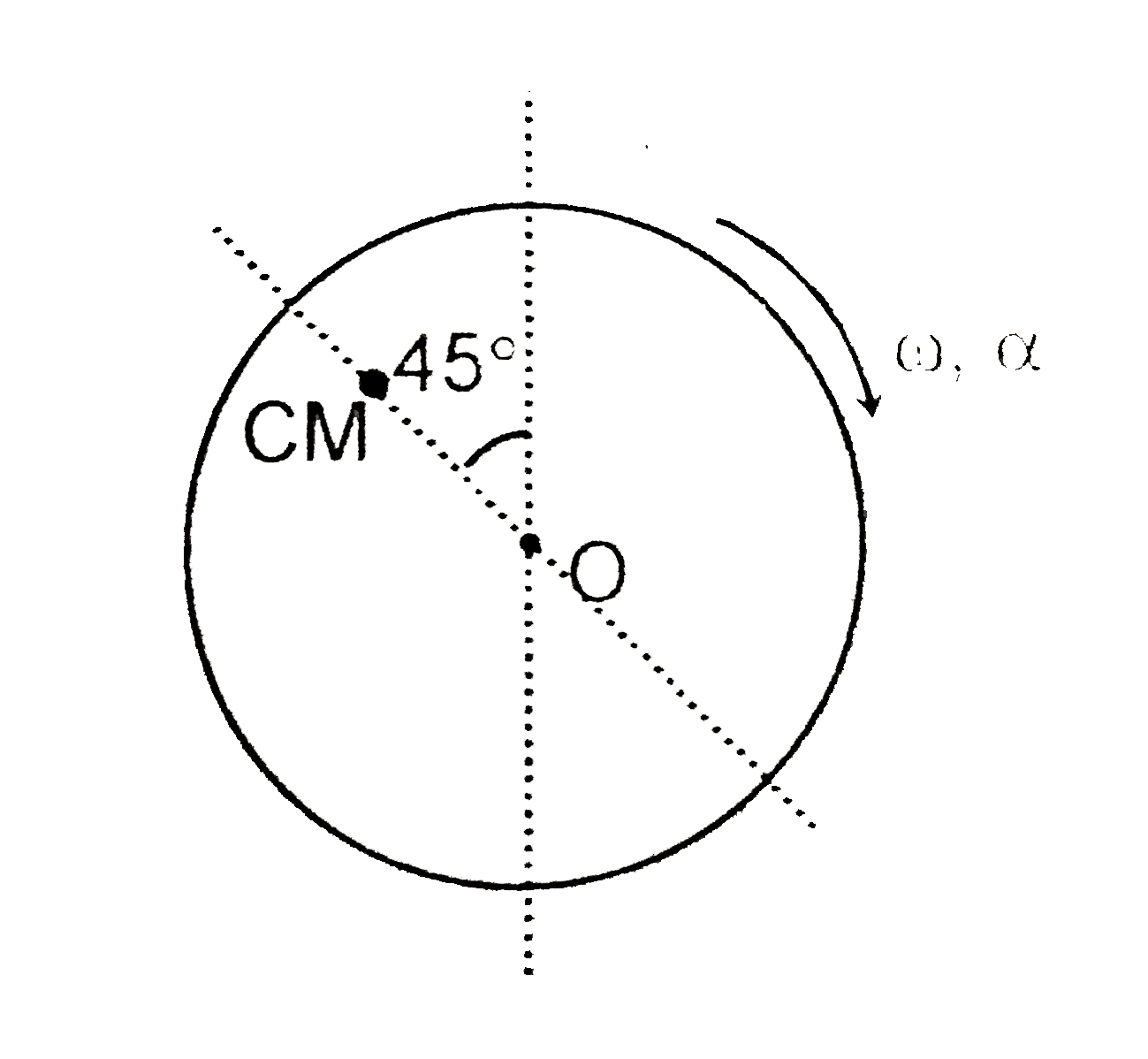
A solid sphere of mass m and radius R is rolling without slipping on a rough horizontal surface with angular acceleration $α$ . Centre of mass of sphere lies at a distance $\frac{R}{\sqrt{2}}$ from the centre of sphere. Find the normal force applied by sphere on the surface at an instant when line joining centre of mass of sphere and centre of sphere makes an angle $45^{\circ}$ with the vertical and angular velocity of sphere at this instant is $ω$ .
A $m g + \frac{m R}{2} (α - ω^{2})$
B $m g - \frac{m R}{2} (α + ω^{2})$
C $m g - \frac{m R}{2} (α - ω^{2})$
D $m g + \frac{m R}{2} (α + ω^{2})$

A2Z-ROTATIONAL DYNAMICS-Problems Based On Mixed Concepts

Home
Ask Doubt
Profile