Assertion : Velocity of particles, while crossing mean position (in stationary waves) varies from maximum at antinodes to zero at nodes.
If both assertion and reason are true and reason is the correct expleanation of assertion.
If both assertion and reason are true and reason is not the correct expleanation of assertion.
If assertion is true but reason is false.
If assertion and reason both are false.
The correct Answer is:A
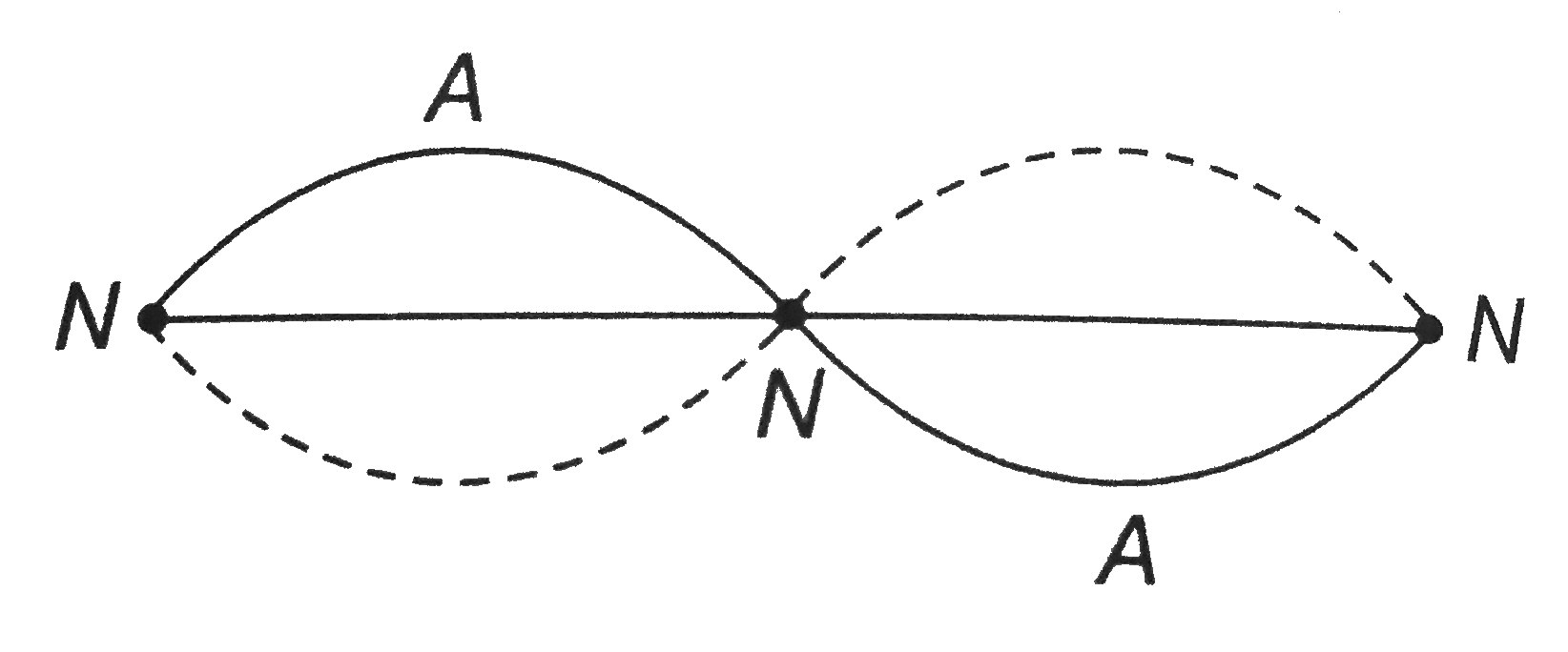
Stationary wave A node is a place of zero amplitude and an antinode is a place of maximum amplitude.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - NEET Questions46videos
WAVES AND ACOUSTICS
- BOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Chapter Test30videos
WAVES AND ACOUSTICS
- BOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Problems Based On Mixed Concepts15videos
WAVES AND ACOUSTICS
- BOOK - A2ZCHAPTER - VECTORSEXERCISE - Chapter Test29videos
VECTORS
- BOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Chapter Test29videos
WORK, ENERGY, POWER AND COLLISION
Similar Questions
Knowledge Check
- Question 1 - Select One
Velocity of a particle in stationary wave is maximum at
AAntinodesBNodeCMid way between node and antinodeDCan not be predicted - Question 2 - Select One
Particle velocity at the node point in a stationary waves is
AMaximumBZeroCMinimumDAverage velocity - Question 3 - Select One
The velocity of a particle in simple harmonic motion at displacement y from mean position is
Aω√(a2+y2)Bω√(a2−y2)CwyDω2√(a2+y2)
In a stationary wave, the particle velocity at the nodal points is
View SolutionIn a string wave , all particles of the medium cross the mean position with
View SolutionThe velocity of a particle in simple harmonic motion at displacement y from mean position is
View SolutionIn a stationary wave, all particles of the medium cross the mean position with (
View SolutionThe distance between any two successive nodes or antinodes in stationary waves is
View Solution

