The maximum range of a gun from horizontal terms is 16km If g=10m/s2 what must be the muzele velocity of the sheet?
400m/s
200m/s
100m/s
50m/s
The correct Answer is:a
To solve the problem, we need to find the muzzle velocity of a gun given the maximum range and the acceleration due to gravity.
1. Understand the Range Formula:
The formula for the maximum range R of a projectile launched at an angle θ is given by:
R=v2sin(2θ)g
where v is the muzzle velocity, g is the acceleration due to gravity, and θ is the angle of projection.
2. Determine the Angle for Maximum Range:
The maximum range occurs at an angle of 45∘. Therefore, we can simplify the sine term:
sin(2θ)=sin(90∘)=1
Thus, the range formula simplifies to:
R=v2g
3. Substitute the Given Values:
We know the maximum range R=16km=16,000m and g=10m/s2. Substituting these values into the formula gives:
16000=v210
4. Rearranging the Equation:
To find v2, we rearrange the equation:
v2=16000×10
v2=160000
5. Calculating the Muzzle Velocity:
Now, take the square root of both sides to find v:
v=√160000=400m/s
6. Conclusion:
The muzzle velocity of the gun is 400m/s.
Final Answer:
The muzzle velocity of the gun must be 400m/s.
The formula for the maximum range R of a projectile launched at an angle θ is given by:
R=v2sin(2θ)g
where v is the muzzle velocity, g is the acceleration due to gravity, and θ is the angle of projection.
The maximum range occurs at an angle of 45∘. Therefore, we can simplify the sine term:
sin(2θ)=sin(90∘)=1
Thus, the range formula simplifies to:
R=v2g
We know the maximum range R=16km=16,000m and g=10m/s2. Substituting these values into the formula gives:
16000=v210
To find v2, we rearrange the equation:
v2=16000×10
v2=160000
Now, take the square root of both sides to find v:
v=√160000=400m/s
The muzzle velocity of the gun is 400m/s.
The muzzle velocity of the gun must be 400m/s.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - MOCK TESTEXERCISE - General Kinematics30videos
MOCK TEST
- BOOK - A2ZCHAPTER - MOCK TESTEXERCISE - Motion With Constant Acceleration15videos
MOCK TEST
- BOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test29videos
KINETIC THEORY OF GASES AND THERMODYNAMICS
- BOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test29videos
MOTION IN TWO DIMENSION
Similar Questions
The maximum range of a bullet fired from a toy pistol mounted on a car at rest is R0=40m. What will be the acute angle of inclination of the pistol for maximum range when the car is moving in the direction of firing with uniform velocity V=20m/s, on a horizontal surface? (g=10m/s2)
View SolutionThe maximum range of a gun of horizontal terrain is 16 km. If g = 10 mis, then the muzzle velocity of a shell must be
View Solution
Knowledge Check
- Question 1 - Select One
The maximum range of gun on horizontal terrain is 15 km. If g = 10 m/s2. What
must be the muzzle velocity of the shell ?A387.2 m//sB390 m//sC380 m//sD350 m//s - Question 2 - Select One
The maximum range of a gun on horizontal terrain is 1km. If g=10ms−2, what must be the muzzle velocity of the shell ?
A400ms−1B200ms−1C100ms−1D50ms−1 - Question 3 - Select One
The maximum range of a gun of horizontal terrain is 16 km. If g = 10 mis, then the muzzle velocity of a shell must be
A200√2m/sB160m/sC800m/sD400m/s
The maximum range of a gun on horizontal terrain is 25 km. If g= 10 ms−2, the muzzle velocity of the shell
View SolutionThe velocity of kerosene oil in a horizontal pipe is 5m/s. If g=10m/s2 then the velocity head of oil wlill be
View SolutionA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
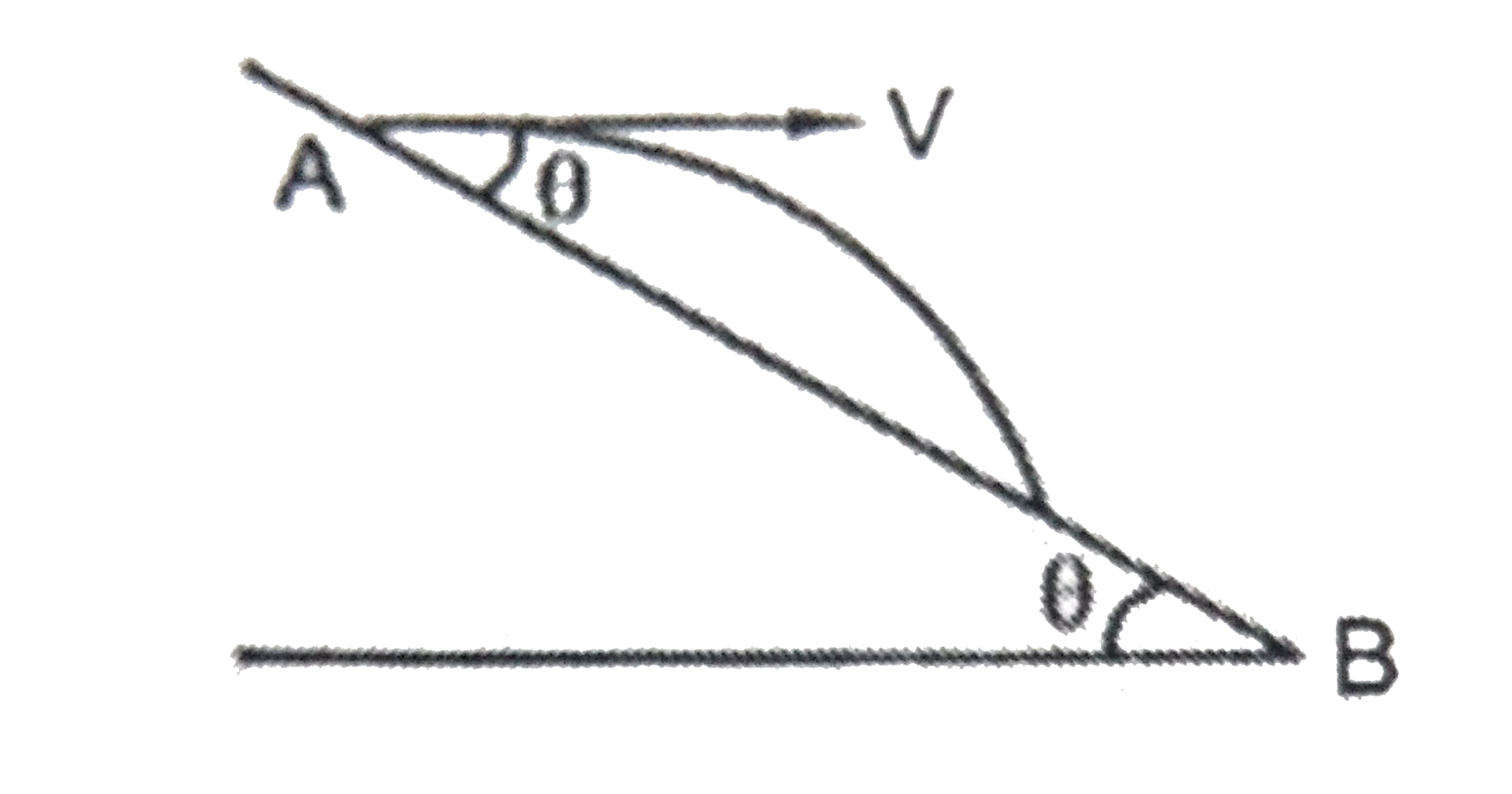
What is the velocity of the particle just before it hits the plane?View SolutionA missile is fired for maximum range with an initial velocity of 20m/s. If g=10m/s2, the range of the missile is
View SolutionA bullet of mass 10 g is fired from a gun of mass 6 kg with a velocity of 300 m/s. Calculate the recoil velocity of the gun.
 View Solution
View Solution

