An asteroid of mass $m$ is approaching earth, initially at a distance $10 R_{E}$ with speed $v_{i}$ . It hits earth with a speed $v_{f}$ ( $R_{E}$ and $M_{E}$ are radius and mass of earth),. Then

A

$v_{f}^{2} = v_{i}^{2} + \frac{2 G m}{M_{e} R_{e}} (1 - \frac{1}{10})$

B

$v_{f}^{2} = v_{i}^{2} + \frac{2 G m_{e}}{R_{e}} (1 + \frac{1}{10})$

C

$v_{f}^{2} = v_{i}^{2} + \frac{2 G m_{e}}{R_{e}} (1 - \frac{1}{10})$

D

$v_{f}^{2} = v_{i}^{2} + \frac{2 G m}{R_{e}} (1 - \frac{1}{10})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Applying law of conservation of energy for asteroid at a distance $10 R_{e}$ and at earth's surface,

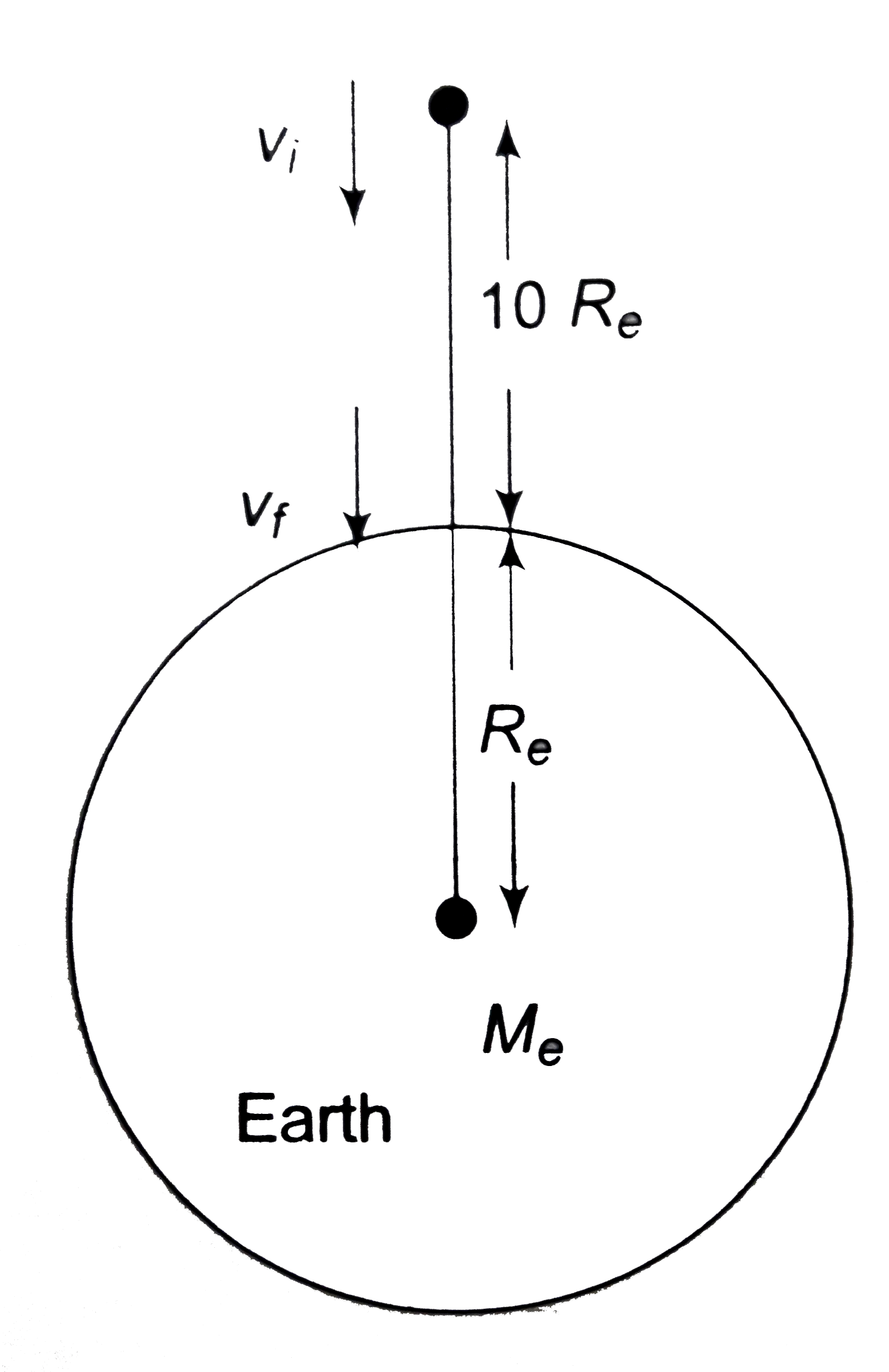
$K_{i} + U_{i} = K_{f} + U_{f}$ ....(i)
Now, $K_{i} = \frac{1}{2} m v_{i}^{2}$ and $U_{i} = \frac{G M_{e} m}{10 R_{e}}$
$K_{f} = \frac{1}{2} m v_{f}^{2}$ and $U_{f} = - \frac{G M_{e} m}{R_{e}}$
Substituting these values in Eq.(i),we get
$\frac{1}{2} m v_{i}^{2} - \frac{G M_{e} m}{10 R_{e}} = \frac{1}{2} m v_{f}^{2} - \frac{G M_{e} m}{R_{e}}$
$\Rightarrow \frac{1}{2} m v_{f}^{2} = \frac{1}{2} m v_{i}^{2} + \frac{G M_{e} m}{R_{e}} - \frac{G M_{e} m}{10 R_{e}}$
$\Rightarrow v_{f}^{2} = v_{i}^{2} + \frac{2 G M_{e}}{R_{e}} - \frac{2 G M_{e}}{10 R_{e}}$
$∴ v_{f}^{2} = v_{i}^{2} + \frac{2 G M_{e}}{R_{e}} (1 - \frac{1}{10})$

|

Updated on:21/07/2023

Class 11 PHYSICS MOCK TEST

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
An asteroid of mass $m$ is approaching earth, initially at a distance $10 R_{E}$ with speed $v_{i}$ . It hits earth with a speed $v_{f}$ ( $R_{E}$ and $M_{E}$ are radius and mass of earth),. Then
A $v_{f}^{2} = v_{i}^{2} + \frac{2 G M}{R_{e}} (1 + \frac{1}{10})$
B $v_{f}^{2} = v_{i}^{2} + \frac{2 G M_{e}}{R_{e}} (1 + \frac{1}{10})$
C $v_{f}^{2} = v_{i}^{2} + \frac{2 G M_{e}}{R_{e}} (1 - \frac{1}{10})$
D $v_{f}^{2} = v_{i}^{2} + \frac{2 G M}{R_{e}} (1 - \frac{1}{10})$
Question 2 - Select One
A satellite of mass m goes round the earth along a circular path of radius r. Let $m_{E}$ be the mass of the earth and $R_{E}$ its radius then the linear speed of the satellite depends on.
A $m, m_{E}$ and $r$
B $m, R_{E}$ and $r$
C $m_{E}$ only
D $m_{E}$ and $r$
Question 3 - Select One
A particle of mass m is projected from the surface of earth with a speed $V_{0} (V_{0} <$ escape velocity). Find the speed of particle at height $h = R$ (radius of earth). (Take, $R = 6400 k m$ and $g = 9.8 m / s^{2})$
A $\sqrt{g R}$
B $\sqrt{v_{0}^{2} - 2 g R}$
C $\sqrt{v_{0}^{2} - g R}$
DNone of these

A2Z-MOCK TEST-Motion With Constant Acceleration

Home
Ask Doubt
Profile