The temperature (T) dependence of resistivity (rho) of a semiconductor is represented by :
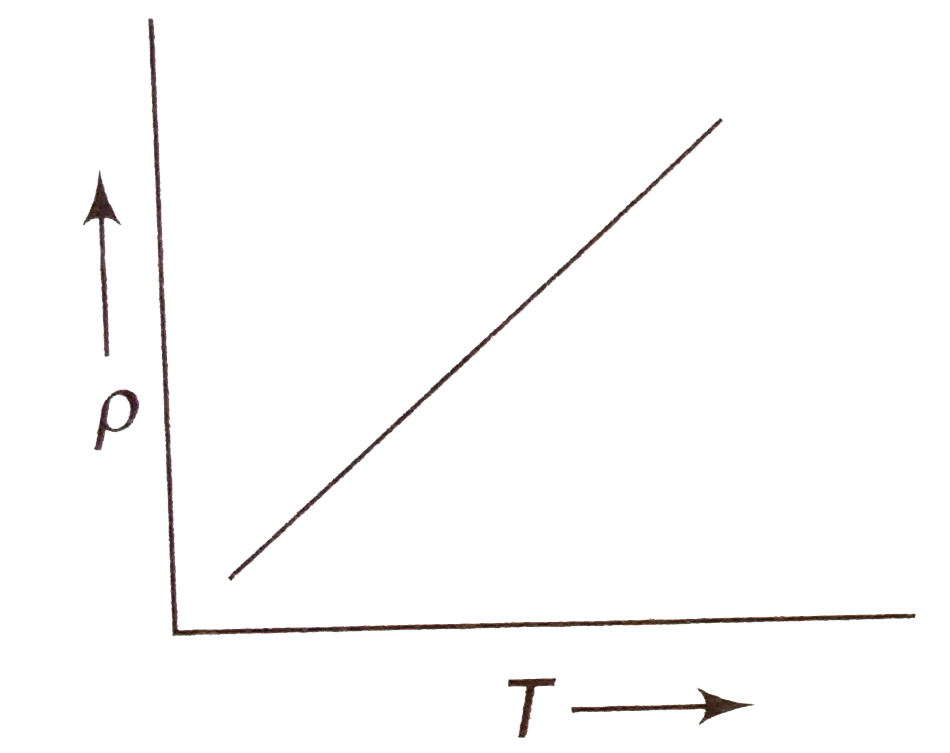
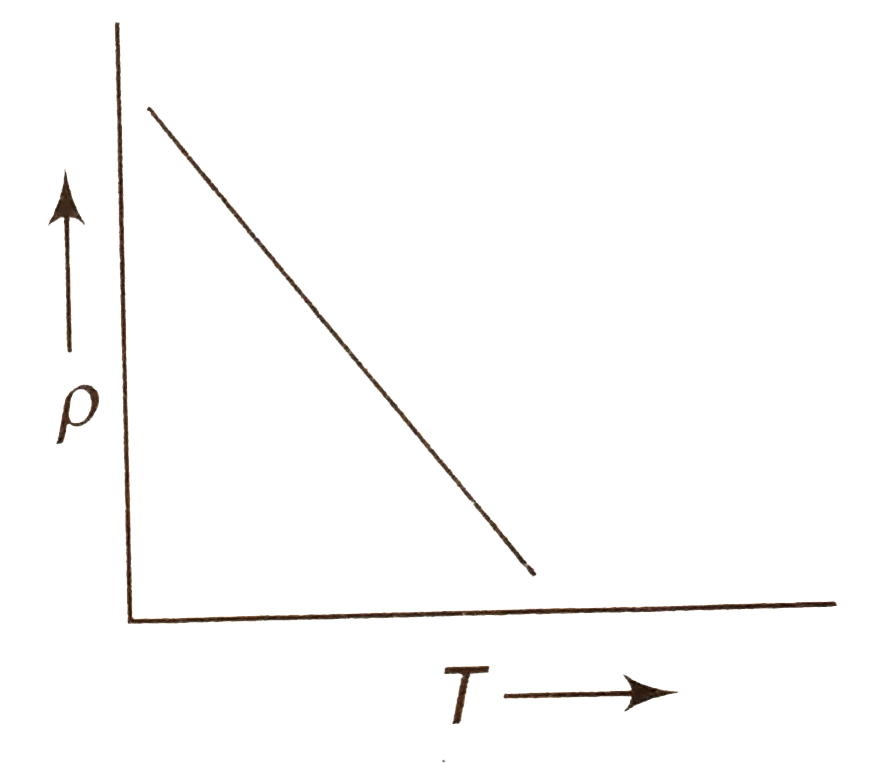
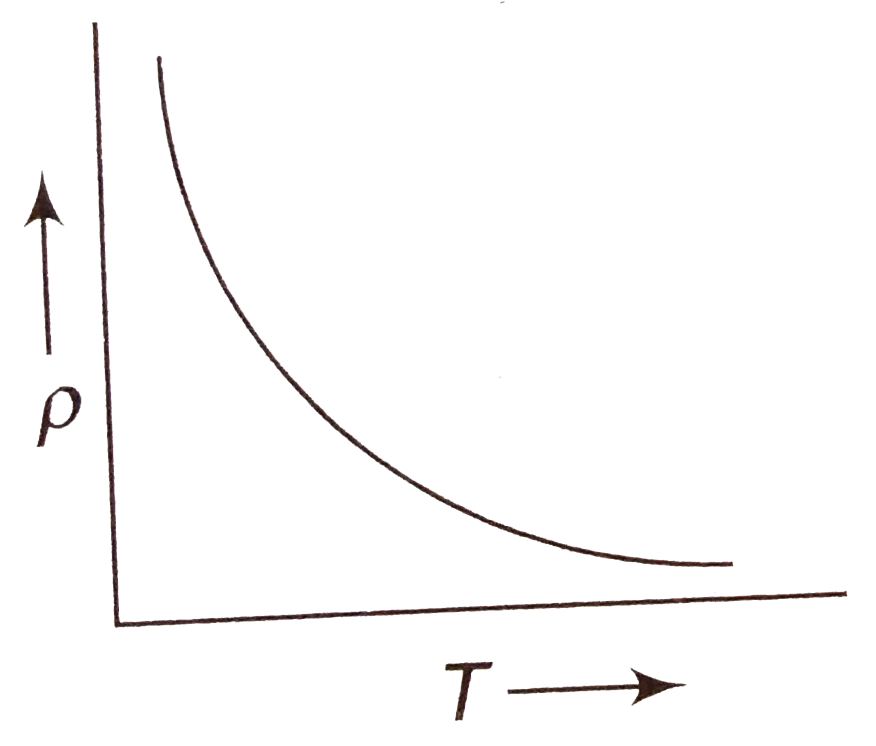
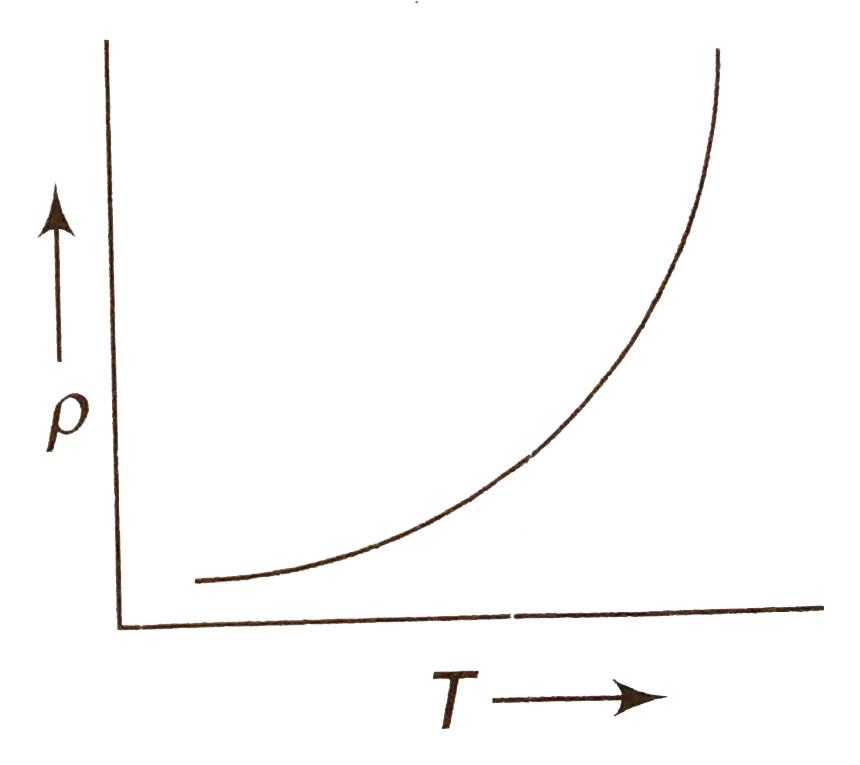
The correct Answer is:B
To solve the problem regarding the temperature dependence of resistivity (ρ) of a semiconductor, we can follow these steps:
1. Understand the Relationship Between Conductivity and Resistivity:
- The conductivity (σ) of a semiconductor increases with temperature. This is a key property of semiconductors.
- The resistivity (ρ) is inversely proportional to conductivity:
ρ=1σ
2. Establish the Temperature Dependence:
- As the temperature (T) increases, the conductivity (σ) increases. Therefore, the resistivity (ρ) must decrease.
- This can be expressed mathematically as:
σ∝T(as T increases, σ increases)
- Consequently, we can say:
ρ∝1T(as T increases, ρ decreases)
3. Formulate the Relationship:
- From the above relationship, we can derive that:
T⋅ρ=constant
- This implies that the product of temperature and resistivity remains constant for a semiconductor.
4. Graphical Representation:
- The relationship T⋅ρ=constant indicates that if you plot resistivity (ρ) on the y-axis and temperature (T) on the x-axis, the graph will be a rectangular hyperbola.
- This is because as one variable increases, the other decreases, maintaining a constant product.
5. Conclusion:
- Therefore, the correct representation of the temperature dependence of resistivity in a semiconductor is that it forms a rectangular hyperbola.
Final Answer:
The temperature dependence of resistivity (ρ) of a semiconductor is represented by a rectangular hyperbola.
---
- The conductivity (σ) of a semiconductor increases with temperature. This is a key property of semiconductors.
- The resistivity (ρ) is inversely proportional to conductivity:
ρ=1σ
- As the temperature (T) increases, the conductivity (σ) increases. Therefore, the resistivity (ρ) must decrease.
- This can be expressed mathematically as:
σ∝T(as T increases, σ increases)
- Consequently, we can say:
ρ∝1T(as T increases, ρ decreases)
- From the above relationship, we can derive that:
T⋅ρ=constant
- This implies that the product of temperature and resistivity remains constant for a semiconductor.
- The relationship T⋅ρ=constant indicates that if you plot resistivity (ρ) on the y-axis and temperature (T) on the x-axis, the graph will be a rectangular hyperbola.
- This is because as one variable increases, the other decreases, maintaining a constant product.
- Therefore, the correct representation of the temperature dependence of resistivity in a semiconductor is that it forms a rectangular hyperbola.
The temperature dependence of resistivity (ρ) of a semiconductor is represented by a rectangular hyperbola.
Topper's Solved these Questions
- MOCK TESTBOOK - A2ZCHAPTER - MOCK TESTEXERCISE - Motion With Constant Acceleration
15 Videos
- MOCK TESTBOOK - A2ZCHAPTER - MOCK TESTEXERCISE - Motion With Constant Acceleration
15 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test
29 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
Resistivity of a semiconductor depends on
AShape of semiconductorBAtomic nature of semiconductorCLength of semiconductorDShape and atomic nature of semiconductorAfter doping, resistivity of a semiconductor
Adoes not alterBincreasesCdecreasesDmay increase or decrease depending on the dopantResistivity of semiconductor depends on
Ashape of semiconductorBlength of semiconductorCatomic nature of semiconductorDsphape and atomic nature of semiconductor
Average and instantaneous current | Drift velocity | Mobility| Current density | Ohm's experimental law | Ohm's Law from drift velocity | Temperature dependence of resistivity for conductors | semi comductors and super conductors | Temperature dependence of resistance
View SolutionTemperature dependence of resistivity p(T) of semiconductors, insulators and metals is significantly based on the following factors:
View SolutionThe temperature coefficient of resistivity of a semiconductor is
View SolutionThe electrical resistivity of a semiconductor :
View SolutionWith the increase of temperature, the resistivity of a semiconductor
View Solution
