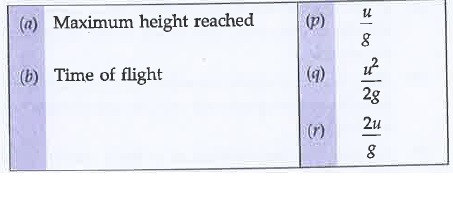
Two stones are thrown vertically upwards simultaneously with their initial velocities u1andu2 respectively. Prove that the heights reached by them would be in the ratio of u21:u22 (Assume upward acceleration is −g and downward acceleration to be +g).
At the highest point, v=0.
∴ From v2−u2=2gh
0−u2=2(−g)h , h=u22g
For two stones : h1=u212gandh2=u222g
∴h1h2=u21/2gu22/2g=u21u22
which was to be proved.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - MOTIONEXERCISE - very short answer queation25videos
MOTION
- BOOK - PRADEEPCHAPTER - MOTIONEXERCISE - Short answer questions15videos
MOTION
- BOOK - PRADEEPCHAPTER - MOTIONEXERCISE - NCERT short answer question7videos
MOTION
- BOOK - PRADEEPCHAPTER - GRAVITATIONEXERCISE - Model Test paper (1)36videos
GRAVITATION
- BOOK - PRADEEPCHAPTER - SOUNDEXERCISE - Model Test (sec - B)12videos
SOUND
Similar Questions
Knowledge Check
- Question 1 - Select One
Two bodies are thrown vertically upwards with their initial velocity in the ratio 2:3. Then the ratio of the maximum height attained by them is
A1:1B4:9C2:3D√2:√3 - Question 2 - Select One
A stone is thrown vertically upwards with an initial velocity u from the top of a tower of lieight 12u2g With what velocity does the stone reach the ground?
AuB4uC5uD2√6u - Question 3 - Select One
A body thrown vertically upwards with an initial valocity u reaches maximum height in 6 s. The ratio of the distances traveled by the body in the first second the seventh second is
A1:1B11:1C1:2D1:11
A particle is thrown vertically upward with a velocity of u. Find its time of flight.
View SolutionA stone is thrown vertically upwards with an initial speed u from the top of a tower, reaches the ground with a speed 3u. The height of the tower is :
View SolutionQuestion Stimulus
A stone is thrown vertically upwards with an initial velocity of 30ms−1. Acceleration due to gravity g=10ms−2. The maximum height reached by the stone isView SolutionTwo particles are thrown simultaneously from points A and B with velocities u1=2ms−1andu2−14ms−1, respectively, as shown in figure.
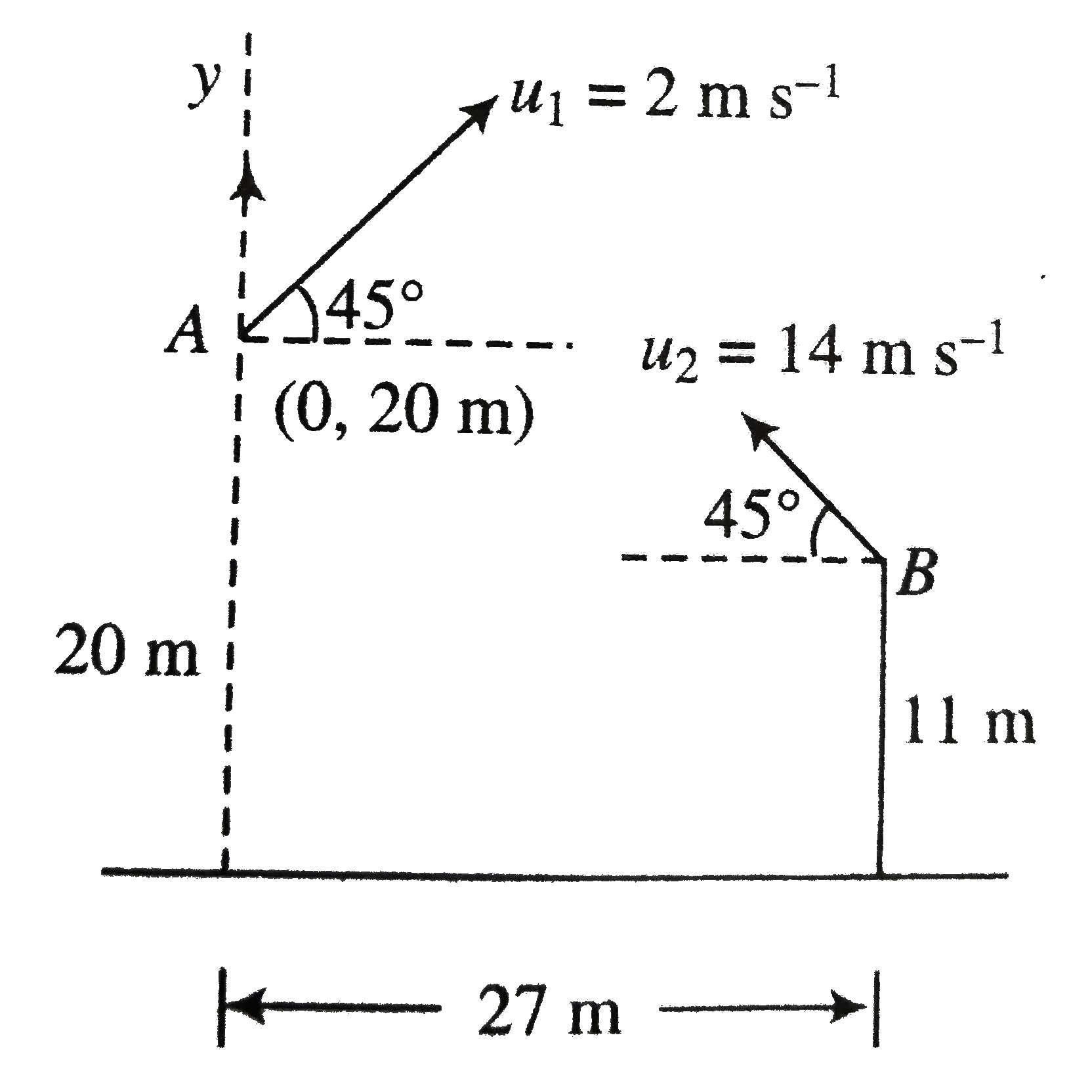
Minimum separation between A and B isView SolutionTwo particles are thrown simultaneously from points A and B with velocities u1=2ms−1andu2−14ms−1, respectively, as shown in figure.
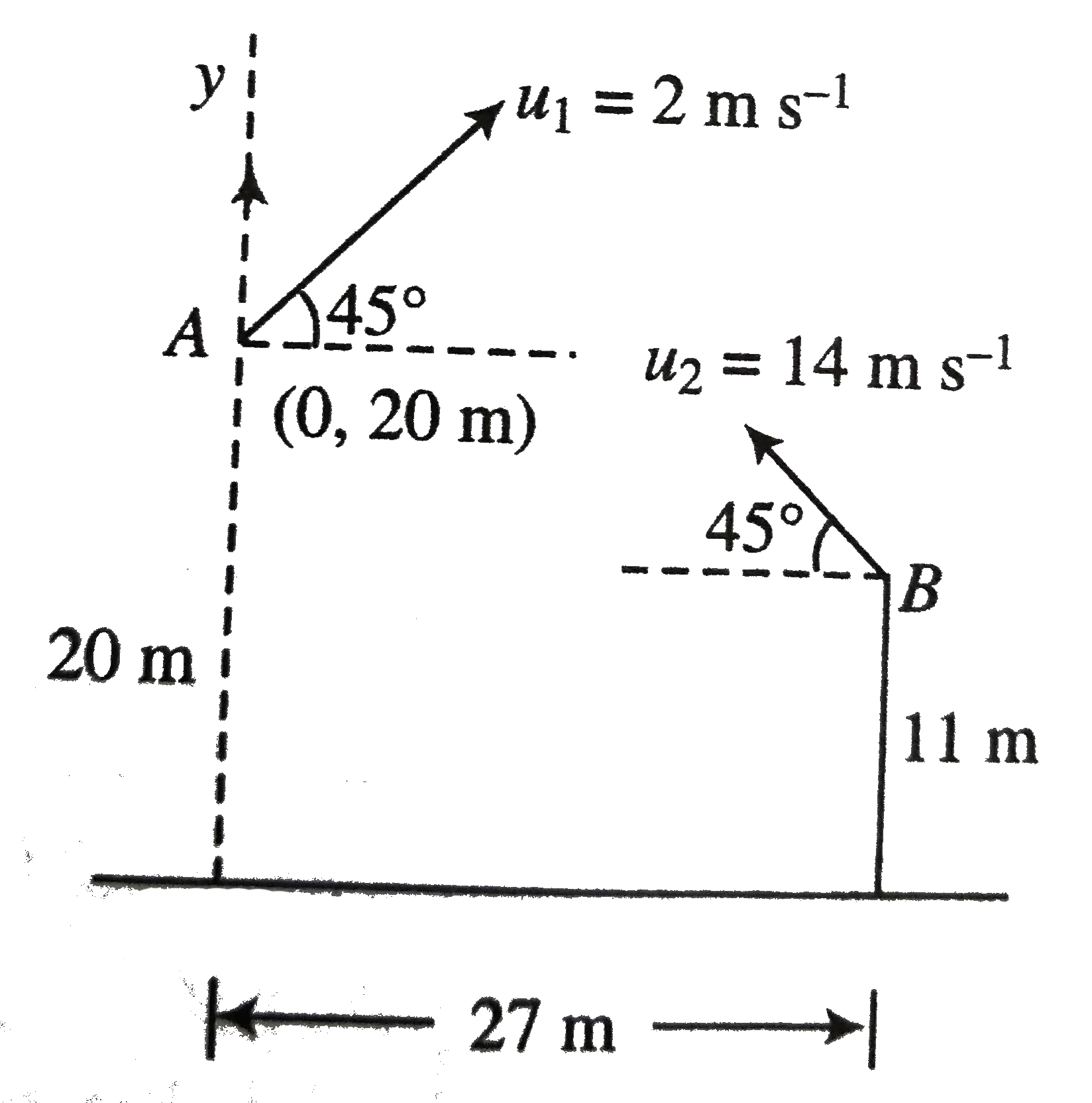
The relative velocity of B as seen from A inView Solution