A student sets uo a slinky PQ on a smooth table top in the manner
How can he produce transverse waves in the slinky by moving its free end Q ?
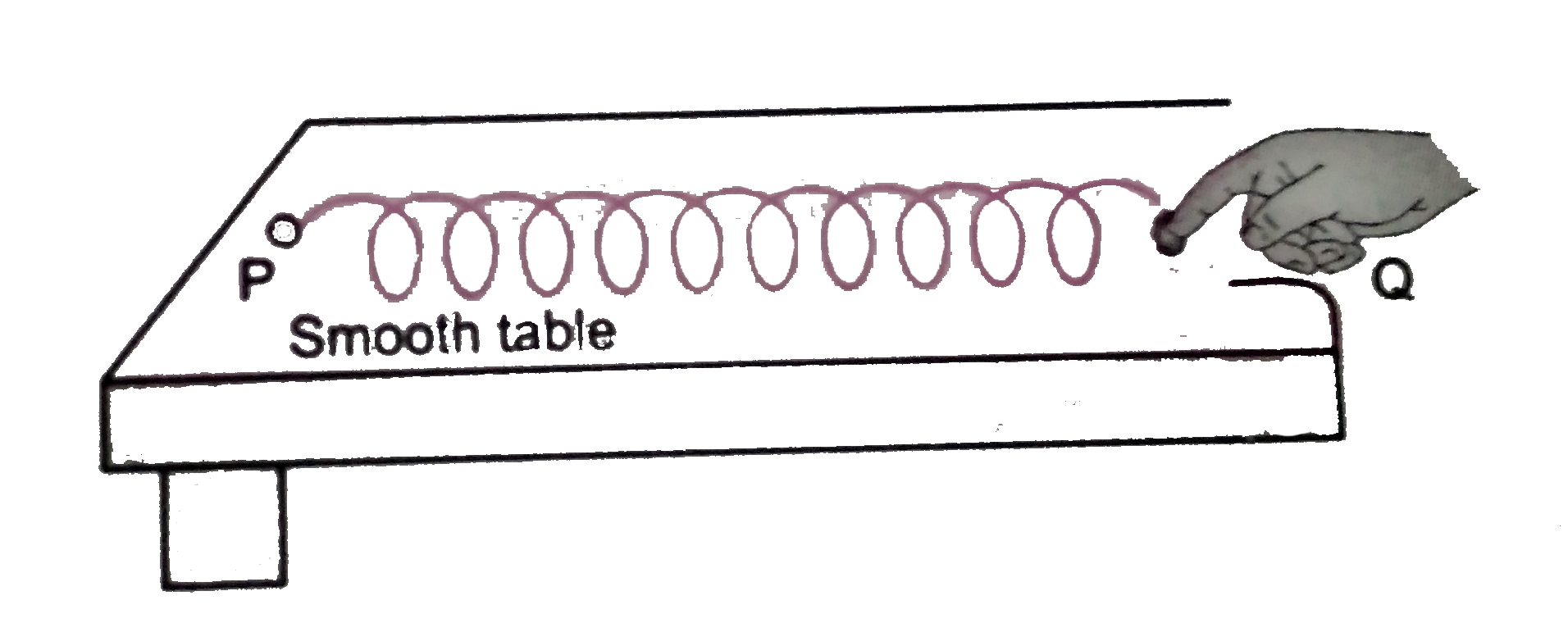 .
.
at an angle of 45∘ with the table top
backward and forward along the length of the slinky
up and down
left and right.
The correct Answer is:C
Step by step video, text & image solution for A student sets uo a slinky PQ on a smooth table top in the manner How can he produce transverse waves in the slinky by moving its free end Q ? . by Physics experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOUNDBOOK - PRADEEPCHAPTER - SOUNDEXERCISE - Mock Test (Sec - A)
24 Videos
- SOUNDBOOK - PRADEEPCHAPTER - SOUNDEXERCISE - Mock Test (Sec - B)
12 Videos
- SOUNDBOOK - PRADEEPCHAPTER - SOUNDEXERCISE - (Exemplar) Multiple Choice
9 Videos
- MOTIONBOOK - PRADEEPCHAPTER - MOTIONEXERCISE - Mock test
24 Videos
- WORK AND ENERGYBOOK - PRADEEPCHAPTER - WORK AND ENERGYEXERCISE - III Quiz Testing
10 Videos
Similar Questions
A chain (AB) of length l loaded in a smooth horizontal table so that its fraction of length h hangs freely and toucher the surface of the table with its end B. At a certain moment ,the end A of the chain is set free. With what velocity will this end the chain slip out of the table ?
 .
.
View SolutionThe water level in a vertical glass tube 1.00 m long can be adjusted to any position in the tube. A tuning fork vibrating at 900 Hz is held just over the open top end of the tube, to set up a standing wave of sound in the air-filled top portion of the tube. (That airfilled top portion acts as a tube with one end closed and the other end open.) (a) For how many different positions of the water level will sound from the fork set up resonance in the tube's air-filled portion? What are the (b) least and (c ) second least water heights in the tube for resonance to occur?
View Solution
Knowledge Check
A string has a linear mass density μ. The ends of the string are joined to form a closed loop and is given the shape of a circular ring of radius R. this ring is now rotated about its axis with an angular velocity ω
Q. The velocity of the transverse wave set up in the string, with respect to an observer at the centre ofAωRB2ωRC3ωRD4ωRA string has a linear mass density μ. The ends of the string are joined to form a closed loop and is given the shape of a circular ring of radius R. this ring is now rotated about its axis with an angular velocity ω
Q. The velocity of a transverse wave set up in the string, with respect to the string isAωRB2ωRC3ωRD4ωRWhen a particle is restricted to move along x-axis between x=0 and x=a, where α if of nenometer dimension, its energy can take only certain specific values. The allowed energies of the particle moving in such a restricted region, correspond to the formation of standing waves with nodes at its ends x=0 and x=a. The wavelength of this standing wave is related to the linear momentum p of the particle according to the de Broglie relation. The energy of the particle of mass m is related to its linear momentum as E=p22m. Thus the energy of the particle can be denoted by a quantum number n taking values 1,2,3, ...(n=1, called the ground state) corresponding to the number of loops in the standing wave.
Use the model described above to answer the following three questions for a particle moving along the line from x=0 to x=α. Take h=6.6×10−34Js and e=1.6×10−19C
Q. The speed of the particle that can take discrete values is proportional toAn−32Bn−1Cn12Dn
A wheel of rdius r and moment of inertia I about its axis is fixed at top of an inclined plane of inclination θ as shownin figure. A string is wrapped round the wheel and its free end supports a block of mass M which can slide on the plane. INitialy, tehwheel is rotating at a speed ω in direction such that the block slides pu the plane. How far wil the block move before stopping?
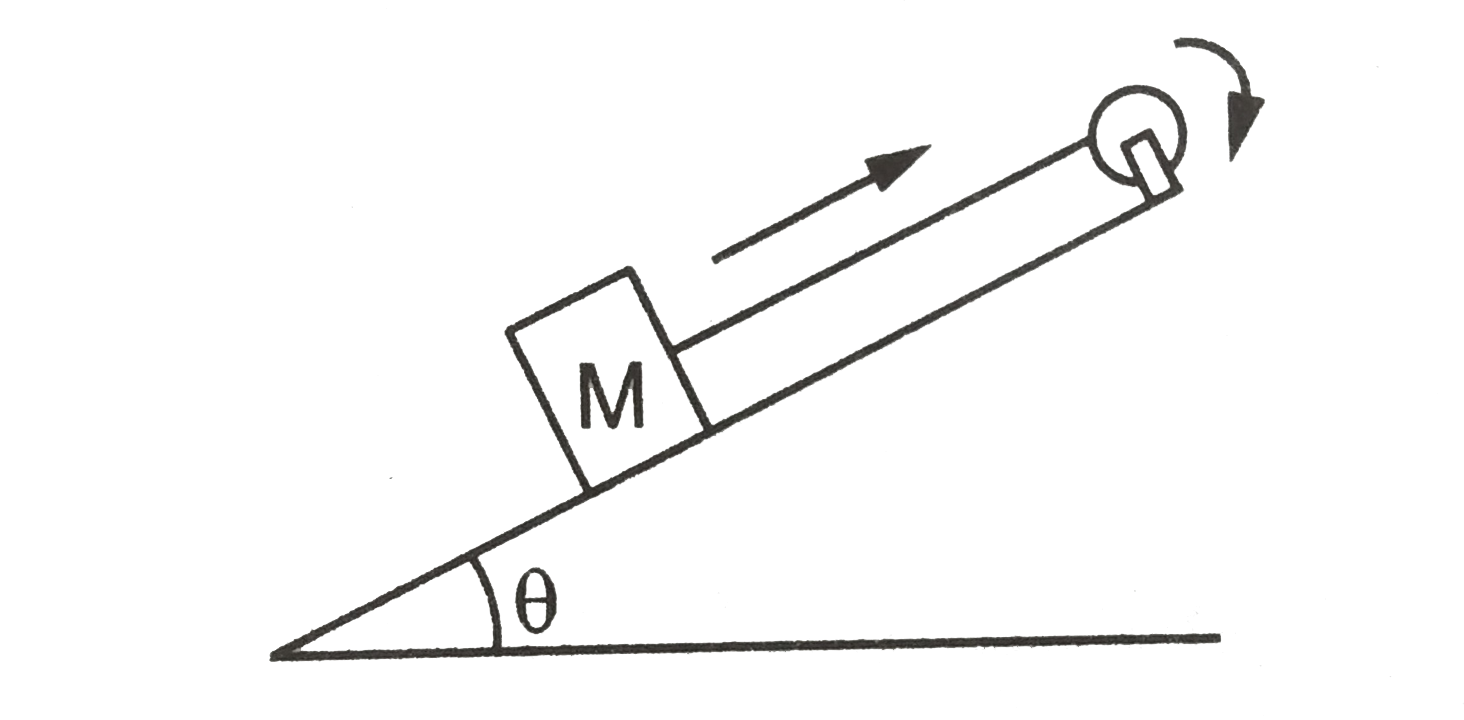
View SolutionA particle of mass m is attached to one end of a weightless and inextensible string of length L. The particle is on a smooth horizontal table. The string passes through a hole in the table and to its other and is attached a small particle of equal mass m. The system is set in motion with the first particle describing a circle on the table with constant angular velocity ω1 and the second particle moving in the horizontal circle as a conical pendulum with constant angular velocity ω2 Show that the length of the portions of the string on either side of the hole are in the ratio ω22:ω21
View SolutionWhen a particle is restricted to move along x-axis between x=0 and x=a, where α if of nenometer dimension, its energy can take only certain specific values. The allowed energies of the particle moving in such a restricted region, correspond to the formation of standing waves with nodes at its ends x=0 and x=a. The wavelength of this standing wave is related to the linear momentum p of the particle according to the de Broglie relation. The energy of the particle of mass m is related to its linear momentum as E=p22m. Thus the energy of the particle can be denoted by a quantum number n taking values 1,2,3, ...(n=1, called the ground state) corresponding to the number of loops in the standing wave.
Use the model described above to answer the following three questions for a particle moving along the line from x=0 to x=α. Take h=6.6×10−34Js and e=1.6×10−19 C.
Q. If the mass of the particle is m=1.0×10−30kg and α=6.6nm, the energy of the particle in its ground state is closest to
View SolutionWhen a particle is restricted to move along x-axis between x=0 and x=a, where α if of nenometer dimension, its energy can take only certain specific values. The allowed energies of the particle moving in such a restricted region, correspond to the formation of standing waves with nodes at its ends x=0 and x=a. The wavelength of this standing wave is related to the linear momentum p of the particle according to the de Broglie relation. The energy of the particle of mass m is related to its linear momentum as E=p22m. Thus the energy of the particle can be denoted by a quantum number n taking values 1,2,3, ...(n=1, called the ground state) corresponding to the number of loops in the standing wave.
Use the model described above to answer the following three questions for a particle moving along the line from x=0 to x=α. Take h=6.6×10−34Js and e=1.6×10−19 C.
Q. The allowed energy for the particle for a particular value of n is proportional to
View SolutionA stretched slinky is given a sharp push along its length. A wave travels from one end to another. The wave so produced is
View Solution