A 2.5 cm candle is placed 12 cm away from a convex mirror of focal length 30 cm. Give the location of the image and the magnification.
The correct Answer is:8.6cm ; 1.8cm
To solve the problem of finding the location of the image and the magnification produced by a convex mirror, we will follow these steps:
Step 1: Identify the given values
- Height of the object (candle), ho=2.5cm
- Object distance, u=−12cm (negative as per sign convention)
- Focal length of the convex mirror, f=+30cm (positive for convex mirrors)
Step 2: Use the mirror formula
The mirror formula is given by:
1f=1v+1u
We can rearrange this to find v:
1v=1f−1u
Step 3: Substitute the values into the formula
Substituting the values we have:
1v=130−1−12
This simplifies to:
1v=130+112
Step 4: Find a common denominator and solve for v
The least common multiple of 30 and 12 is 60. We can rewrite the fractions:
130=260,112=560
Now substituting these values:
1v=260+560=760
Now, taking the reciprocal to find v:
v=607≈8.57cm
Step 5: Determine the nature of the image
Since v is positive, the image formed is virtual and located on the same side as the object.
Step 6: Calculate the magnification
The magnification m is given by:
m=−vu
Substituting the values:
m=−60/7−12=60/712=6084=57≈0.71
Conclusion
- The location of the image is approximately 8.57cm from the mirror (virtual image).
- The magnification is approximately 0.71.
- Height of the object (candle), ho=2.5cm
- Object distance, u=−12cm (negative as per sign convention)
- Focal length of the convex mirror, f=+30cm (positive for convex mirrors)
The mirror formula is given by:
1f=1v+1u
We can rearrange this to find v:
1v=1f−1u
Substituting the values we have:
1v=130−1−12
This simplifies to:
1v=130+112
The least common multiple of 30 and 12 is 60. We can rewrite the fractions:
130=260,112=560
Now substituting these values:
1v=260+560=760
Now, taking the reciprocal to find v:
v=607≈8.57cm
Since v is positive, the image formed is virtual and located on the same side as the object.
The magnification m is given by:
m=−vu
Substituting the values:
m=−60/7−12=60/712=6084=57≈0.71
- The location of the image is approximately 8.57cm from the mirror (virtual image).
- The magnification is approximately 0.71.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - REFLECTION AND REFRACTIONEXERCISE - NCERT EXEMPLAR PROBLEMS19videos
REFLECTION AND REFRACTION
- BOOK - PRADEEPCHAPTER - REFLECTION AND REFRACTIONEXERCISE - Mock Test26videos
REFLECTION AND REFRACTION
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECTS OF ELECTRIC CURRENTEXERCISE - Mock Test21videos
MAGNETIC EFFECTS OF ELECTRIC CURRENT
- BOOK - PRADEEPCHAPTER - SOURCES OF ENERGYEXERCISE - Mock Test20videos
SOURCES OF ENERGY
Similar Questions
A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.
View SolutionA 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror?
View Solution
Knowledge Check
- Question 1 - Select One
A 4.5cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.
A6 cm , 0.718B6.7 cm , 0.558CCannot be determinedDNONE OF THESE - Question 2 - Select One
A 4.5cm needle is placed 12cm away from a convex mirror of focal length 15cm. Given the location of the image and the magniftication.
A6.7cm,5/9B7.5cm,5/9C6.7cm,9/5D7.5cm,9/5 - Question 3 - Select One
A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. The position of image and the magnification respectively are
A3.33,57B6.7cm,1.8C0.15 cm, 1.8D6.7cm,59
An object is placed 15 cm from a convex mirror of radius of curvature 90 cm. Calculate position of the image and its magnification.
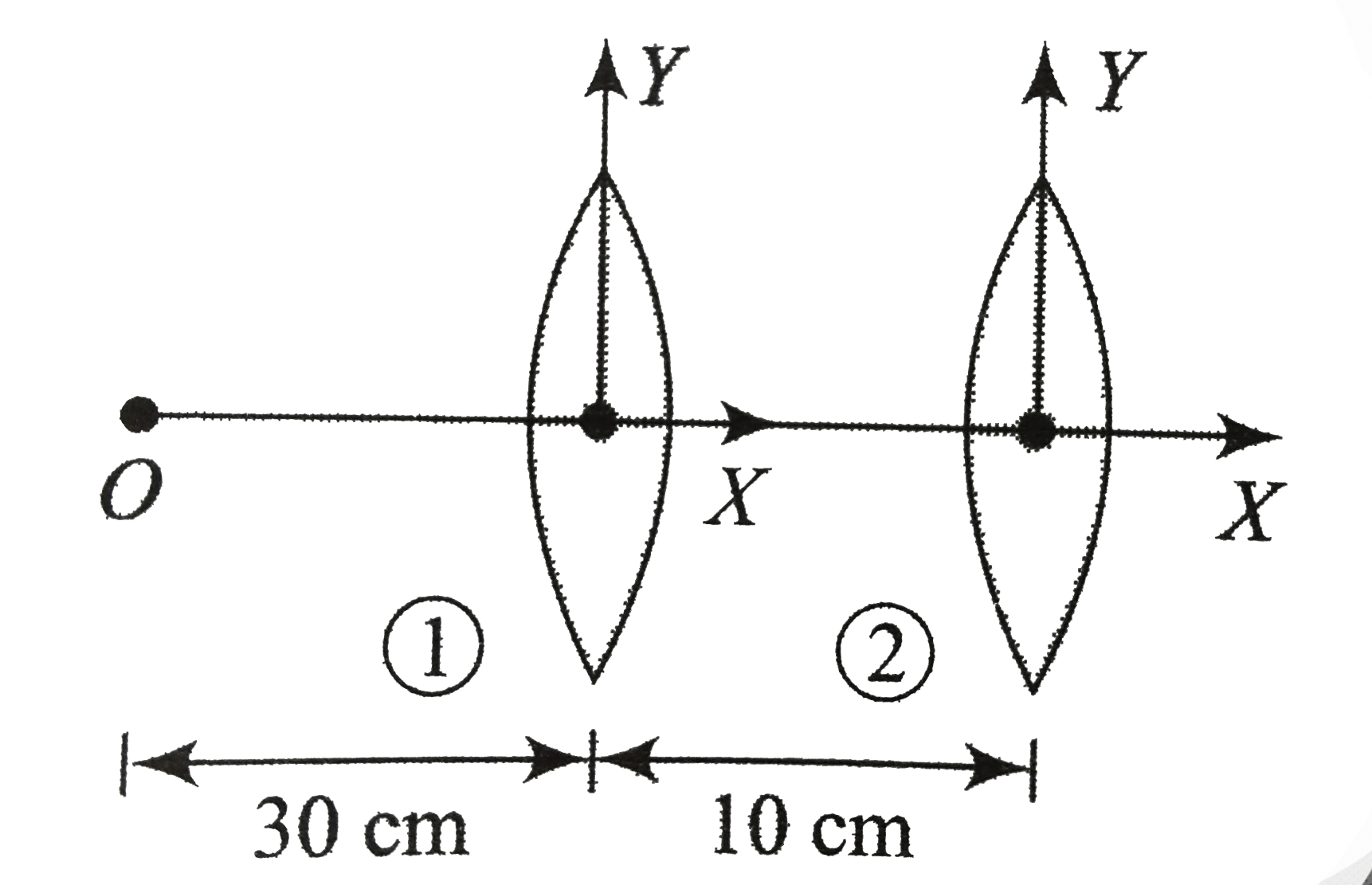
View SolutionA convex lens of focal length 20cm is placed 10cm away from a second convex lens of focal length 25 cm. What wil be the location of the image of an object at 30 cm in front of the first lens?
 View Solution
View SolutionAn object is placed 10 cm in front of a convex mirror of focal length 20 cm . The distance of the image from the mirror is
View SolutionIf an object is 30 cm away from a concave mirror of focal length 15 cm the image will be
View SolutionAn object is placed at 20 cm from a convex mirror of focal length 20 cm. The distance of the image from the pole of the mirror is
View Solution

