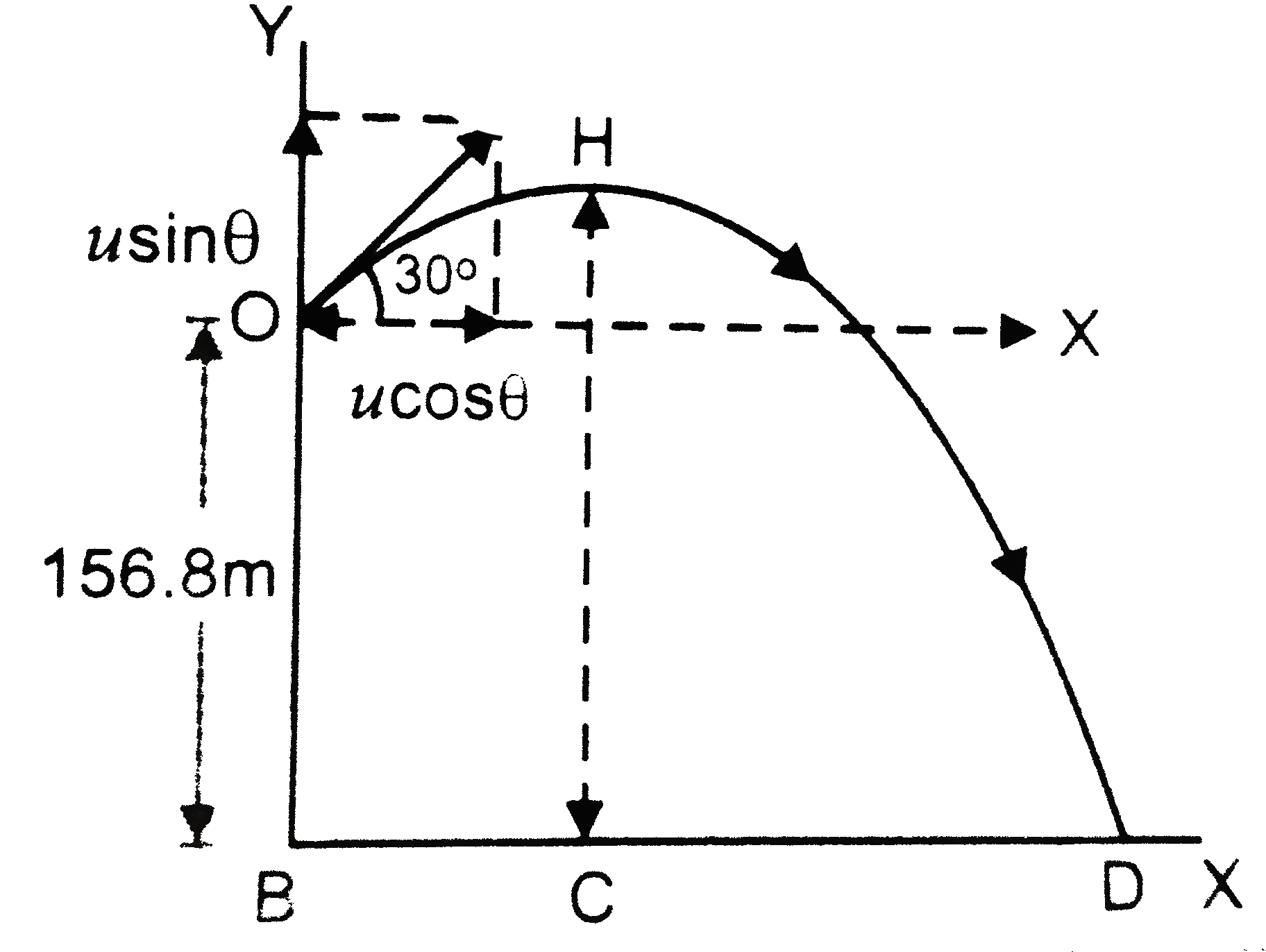
For the top of a tower 156.8m high, a projectile is thrown up with a velcity of 39.2ms−1, makingan angle 30%(∘) with borizontal direction. Find the distance from the foot of tower wher it strikes the ground and the time taken byit do so.
If Fig. 2(d). 26, height tower
OB=156.8m,u=39.2m/s,θ=30∘
Component of velocity along OX
=ucosθ=30∘.2cos30∘=33.947m−10
 .
.
Component of velocity along OY
=usinθ=39.2sin30∘=19.6ms−1
Let (t) be the total time of flight (i.e time in going from O→D).ConsrtheverticaldownwarddirectionOBasthepositivedirectionofy−aξs.Tak∈gmotionofaprojecti≤omO to DalongY−aξs,wehave<br>y_(0) =0, y= 156.8 m,<br>u_(y) =- sin 30^(@) =- 19.6 m//s,<br>a_(y) =9.8 m//s^(2) , t=t<br>As,y=y0+uyt+12YT2 ltBRgt :. 156. 8 =0 + (-19.6) t+ 1/2 xx 9.8 xx t^(2)<br>or156.8=−19.6+4.9t2
or 4.9t2−19.6t156.8=0
or t2−4t−32=0
ort2−8t+4t−32=0
or t(t−8)+4(t−8)=0
or (t−4)(t−8)=0ort=−4or8.
As t=−4sis¬possib≤∴t=8 s<br>Distanceomthef∞tof→werwheritstrikesthegroundis
BD=ucos30∘×t=33.947×=271.57m.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 Conceptual Problems2videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 Con ceptual Problems14videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 3 SolvedExamples46videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions19videos
GRAVIATION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions17videos
LAWS OF MOTION
Similar Questions
A ball is thrown from the top of a tower of 61m high with a velocity 24.4ms−1 at an elevation of 30∘ above the horizontal. What is the distance from the foot of the tower to the point where the ball hits the ground?
View SolutionA ball is thrown with velocity 40m/s at angle 30∘ with horizontal from the top of a tower of height 60m. At what horizontal distance from the foot of tower, the ball will strike the gorund ?
View Solution
Knowledge Check
- Question 1 - Select One
A ball is thrown from the top of tower with an initial velocity of 10ms−1 at an angle of 30∘ with the horizontal. If it hits the ground of a distance of 17.3m from the back of the tower, the height of the tower is (Takeg=10ms−2)
A5mB20mC15mD10m - Question 2 - Select One
From the top of a tower of height 40m, a ball is projected upward with a speed of 20ms−1 at an angle of elevation of 30∘. Then the ratio of the total time taken by the ball to hit the ground to the time taken to ball come at same level as top of tower.
A2:1B3:1C3:2D4:1 - Question 3 - Select One
From the top of a tower, 80m high from the ground a stone is thrown in the horizontal direction with a velocity of 8ms1. The stone reaches the ground after a time t and falls at a distance of d from the foot of the tower. Assuming g=10ms2, the time t and distance d are given respectively by
A6s,64mB6s,48mC4s,32mD4s,16m
A particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
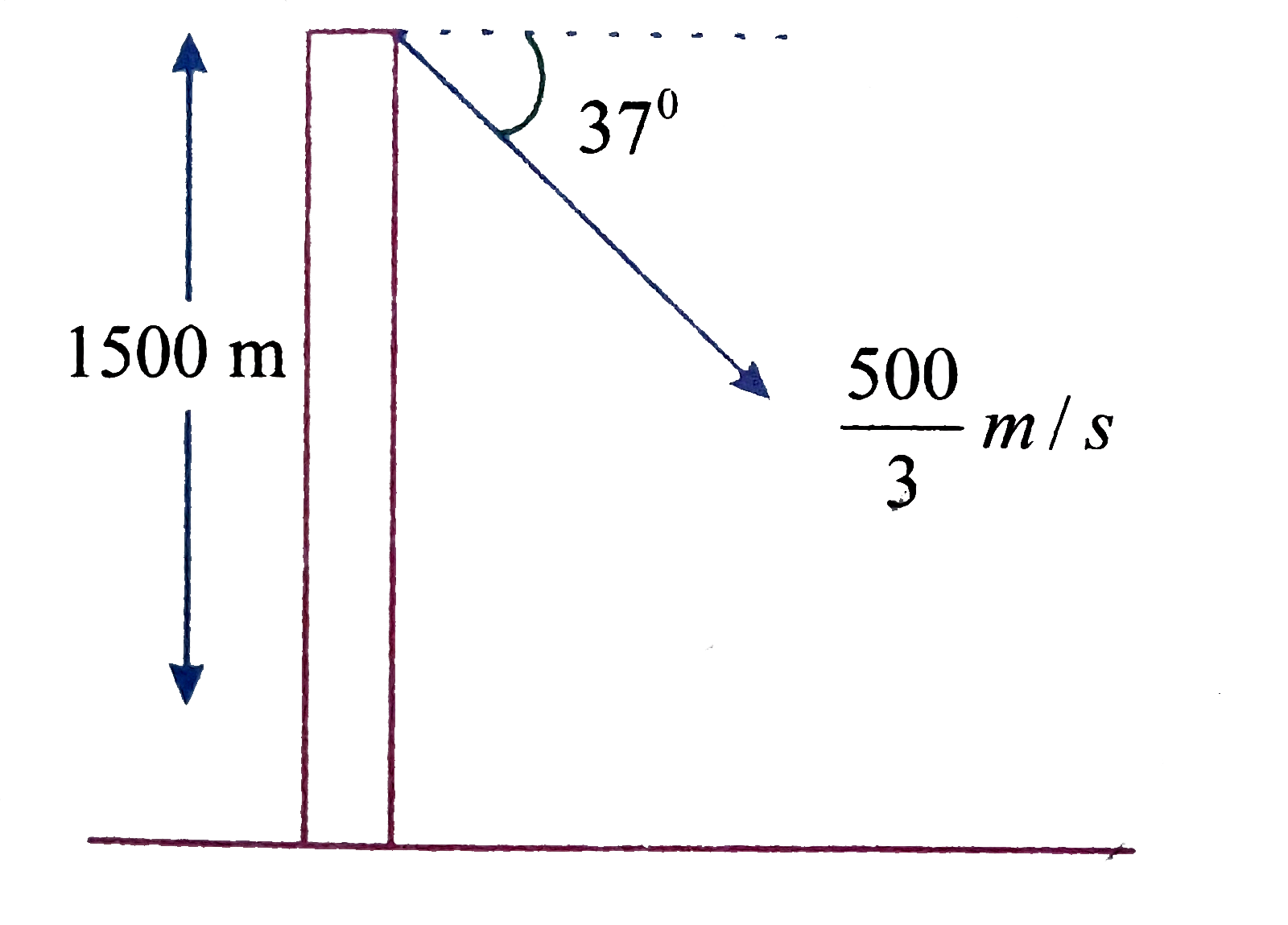 View Solution
View SolutionFrom the top of a tower 180 m high, it was observed that the angle of depression of the bottom of a cat sitting on the ground was 30∘. Find the distance of the cat from the foot of the tower.
View SolutionA stone is thrown up from the top of a tower 20,m with a velocity of 24m/s a tan elevation of 30∘ above the horizontal. Find the horizontal distance from the foot of the tower to the point at which the stone hits the ground. Take g=10m/s2
View SolutionA ball is thrown from the top of a 35m high tower with initial velocity of magnitude u=80 ms−1 at an angle 25∘ with horizontal.
Find the time to reach the ground and the horizontal distance covered by the ball.View SolutionFrom the top of a tower, two balls are thrown horizontally with velocities u1 and u2 in opposite directions. If their velocities are perpendicular to each other just before they strike the ground, find the height of tower.
View Solution

