Show that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion.
To show that the angular momentum about any point of a single particle moving with constant velocity remains constant throughout the motion, we can follow these steps:
Step 1: Define Angular Momentum
The angular momentum L of a particle about a point O is given by the vector cross product:
L=R×P
where R is the position vector from point O to the particle, and P is the linear momentum of the particle.
Step 2: Express Linear Momentum
The linear momentum P of the particle is given by:
P=MV
where M is the mass of the particle and V is its velocity.
Step 3: Determine the Position Vector
Let R be the position vector from point O to the particle. The magnitude of R can be expressed as:
R=|R|
The angle θ between the position vector R and the velocity vector V is important for calculating the sine component.
Step 4: Calculate the Magnitude of Angular Momentum
The magnitude of angular momentum can be expressed as:
L=|R||P|sinθ
Substituting P=MV:
L=R⋅(MV)sinθ
Step 5: Analyze the Geometry
In the scenario, the distance D from point O to the line of motion of the particle is constant. This distance can be expressed in terms of R and θ:
D=Rsinθ
Thus, we can rewrite the angular momentum as:
L=MVD
Step 6: Establish Constants
Since the mass M, the velocity V, and the distance D (the perpendicular distance from point O to the line of motion) are all constant, we can conclude:
L=MVD(constant)
Conclusion
Therefore, the angular momentum L about point O remains constant throughout the motion of the particle moving with constant velocity.
---
The angular momentum L of a particle about a point O is given by the vector cross product:
L=R×P
where R is the position vector from point O to the particle, and P is the linear momentum of the particle.
The linear momentum P of the particle is given by:
P=MV
where M is the mass of the particle and V is its velocity.
Let R be the position vector from point O to the particle. The magnitude of R can be expressed as:
R=|R|
The angle θ between the position vector R and the velocity vector V is important for calculating the sine component.
The magnitude of angular momentum can be expressed as:
L=|R||P|sinθ
Substituting P=MV:
L=R⋅(MV)sinθ
In the scenario, the distance D from point O to the line of motion of the particle is constant. This distance can be expressed in terms of R and θ:
D=Rsinθ
Thus, we can rewrite the angular momentum as:
L=MVD
Since the mass M, the velocity V, and the distance D (the perpendicular distance from point O to the line of motion) are all constant, we can conclude:
L=MVD(constant)
Therefore, the angular momentum L about point O remains constant throughout the motion of the particle moving with constant velocity.
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE B
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE C
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE A
2 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Knowledge Check
A particle moves with constant angular velocity in a circle. During the motion its
AEnergy is conservedBMomentum is conservedCEnergy and momentum both are conservedDNone of the above is conservedThe angular momentum of a moving body remains constant if
Anet external force is appliedBnet external torque is appliedCnet pressure is appliedDnet external torque is not applied .Assertion: If linear momentum of a particle is constant, then its angular momentum about any point will also remain constant.
Reason: Linear momentum remains constant if Fnet=0 and angular momentum remains constant if τnet=0AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf assertion is true, but the reaction is false.DIf assertion is false but the reason is true.
Which of the following graphs represents the motion of a particle moving with constant velocity?
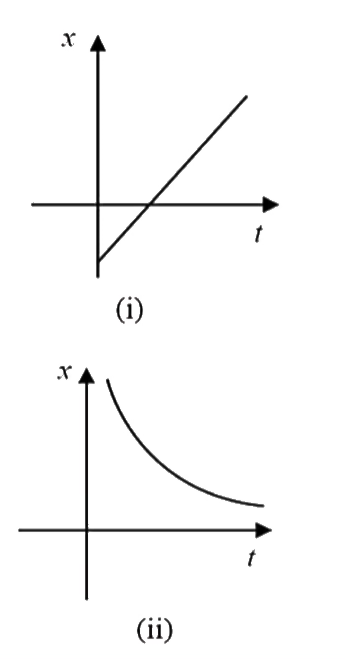
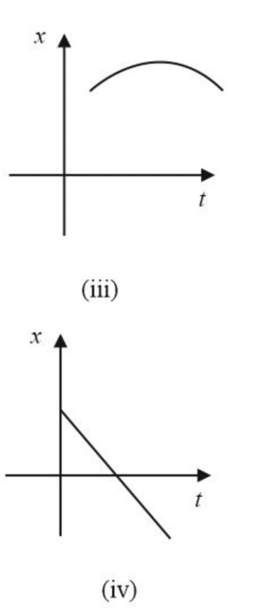
View SolutionShow that the angular momentum of a particle is equal to twice the product of mass and areal velocity.
View SolutionShow that the angular momentum of a particle is equal to twice the product of mass and areal velocity.
View SolutionAngular momentum of the particle rotating with a central force is constant due to
View SolutionAngular momentum of the particle rotating with a central force is constant due to
View Solution
