Calculate moment of inertia of a circular disc about a transverse axis through the centre of the disc. Given, diameter of disc is 40cm, thickness =7cm and density of material of disc =9gcm−3.
To calculate the moment of inertia of a circular disc about a transverse axis through its center, we can follow these steps:
Step 1: Identify Given Values
- Diameter of the disc, D=40cm
- Radius of the disc, R=D2=402=20cm=0.2m
- Thickness of the disc, t=7cm=0.07m
- Density of the material, ρ=9g/cm3=9×103kg/m3
Step 2: Calculate the Volume of the Disc
The volume V of the disc can be calculated using the formula:
V=Area×Thickness=πR2t
Substituting the values:
V=π(0.2)2(0.07)=π(0.04)(0.07)=π(0.0028)m3
Step 3: Calculate the Mass of the Disc
The mass m of the disc can be calculated using the formula:
m=ρV
Substituting the values:
m=9×103kg/m3×π(0.0028)m3
Step 4: Calculate the Moment of Inertia
The moment of inertia I of a disc about an axis through its center is given by:
I=12mR2
Substituting the values of m and R:
I=12(9×103×π(0.0028))(0.2)2
Calculating R2:
R2=(0.2)2=0.04m2
Now substituting this back:
I=12(9×103×π(0.0028))(0.04)
Step 5: Simplify and Calculate
Calculating the numerical values:
I=12×9×103×π×0.0028×0.04
Calculating π≈3.14:
I=12×9×103×3.14×0.0028×0.04
I≈12×9×103×3.14×0.000112
I≈12×9×103×0.00035168
I≈1.58kg m2
Final Answer
The moment of inertia of the circular disc about the transverse axis through its center is approximately:
I≈1.58kg m2
---
- Diameter of the disc, D=40cm
- Radius of the disc, R=D2=402=20cm=0.2m
- Thickness of the disc, t=7cm=0.07m
- Density of the material, ρ=9g/cm3=9×103kg/m3
The volume V of the disc can be calculated using the formula:
V=Area×Thickness=πR2t
Substituting the values:
V=π(0.2)2(0.07)=π(0.04)(0.07)=π(0.0028)m3
The mass m of the disc can be calculated using the formula:
m=ρV
Substituting the values:
m=9×103kg/m3×π(0.0028)m3
The moment of inertia I of a disc about an axis through its center is given by:
I=12mR2
Substituting the values of m and R:
I=12(9×103×π(0.0028))(0.2)2
Calculating R2:
R2=(0.2)2=0.04m2
Now substituting this back:
I=12(9×103×π(0.0028))(0.04)
Calculating the numerical values:
I=12×9×103×π×0.0028×0.04
Calculating π≈3.14:
I=12×9×103×3.14×0.0028×0.04
I≈12×9×103×3.14×0.000112
I≈12×9×103×0.00035168
I≈1.58kg m2
The moment of inertia of the circular disc about the transverse axis through its center is approximately:
I≈1.58kg m2
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PROBLEMS FOR PRACTICE TYPE E1videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - MULTIPLE CHOICE QUESTIONS-I1videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PROBLEMS FOR PRACTICE TYPE C2videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)25videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions19videos
THERMODYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The moment of inertia of a uniform semicircular disc of mass disc through the centre is
A25Mr2B14Mr2C12Mr2DMr2 - Question 2 - Select One
The moment of inertia of a circular disc about an axis passing through the circumstances perpendicular to the plane of the disc is
A(MR2B32MR[^(2)CMR22D54MR2 - Question 3 - Select One
The moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is.
A12MR2BMR2C25MR2D32MR2
Calculate the moment of inertia of uniform circular disc of mass 500 g, radius 10 cm about : diameter of the disc
View SolutionCalculate the moment of inertia of uniform circular disc of mass 500 g, radius 10 cm about : the axis through the centre of the disc and perpendicular to its plane
View SolutionCalculate the ratio of moment of inertia of a thin uniform disc about axis 1 and 2 marked in the figure. O is the centre of the disc.
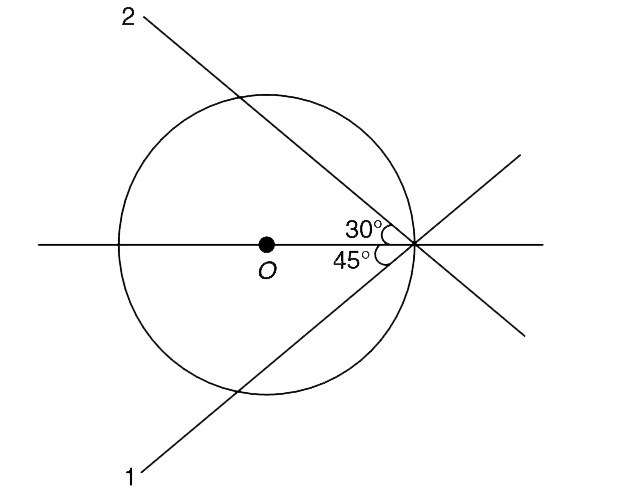 View Solution
View SolutionCalculate the moment of inertia of uniform circular disc of mass 500 g, radius 10 cm about : the axis tangent to the disc and parallel to its diameter
View SolutionThe moment of inertia of a uniform circular disc is maximum about an axis perpendicular to the disc and passing through.
 .View Solution
.View Solution

