An isosceles triangular plates of base 3 m and altitude 3 m is immersed in oil vertically with its base coinciding with its base coinciding with the free surface of the oil of relative density 0.8. Determine the total thrust.
24 N
48 N
36 N
None of these
The correct Answer is:C
To determine the total thrust on the isosceles triangular plate immersed in oil, we can follow these steps:
Step 1: Identify the dimensions of the triangle
The base b of the triangle is given as 3 m, and the altitude h is also given as 3 m.
Step 2: Calculate the area of the triangle
The area A of a triangle can be calculated using the formula:
A=12×base×height
Substituting the values:
A=12×3m×3m=92m2=4.5m2
Step 3: Determine the depth of the center of mass
For an isosceles triangle, the center of mass is located at a distance of h3 from the base. Since the altitude h is 3 m:
Depth of center of mass=33=1m
Step 4: Calculate the pressure at the center of mass
The pressure P at a depth h in a fluid is given by:
P=ρ⋅g⋅h
Where:
- ρ is the density of the fluid (oil in this case),
- g is the acceleration due to gravity (approximately 10m/s2),
- h is the depth of the center of mass.
Given that the relative density of oil is 0.8, the density ρ of oil can be calculated as:
ρ=0.8×1000kg/m3=800kg/m3
Now substituting the values into the pressure formula:
P=800kg/m3×10m/s2×1m=8000Pa
Step 5: Calculate the total thrust (force)
The total thrust F on the triangular plate can be calculated using the formula:
F=P⋅A
Substituting the values we have:
F=8000Pa×4.5m2=36000N=36kN
Final Answer
The total thrust on the isosceles triangular plate is 36 kN.
---
The base b of the triangle is given as 3 m, and the altitude h is also given as 3 m.
The area A of a triangle can be calculated using the formula:
A=12×base×height
Substituting the values:
A=12×3m×3m=92m2=4.5m2
For an isosceles triangle, the center of mass is located at a distance of h3 from the base. Since the altitude h is 3 m:
Depth of center of mass=33=1m
The pressure P at a depth h in a fluid is given by:
P=ρ⋅g⋅h
Where:
- ρ is the density of the fluid (oil in this case),
- g is the acceleration due to gravity (approximately 10m/s2),
- h is the depth of the center of mass.
ρ=0.8×1000kg/m3=800kg/m3
Now substituting the values into the pressure formula:
P=800kg/m3×10m/s2×1m=8000Pa
The total thrust F on the triangular plate can be calculated using the formula:
F=P⋅A
Substituting the values we have:
F=8000Pa×4.5m2=36000N=36kN
The total thrust on the isosceles triangular plate is 36 kN.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Buoyancy And Floatation
40 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Continuity Equation And Bernoulli'S Theorem
32 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
Similar Questions
A isosceles triangle with each base angle equal to 30∘ is inscribed in the ellipsex2a2+y2b2=1. If the base of the triangle coincides with the major axis of the ellipse, then find its eccentricity
View SolutionThe figure shows an isosceles triangular plate of mass M and base L. The angle at the apex is 90∘ . The apex lies at the origin and the base is parallel to X - axis.
The moment of inertia of the plate about its base parallel to the x-axis is

View Solution
Knowledge Check
A triangular lamina of area A and height h is immersed in a liquid of density ρ in a vertical plane with its base on the surface of the liquid. The thrust on the lamina is.
A12AρghB13AρghC16AρghD23AρghAn isosceles triangle made of wood of base 10 feet and height 8 feet is placed vertically with its base on the ground and vertex directly above it. If the triangle faces the sun whose altitude is 30∘, then the tangent of the angle at the apex of the shadow is
A80√3B80√3167C892√3D80√3217An isosceles triangle of wood of base 10 feet and height 8√3 feet is placed vertically with its base on the ground and vertex directly above. The triangle faces the sun whose altitude is 30∘. Then, the tangent of the angle at the apex of the shadow is
A80B8039C892D80217
The vertical angle of a cone in order that the centre of gravity of the whole surface including its plane base may coincide with the centre of gravity of its volume is . . . . . . . . . .
View SolutionIf density of a substance is xkg/m3, find its relative density.
View SolutionA metal plate measuing 2m x 1m is immersed in water and is held there with is shorter side parallel to the free surface at a depth of 5 meters. Calculate the thrust on the plate , (i) when it is horizontal (ii) when it is vertical ,(iii) when it is at an inclination of 30∘ with the vertical .
View SolutionIf the density of iron is 7900kg m−3 , then its relative density is
View SolutionThe figure shows an isosceles triangle plate of mass M and base L.
The angle at the apex is 90∘. The apex lies at the origin and the base is parallel to X−axis.
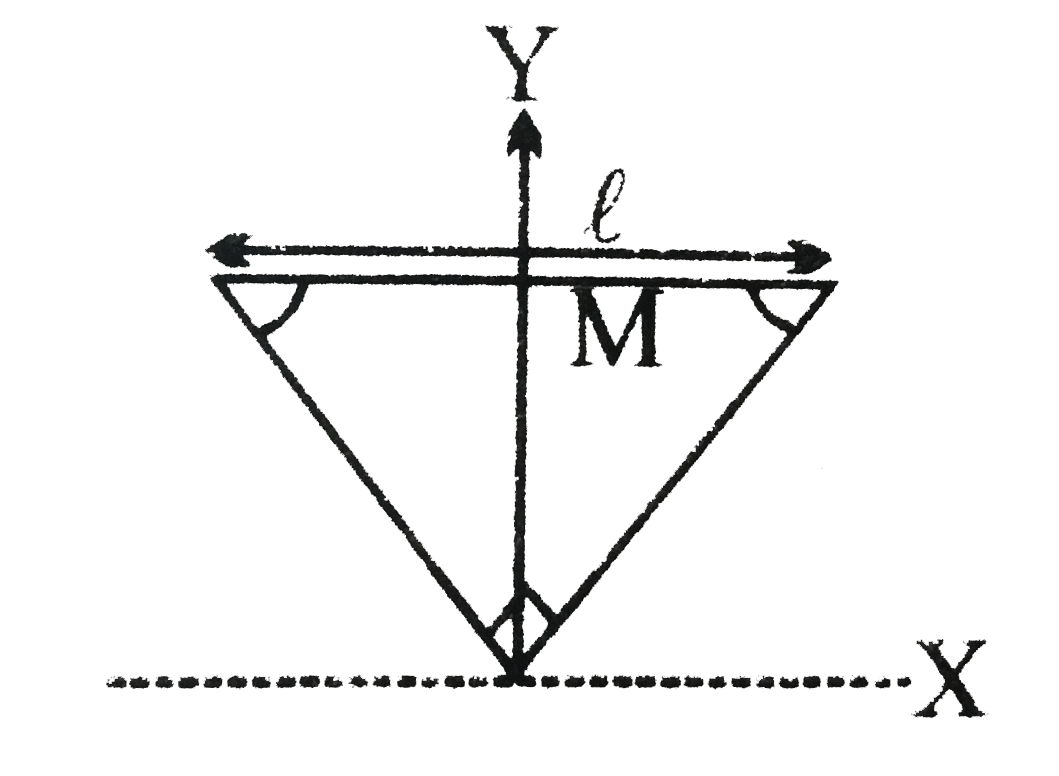
The moment of inertia of the plate about its base parallel to the x−axis is
View Solution