Two non-mixing liquids of densities ρ and (n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length pL(p<1) in the denser liquid. The density d is equal to :
{1+(n+1)p}ρ
{2+(n+1)p}ρ
{2+(n−1)p}ρ
{1+(n−1)p}ρ
The correct Answer is:D
To solve the problem step by step, we need to analyze the situation involving the two non-mixing liquids and the floating cylinder.
Step 1: Understand the setup
We have two liquids:
- The upper liquid has a density of ρ.
- The lower liquid has a density of nρ (where n>1).
- The height of each liquid is h.
- A solid cylinder of length L and density d is placed in this container, floating vertically.
Step 2: Determine the length of the cylinder submerged
The problem states that the cylinder floats with its axis vertical and that a length pL (where p<1) of the cylinder is submerged in the denser liquid (the one with density nρ). Therefore, the length of the cylinder submerged in the upper liquid (density ρ) is:
Lupper=L−pL=(1−p)L
Step 3: Calculate the buoyant force from each liquid
The buoyant force is equal to the weight of the liquid displaced by the submerged part of the cylinder.
1. Buoyant force from the upper liquid (density ρ):
- Volume displaced in the upper liquid:
Vupper=A⋅(1−p)L
- Buoyant force from the upper liquid:
U1=Vupper⋅ρ⋅g=A(1−p)L⋅ρ⋅g
2. Buoyant force from the lower liquid (density nρ):
- Volume displaced in the lower liquid:
Vlower=A⋅pL
- Buoyant force from the lower liquid:
U2=Vlower⋅(nρ)⋅g=A(pL)⋅(nρ)⋅g
Step 4: Set up the equilibrium condition
For the cylinder to float, the total buoyant force must equal the weight of the cylinder. Thus, we have:
U1+U2=Weight of the cylinder
A(1−p)L⋅ρ⋅g+A(pL)⋅(nρ)⋅g=A⋅L⋅d⋅g
Step 5: Simplify the equation
We can cancel A and g from all terms:
(1−p)L⋅ρ+pL⋅nρ=L⋅d
Dividing through by L (since L≠0):
(1−p)ρ+pnρ=d
Step 6: Factor out ρ
ρ(1−p+pn)=d
This simplifies to:
d=ρ(1+p(n−1))
Final Result
Thus, the density d of the cylinder is given by:
d=ρ(1+p(n−1))
We have two liquids:
- The upper liquid has a density of ρ.
- The lower liquid has a density of nρ (where n>1).
- The height of each liquid is h.
- A solid cylinder of length L and density d is placed in this container, floating vertically.
The problem states that the cylinder floats with its axis vertical and that a length pL (where p<1) of the cylinder is submerged in the denser liquid (the one with density nρ). Therefore, the length of the cylinder submerged in the upper liquid (density ρ) is:
Lupper=L−pL=(1−p)L
The buoyant force is equal to the weight of the liquid displaced by the submerged part of the cylinder.
- Volume displaced in the upper liquid:
Vupper=A⋅(1−p)L
- Buoyant force from the upper liquid:
U1=Vupper⋅ρ⋅g=A(1−p)L⋅ρ⋅g
- Volume displaced in the lower liquid:
Vlower=A⋅pL
- Buoyant force from the lower liquid:
U2=Vlower⋅(nρ)⋅g=A(pL)⋅(nρ)⋅g
For the cylinder to float, the total buoyant force must equal the weight of the cylinder. Thus, we have:
U1+U2=Weight of the cylinder
A(1−p)L⋅ρ⋅g+A(pL)⋅(nρ)⋅g=A⋅L⋅d⋅g
We can cancel A and g from all terms:
(1−p)L⋅ρ+pL⋅nρ=L⋅d
Dividing through by L (since L≠0):
(1−p)ρ+pnρ=d
ρ(1−p+pn)=d
This simplifies to:
d=ρ(1+p(n−1))
Thus, the density d of the cylinder is given by:
d=ρ(1+p(n−1))
Topper's Solved these Questions
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - AIIMS Questions
9 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Chapter Test
29 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Assertion Reasoning
15 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
Similar Questions
A container of a large uniform cross-sectional area A resting on a horizontal surface holds two immiscible, non viscous and incompressible liquids of densities d and 2d, each of height H/2 as shown in figure. The lower density liquid is open to atmosphere. A homogeneous solid cylinder of length L(L<H2), cross-sectional area A/5 is immersed such that it floats with its axis vertical of the liquid-liquid interface with length L/4 denser liquid. Determine
(a) density D of the solid and
(b) the total pressure at the bottom of the container. (Atmospheric pressure =P0).
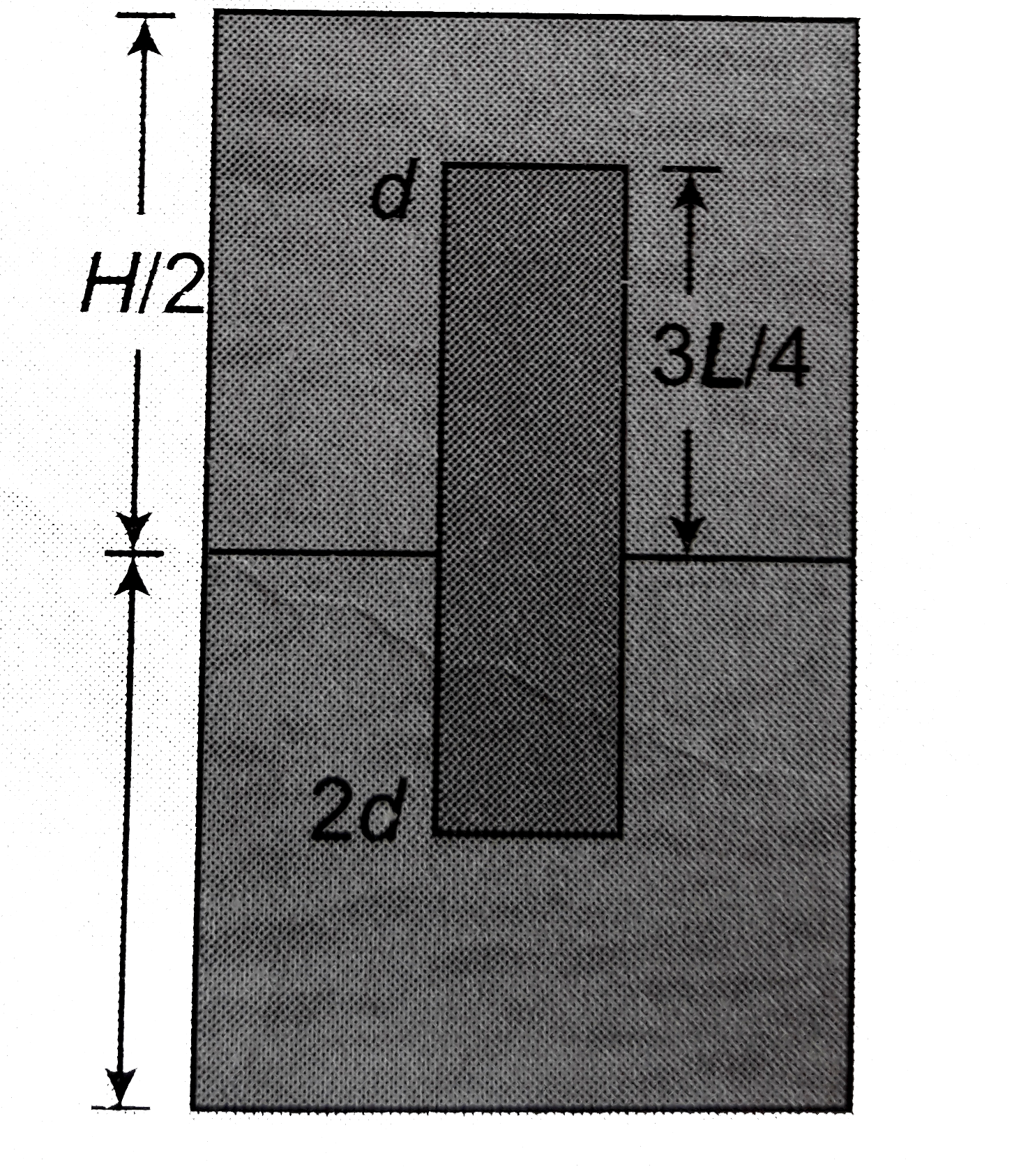 .
.
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holds two-immiscible, non-viscous & incompressible liquids of densities d & 2d, each of height H/2. The lower density liquid is open to the atmoshpere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) corss-sectional area A/5 is immersed such that it floats with its axis vertical at the liquid interface with the length L/4 in the denser liquid. Determine : (i) The density D of the solid & (ii) The total pressure at the bottom of the container.
View Solution
Knowledge Check
Liquid of density ρ in a container exerts pressure P given by (height of liquid h and area A)
AP=AhρgBp=hρgCP=hρgADP=ρgAhIf equal masses of two liquids of densities d1andd2 are mixed together, the density of the mixture is
Ad1d2(d1+d2)B2d1d2(d1+d2)Cd1d22(d1+d2)D(d1+d2)2Fluids at rest exert a normal force to the walls of the container or to the sruface of the Body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.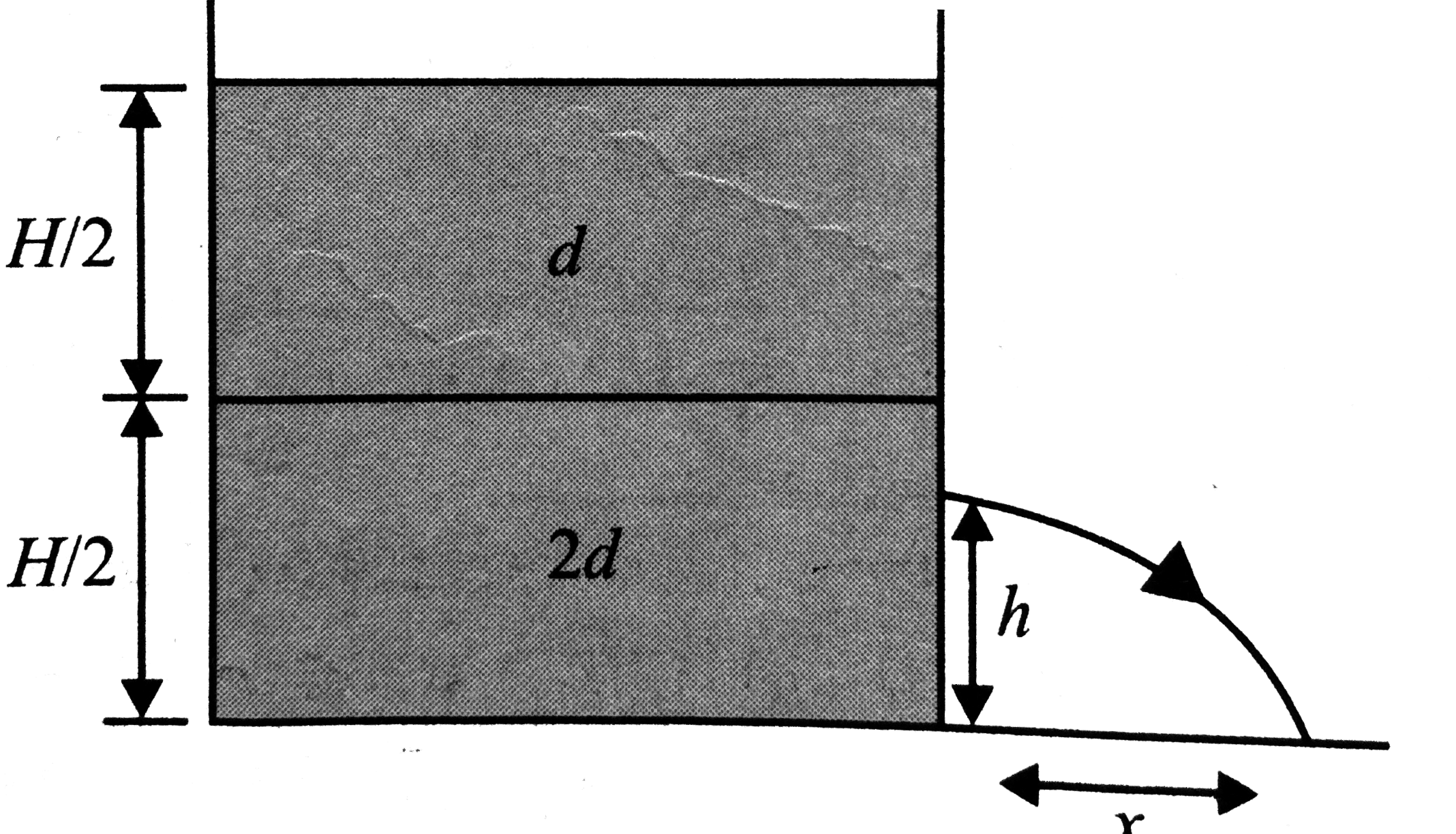
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The density of the solid isA5d4Bd4C2d4D3d4
Three immiscible liquids of densities d1>d2>d3 and refractive indices μ1>μ2>μ3 are put in a beaker. The height of each liquid column is h3. A dot is made at the bottom of the beaker. For near normal vision, find the apparent depth of the dot.
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
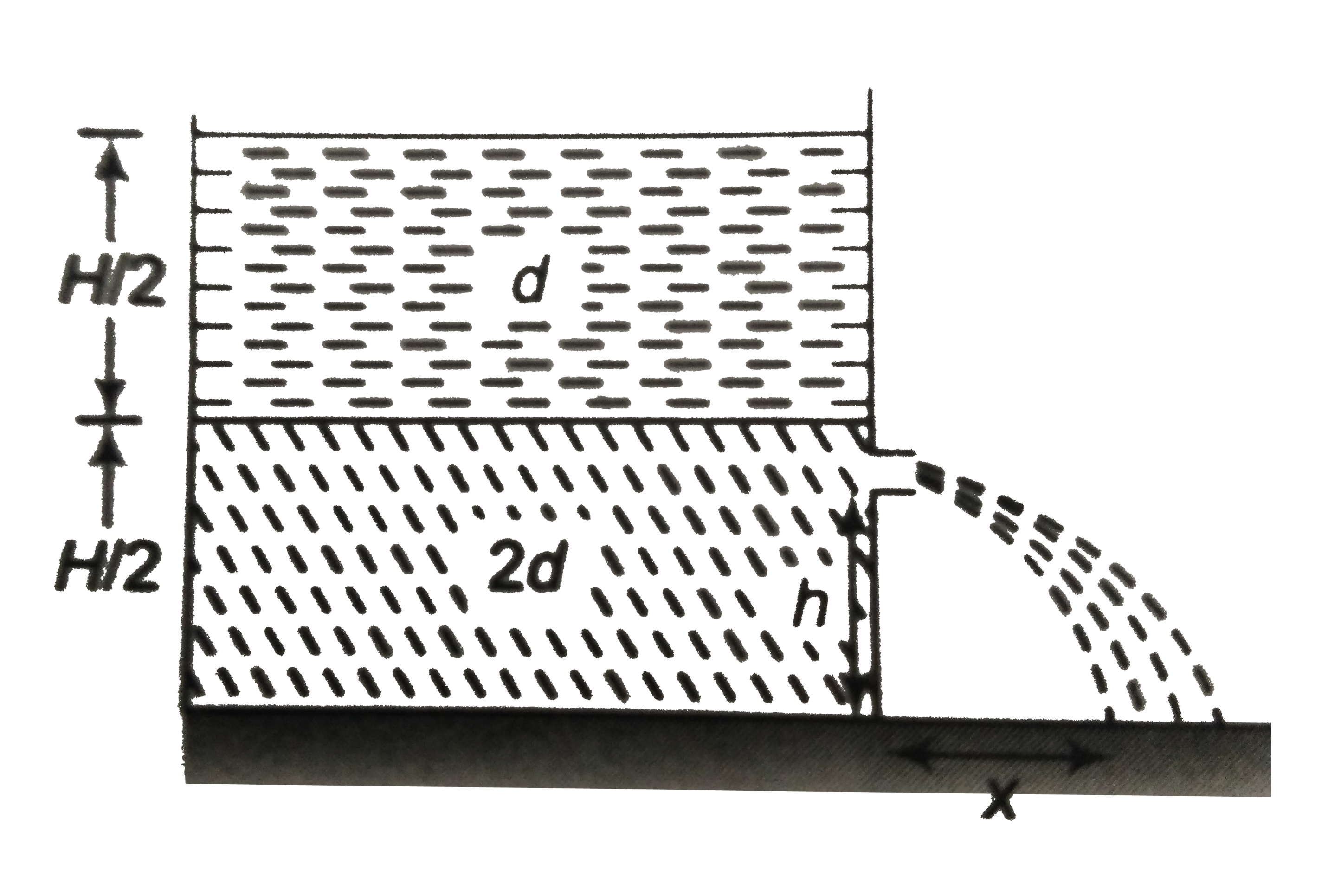
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The density D of the material of the floation cylinder is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
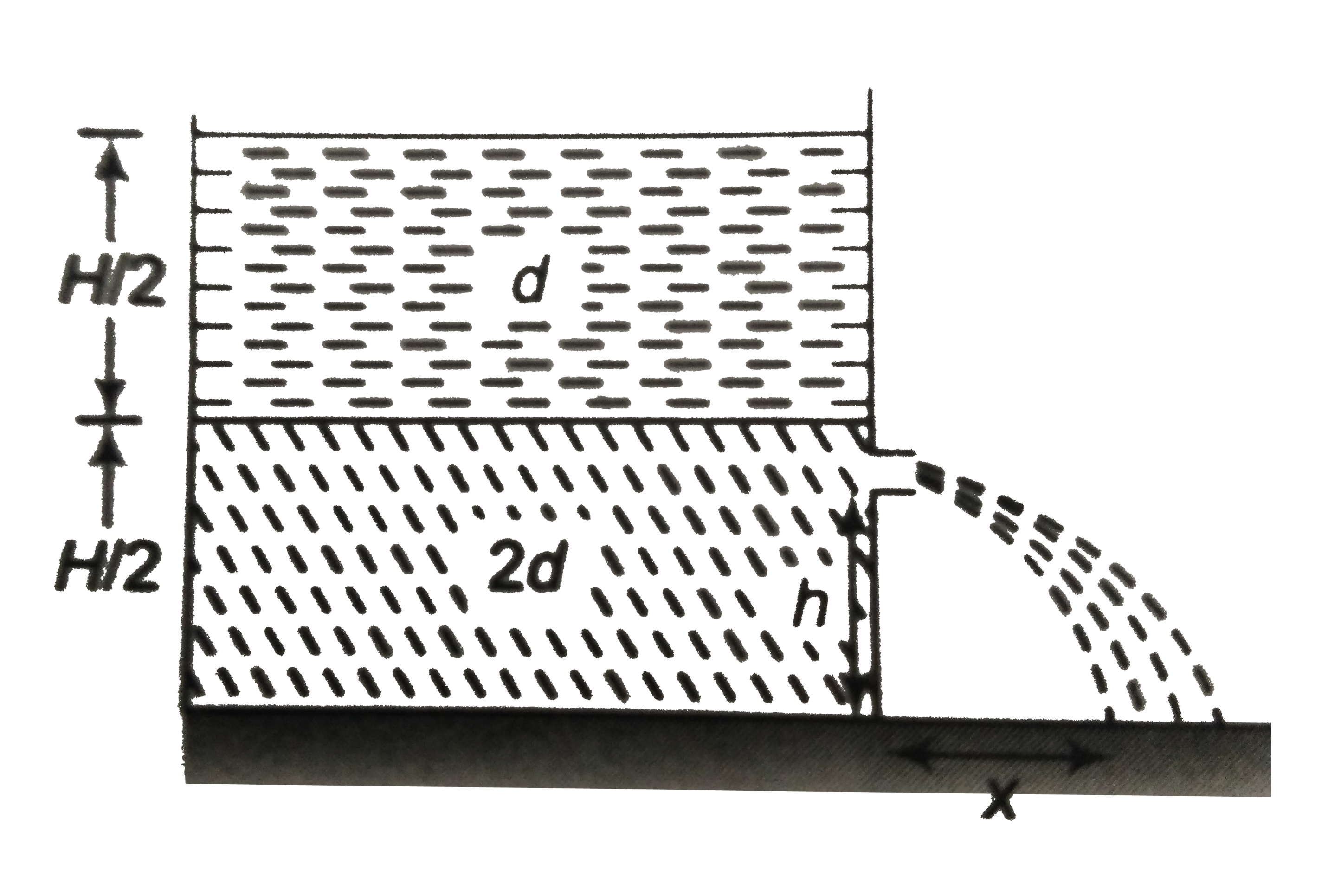
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The total pressure with cylinder, at the bottom of the container is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
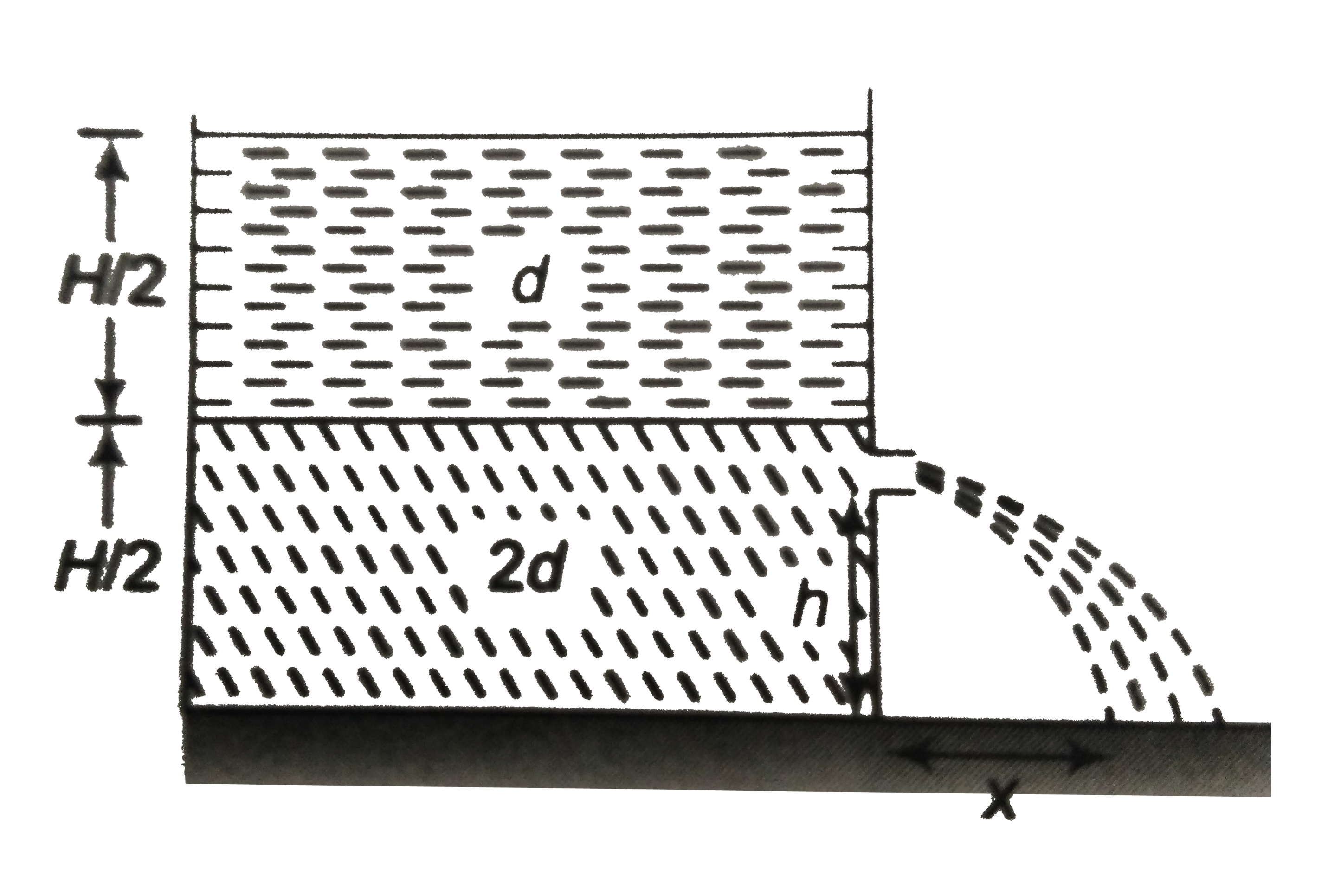
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial speed of efflux without cylinder is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
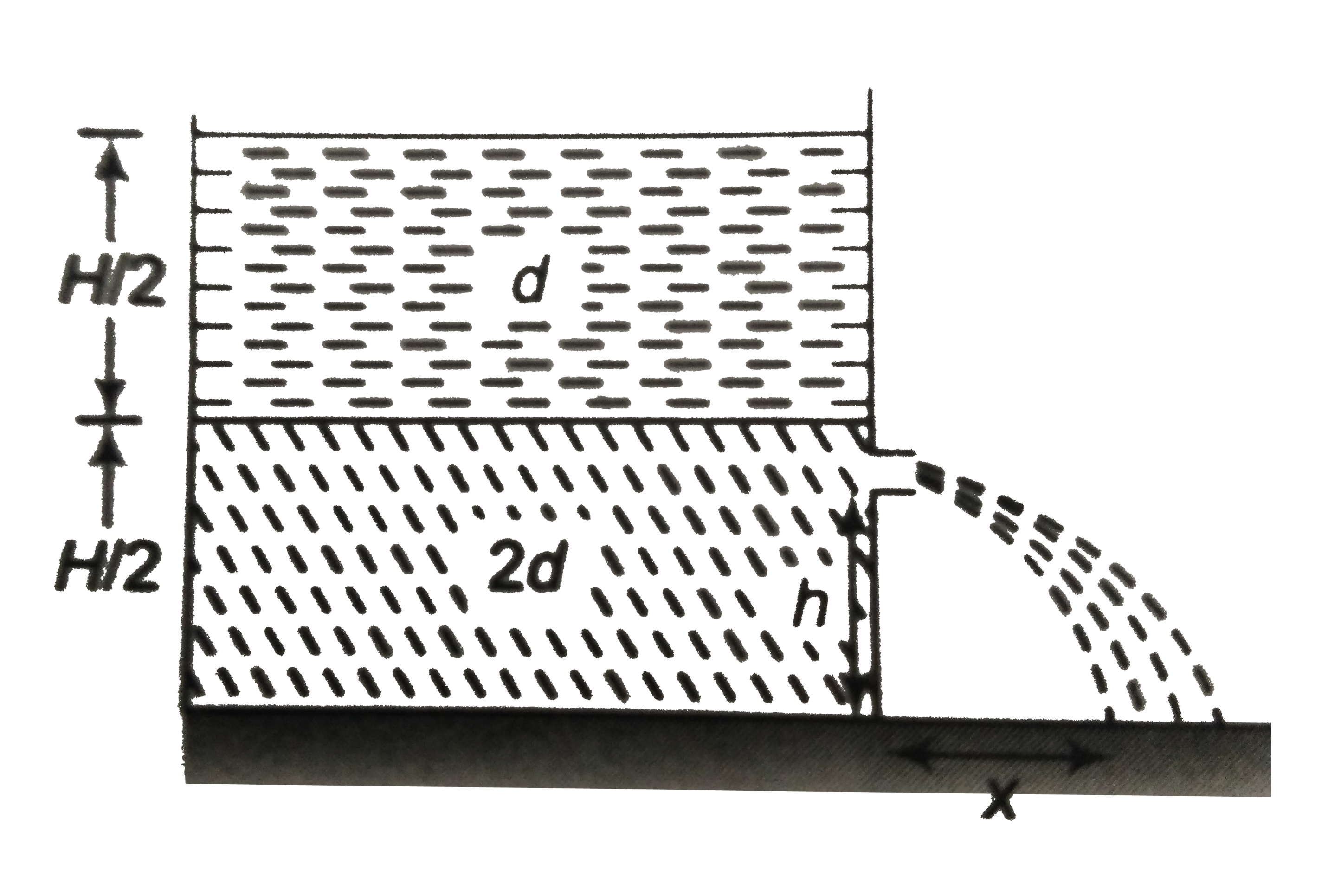
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial value of x is
View Solution
