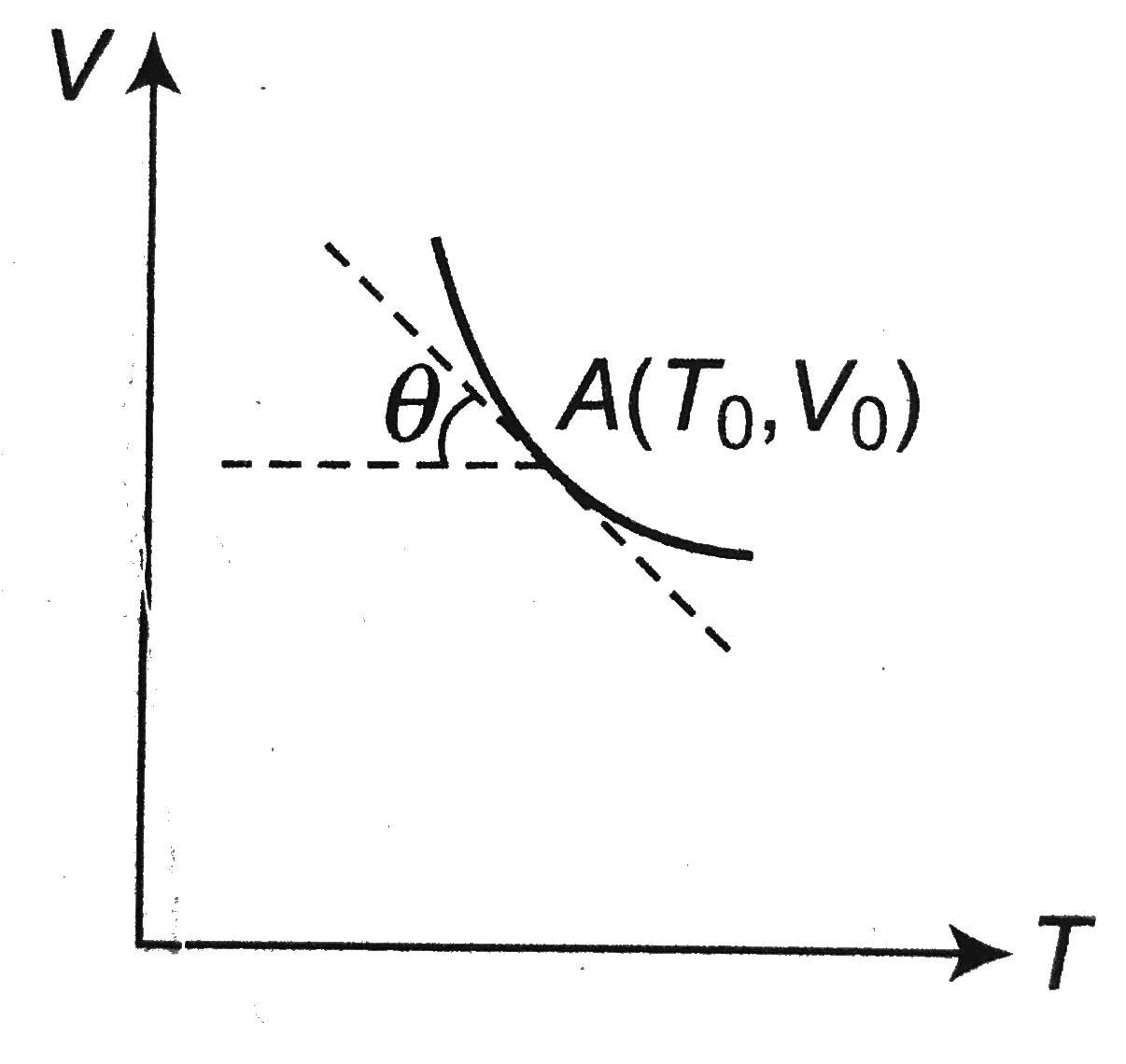
A gas is undergoing an adiabatic process. At a certain stage A, the va

A gas is undergoing an adiabatic process. At a certain stage, the volume and absolute temperature of the gas are $V_{0}, T_{0}$ and the magnitude of the slope of the V-T curve is m. molar specific heat of the gas at constant pressure is [Assume the volume of the gas is taken on the y-axis and absolute temperature of the gas taken on x-axis]
A $\frac{m R T_{0}}{V_{0}}$
B $\frac{M R T_{0}}{2 V_{0}}$
C $\frac{(V_{0} + m T_{0}) R}{V_{0}}$
D $\frac{(V_{0} + m T_{0}) R}{2 V_{0}}$
For an adiabatic process the relation between V and T is given by
A $T V^{γ} =$ constant
B $T^{γ} V =$ constant
C $T V^{1 - γ} =$ constant
D $T V^{γ - 1} =$ constant
During an adiabatic process. The pressure of a gas is to be proportional to the cube of its absolute temperature The value of $γ$ for the gas is :
A $\frac{5}{3}$
B $\frac{7}{5}$
C $\frac{3}{2}$
D $\frac{11}{9}$

A gas is undergoing an adiabatic process. At a certain stage A, the values of volume and temperature $(V_{0}, T_{0})$ . From the details given in the graph, find the value of adiabatic constant $γ$