A train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an/ angle α when the front of the carriage reaches the junction and an angle β when the end of the carriage reaches it. Then, the two lines are inclined to each other at an angle theta, show that 2cotθ=cotα−cotβcotα+cotβ
Step by step video & image solution for A train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an/ angle alpha when the front of the carriage reaches the junction and an angle beta when the end of the carriage reaches it. Then, the two lines are inclined to each other at an angle theta, show that 2 cot theta=cot alpha-cot beta cot alpha + cot beta by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Single Option Correct Type Questions)
65 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (More Than One Correct Option Type Questions)
24 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 7
9 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
A train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an angle α when the front of the carriage reaches the junction, and an angle β when the end of the carriage reaches it. Prove that the two lines are inclined to each other at an angle θ determined by 2cotθ=cotα−cotβ.
View SolutionIf cot2θ=cot(θ−α)cot(θ−β), then show that cot2θ=12[cotα+cotβ]
View Solution
Knowledge Check
A plumb line gets inclined to vertical at angle θ when the carriage travels with an acceleration a. The plumb line is suspended from the roof of the carriage. The acceleration a is given by
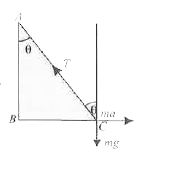 Aa=gtanθBa=gsinθCa=gcosθDa=gsecθ
Aa=gtanθBa=gsinθCa=gcosθDa=gsecθ
Given tanθ+cotϕ=αandcotθ+tanϕ=β
View SolutionIf tanα=x+1,tanβ=x−1, show that 2cot(α−β)=x2
View SolutionIf tanα=x+1,tanβ=x−1, show that 2cot(α−β)=x2.
View SolutionIf tanα=x+1,tanβ=x−1, show that 2cot(α−β)=x2.
View Solutionαandβ are unit vectors inclined to each other at an angle α,α∈(0,π)and∣∣→a+→b∣∣<1, Then α∈
View SolutionIf cot αcotβ=2 show that cos(α+β)cos(α−β)=13
View Solutionαandβ are acute angles and cos2α=3cos2β−13−cos2β then tanαcotβ=
View Solution
