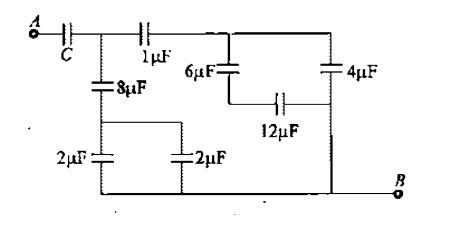
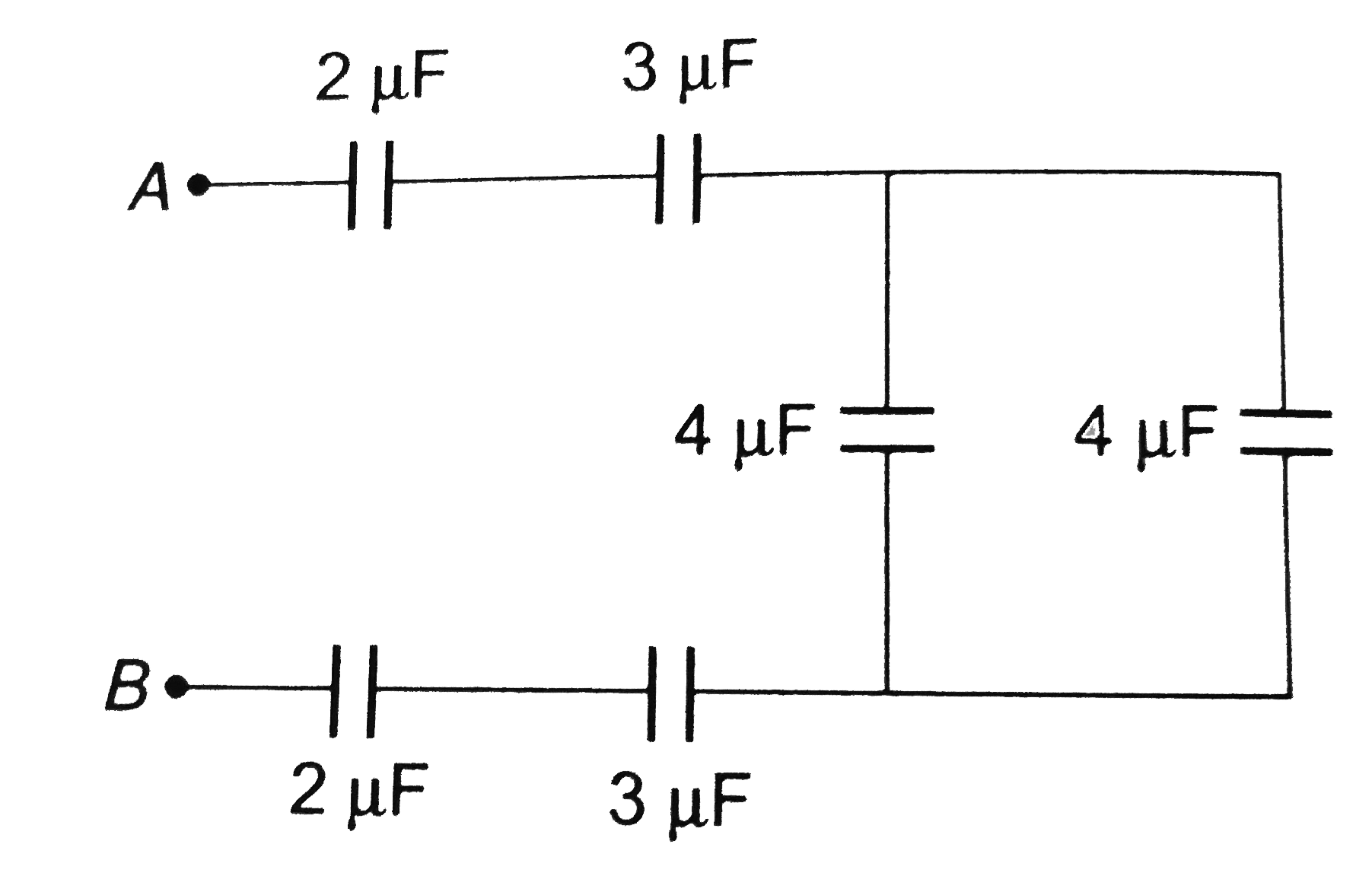
In the circuit as shown in the figure the effective capacitance between A and B is
A
3μF
B
2μF
C
4μF
D
8μF
Video Solution
Text Solution
The correct Answer is:C
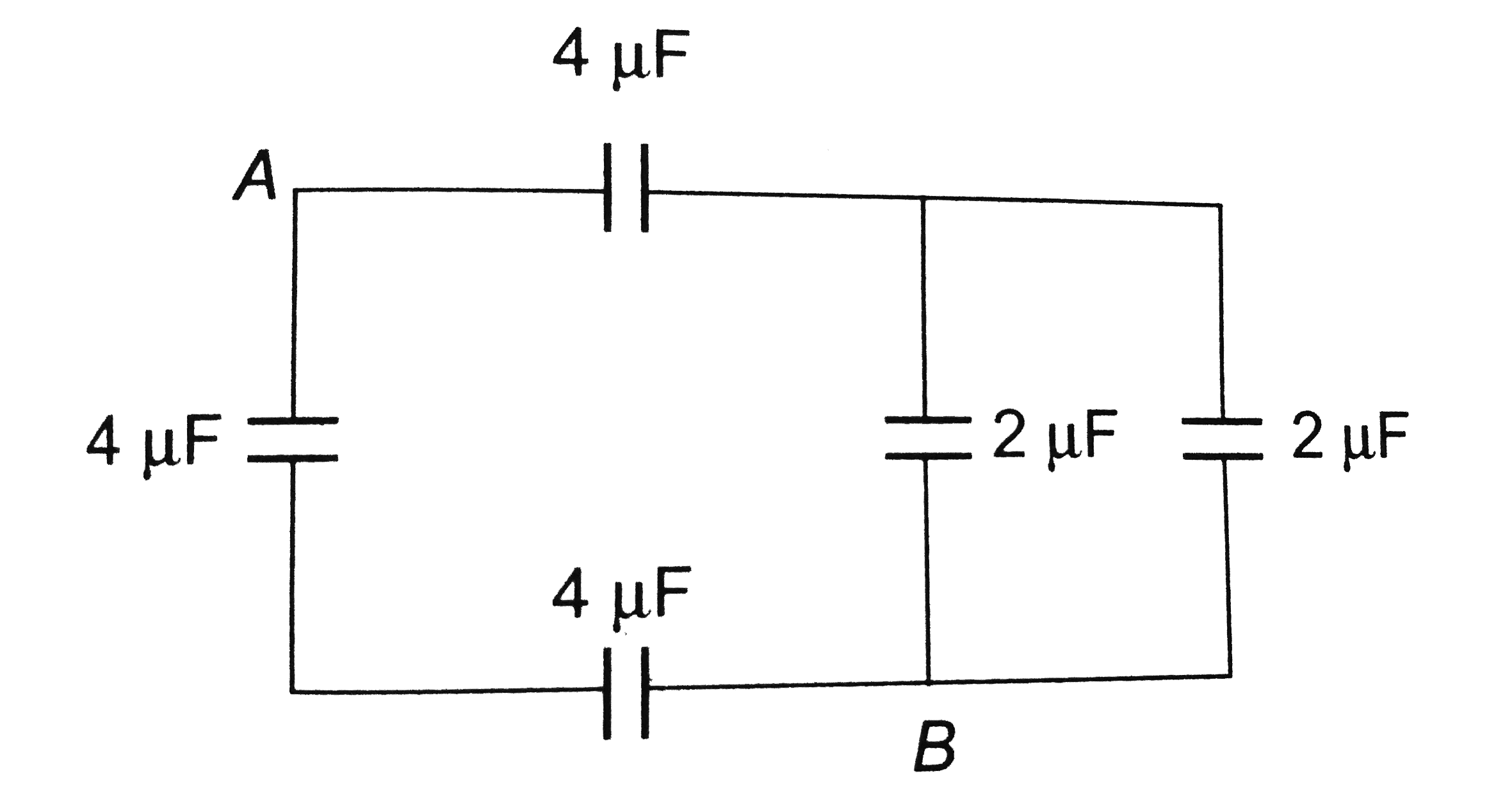
The given circuit can be simplified as follows
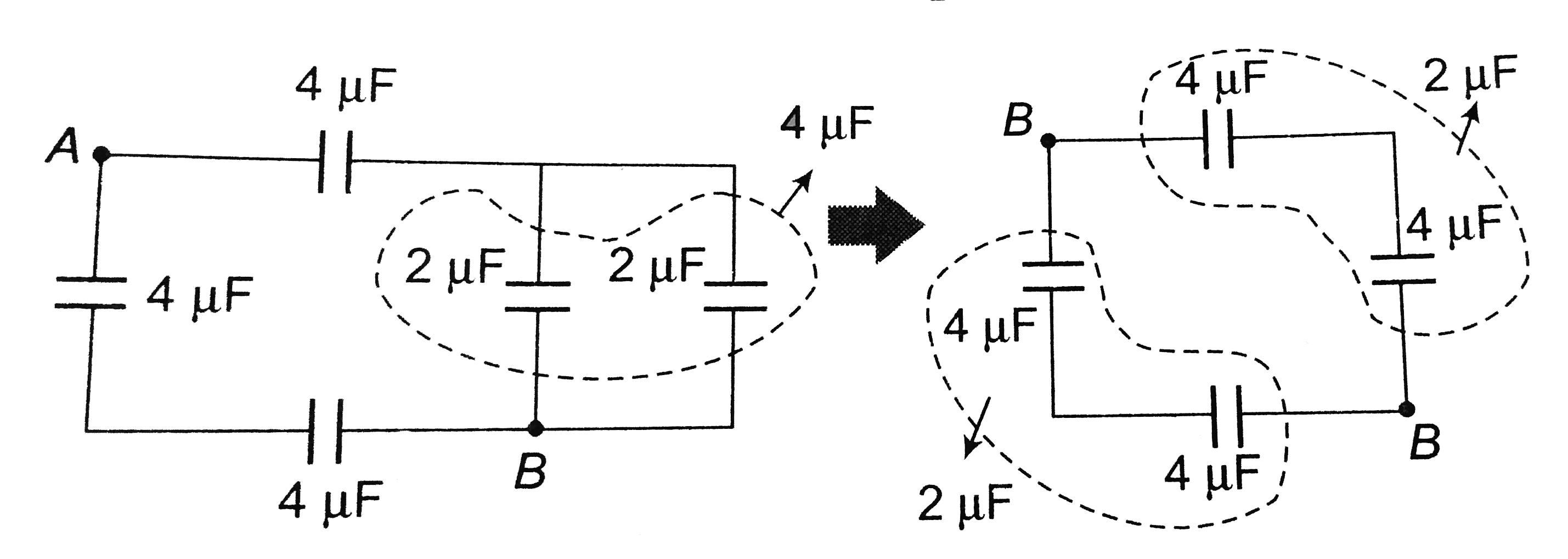
Equivalent capacitance between A and B is CAB=4μF
|
Topper's Solved these Questions
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Problems Based On Mixed Concepts
44 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Section B - Assertion Reasoning
17 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Grouping Of Capacitors
48 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section D - Chapter End Test
29 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - A2ZCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
Knowledge Check
In the circuit as shown in the figure the effective capacitance between A and B is
 A3μFB2μFC4μFD8μF
A3μFB2μFC4μFD8μFSix capacitors each of capacitance of 2μF are connected as shown in the figure. The effective capacitance between A and B is:
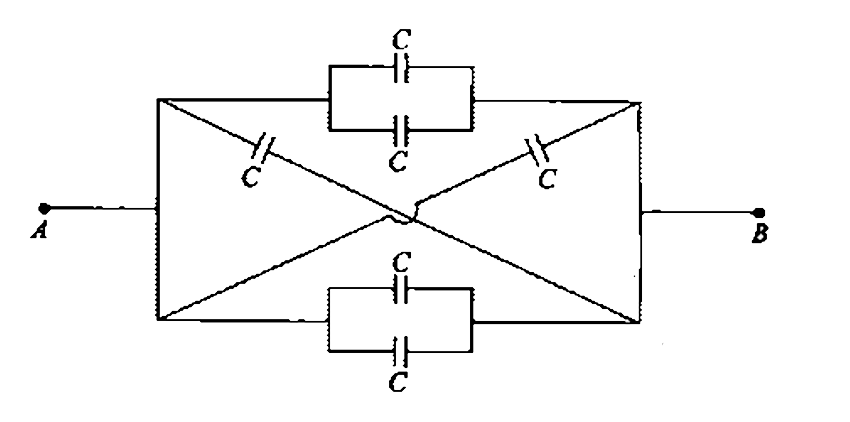 A12μFB8/3μFC3μFD6μF
A12μFB8/3μFC3μFD6μFEight capacitors , each of capacitance 2μF are conneted as shown in the figure . The effective capacitance between point A and B is
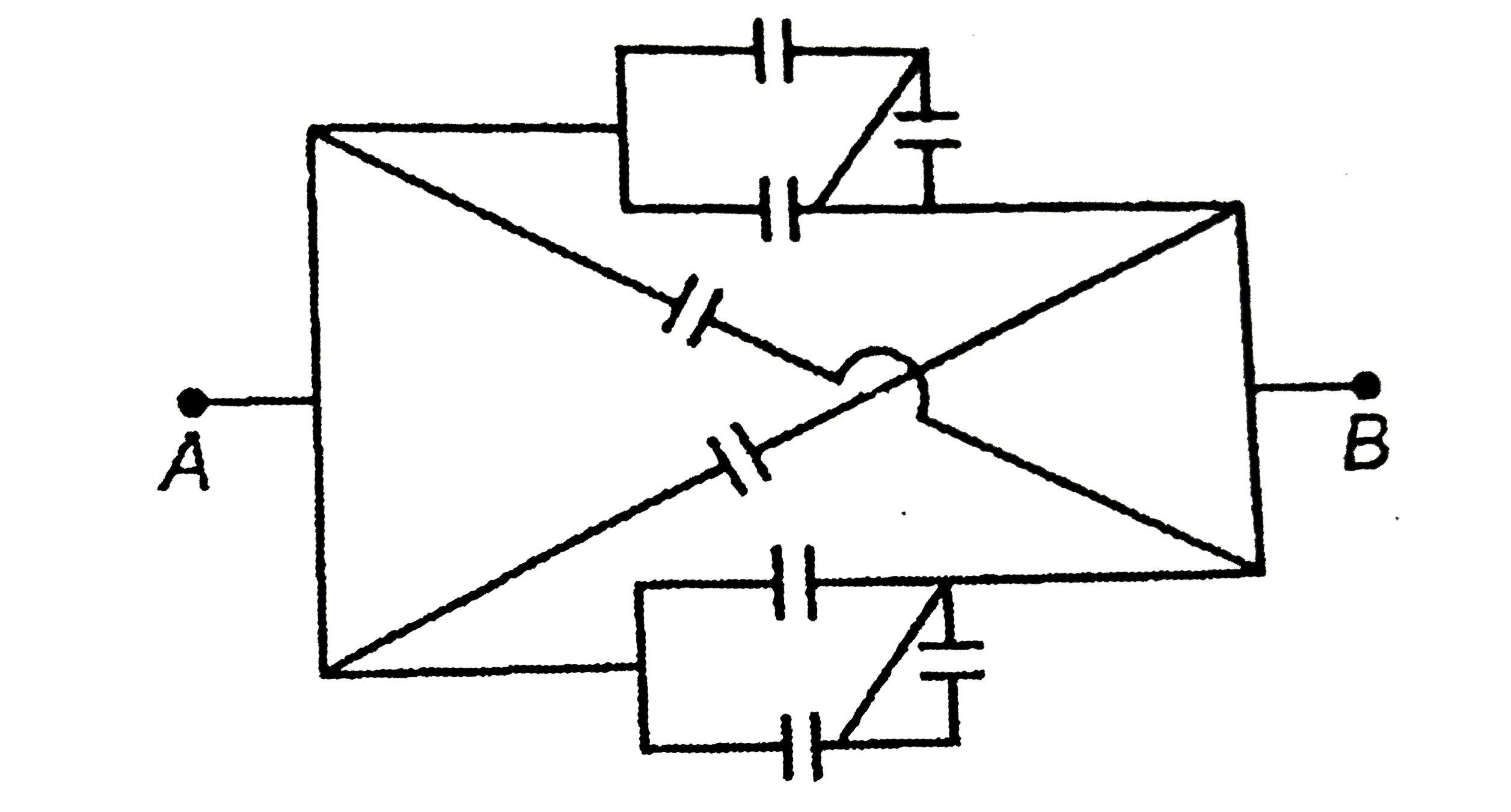 A10μFB12μFC8μFDZero
A10μFB12μFC8μFDZero
Four capacitors are connected in a circuit as shown in the figure. The effective capacitance in μF between points A and B will be
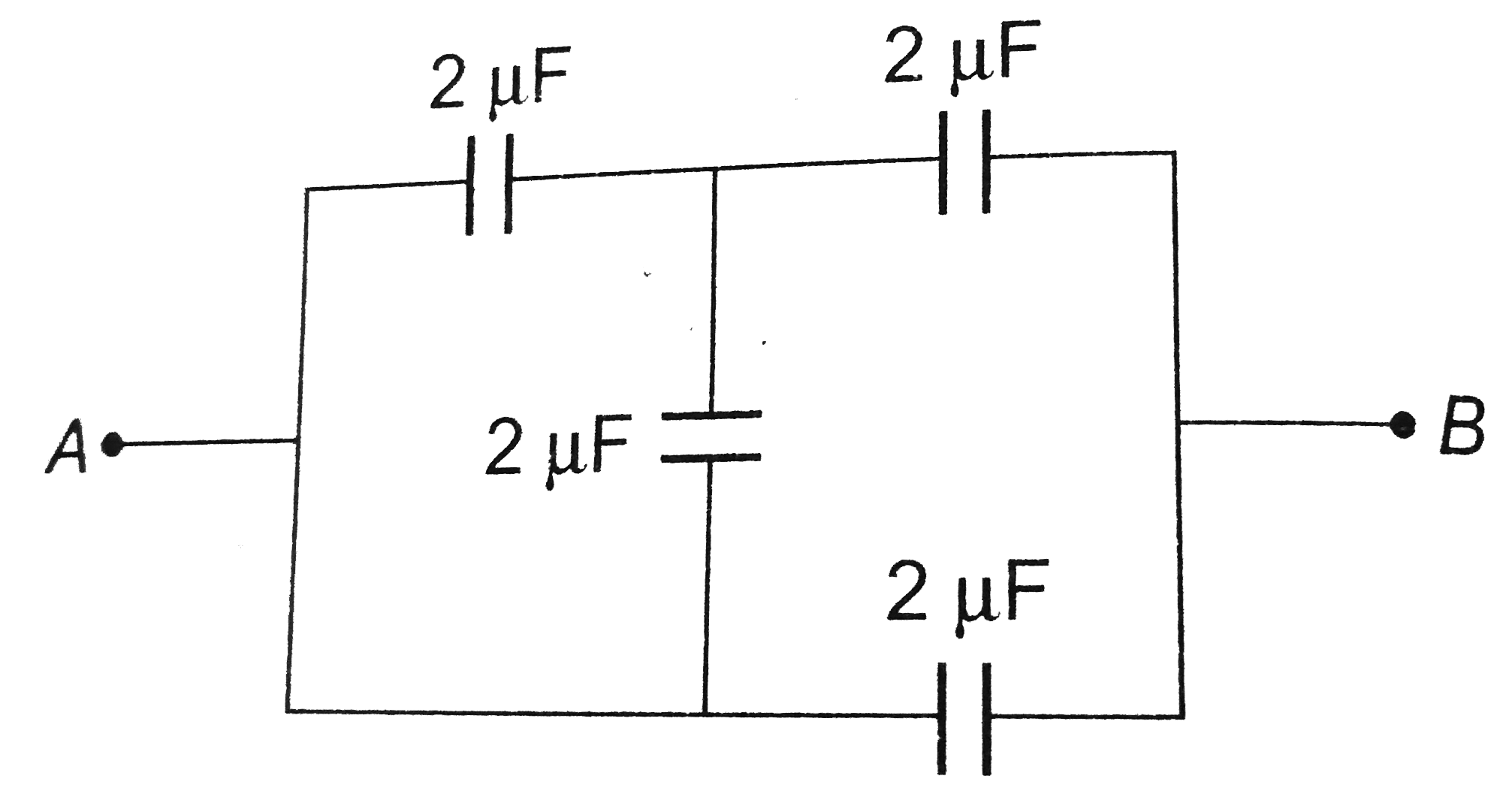
View SolutionConsider an infinite matrix of capacitors as shown in the figure.
The effective capacitance between point A and B will be

View SolutionAn infinite number of identical capacitors each of capacitance 1mF are connected as shown in the figure. Then the equivalent capacitance between A and B is.
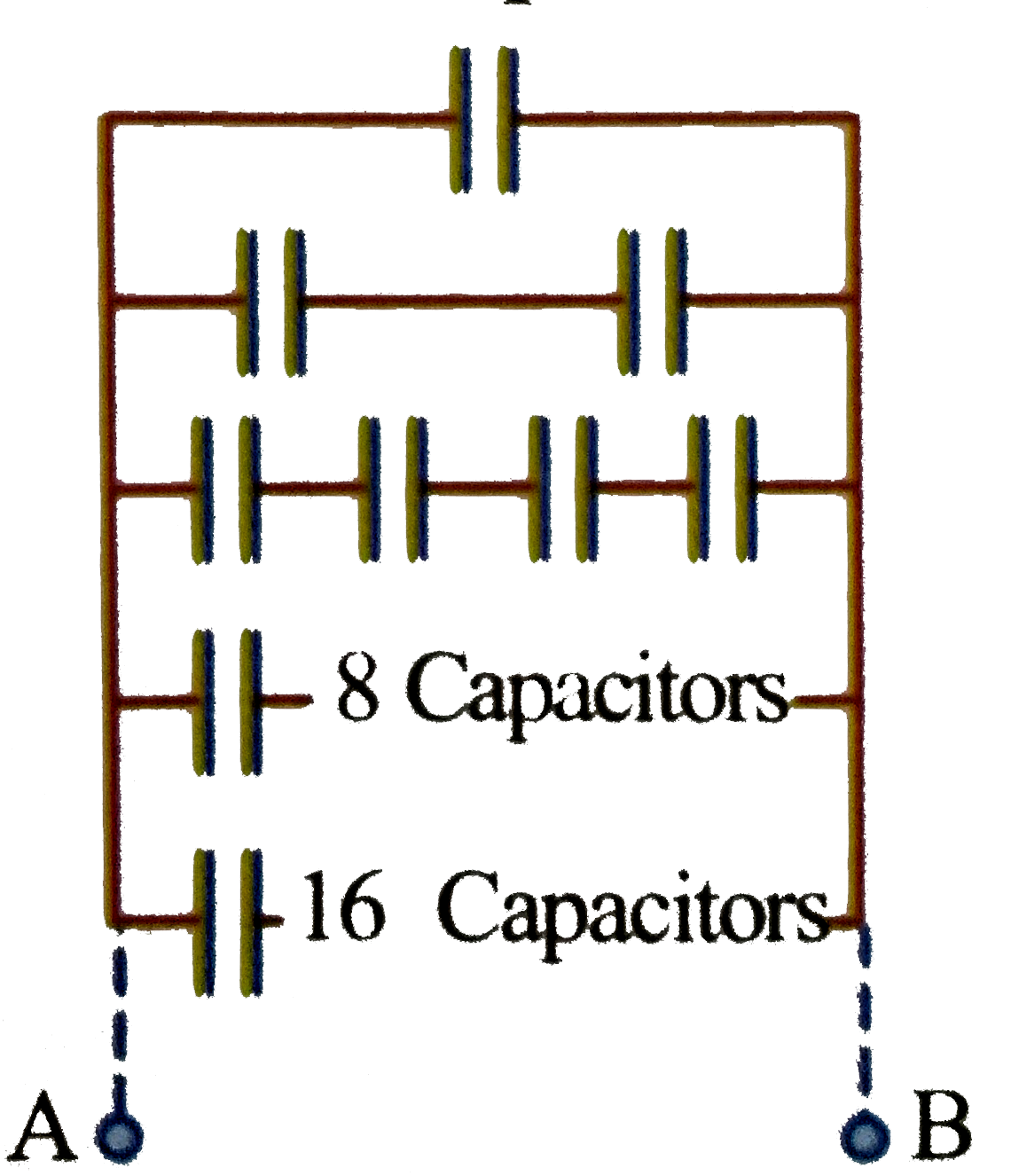 .
.
View SolutionThe effective capacitance between A and B in the figure given is
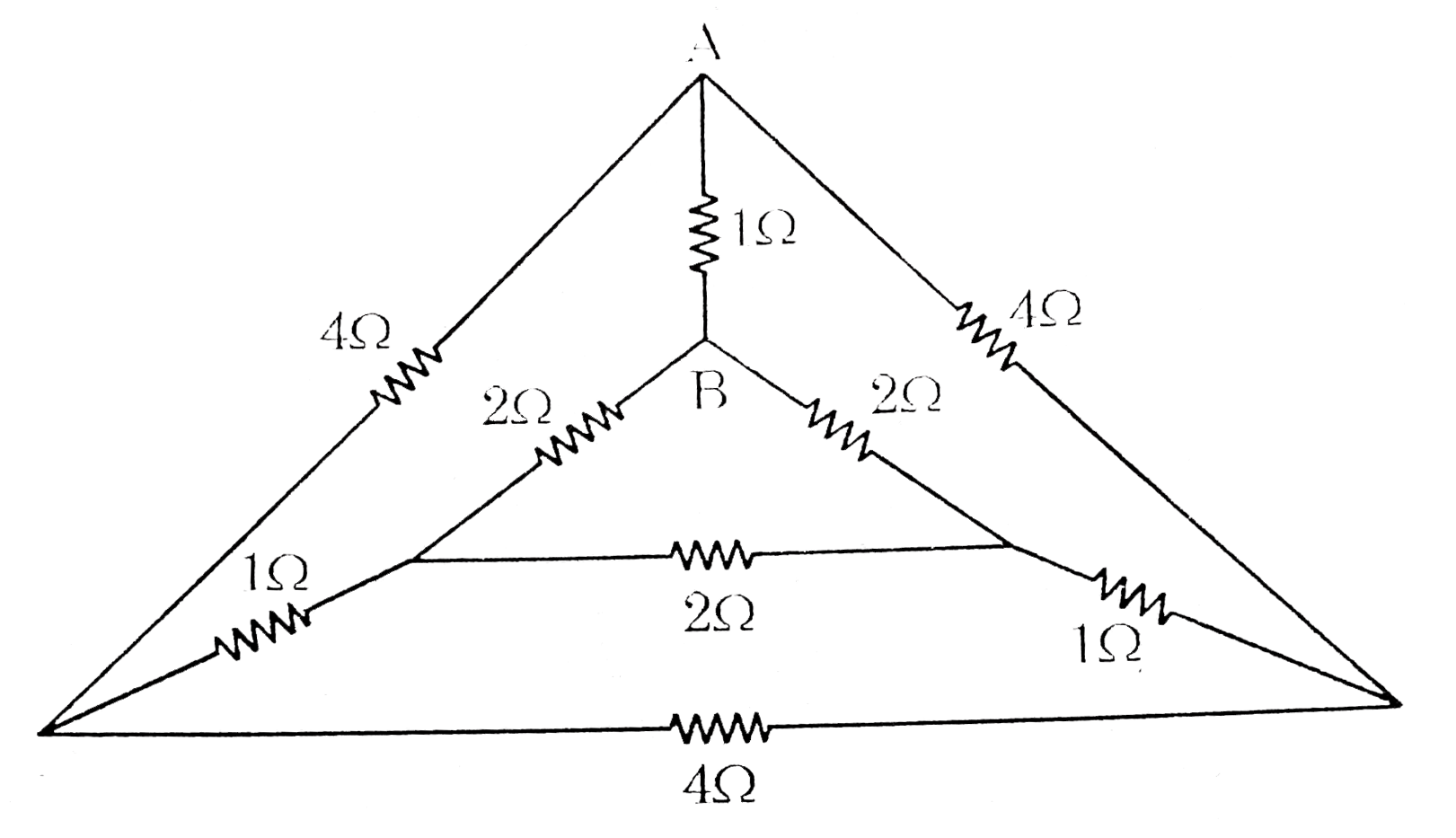
View SolutionConsider the circuit as shown in figure. The equivalent resistance between A and B is
View Solution