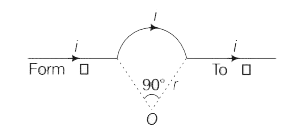
The magnetic field at centre O of the arc in Fig.
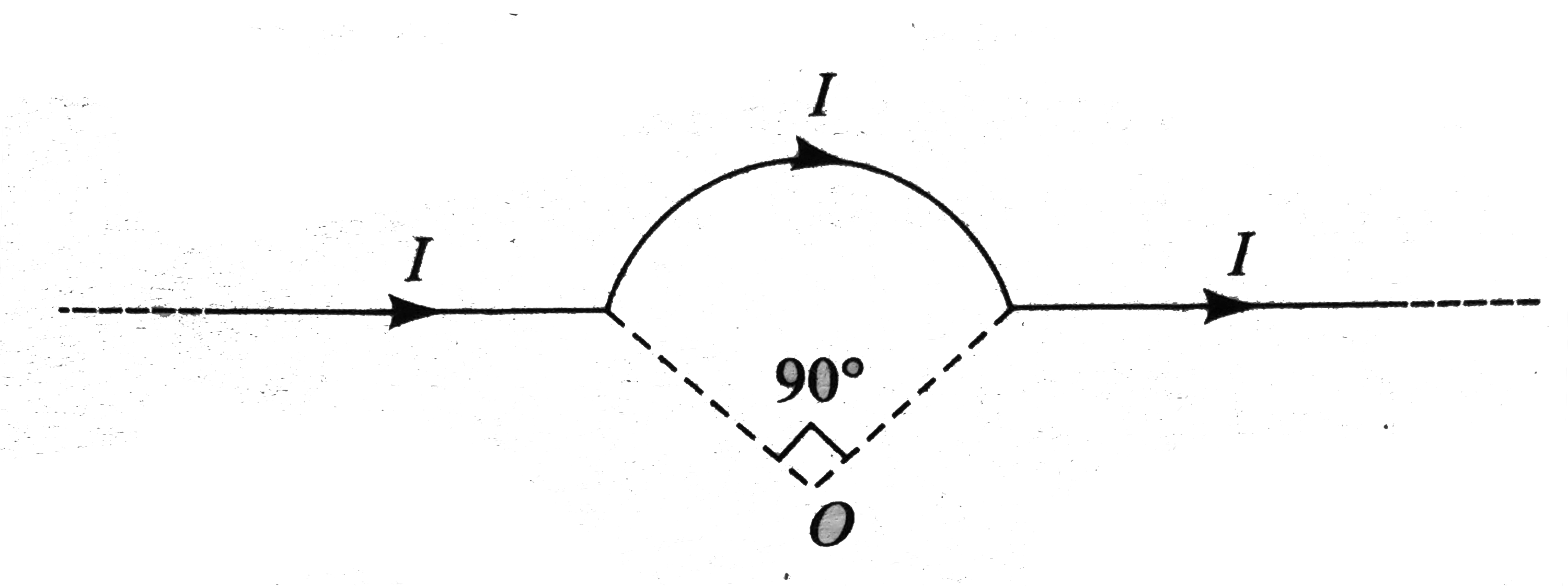
μ0I4π×2Ir[√2+π]
μ0I4π×2Ir[√2+π4]
μ04π×Ir[√2+π]
μ04π×Ir[√2+π4]
The correct Answer is:B
Distance of stright conductor from
B=μ0I×√22πr+μ0I2rπ2×2π
Or B=μ0I4π+2Ir[√2π4].
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Problems Based On Mixed Concepts32videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Section B - Assertion Reasoning31videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Magnetic Field Due To Current44videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SEMICONDUCTOR ELECTRONICSEXERCISE - EXERCISE29videos
SEMICONDUCTOR ELECTRONICS
- BOOK - A2ZCHAPTER - WAVE OPTICSEXERCISE - Section D - Chapter End Test29videos
WAVE OPTICS
Similar Questions
In the net work shown in figure,find the magnetic field at the centre O of the coil.
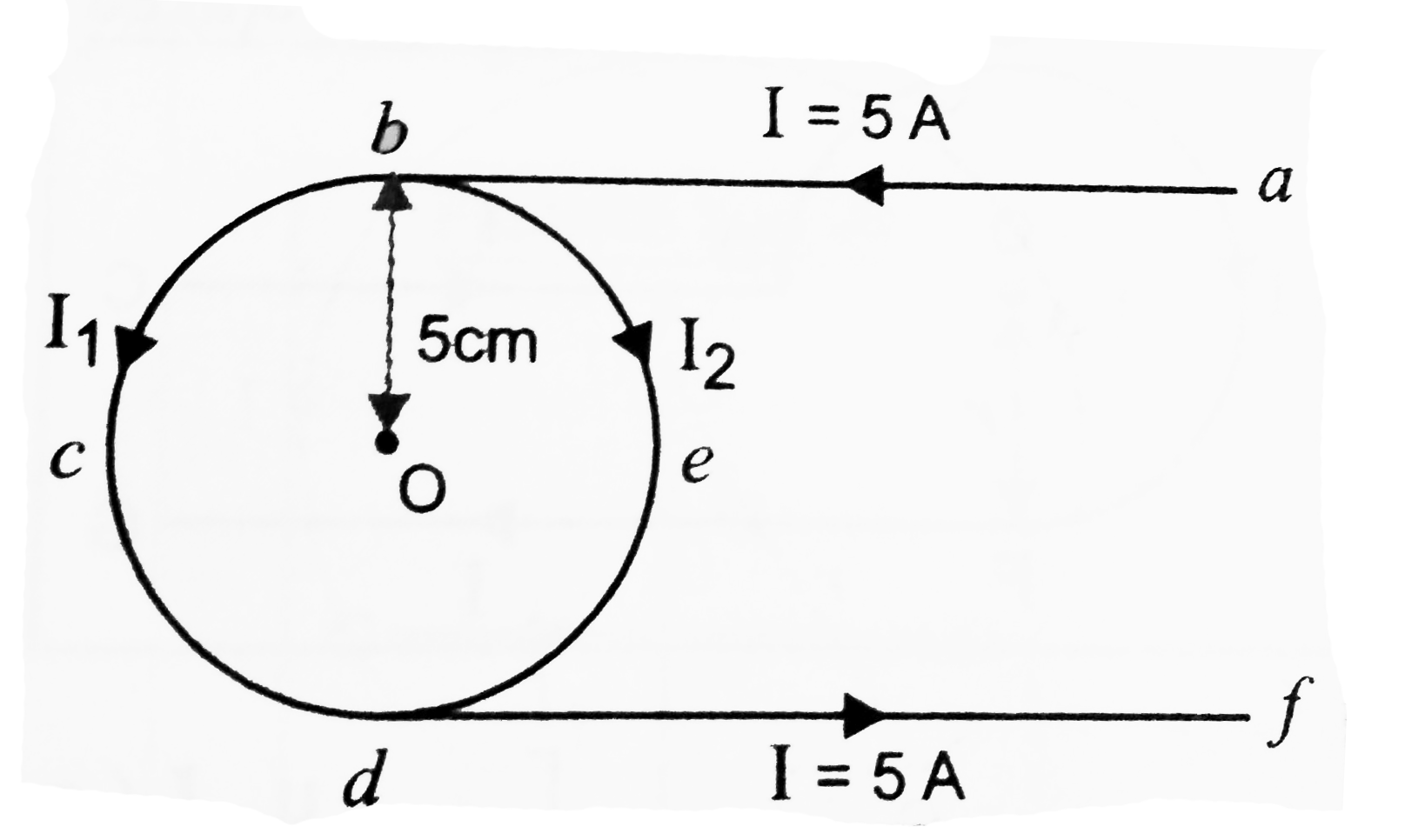 View Solution
View SolutionA battary is connected between two points A and B on the circumference of a uniform conducting ring of radius r and resistance R as shown in Fig. One of the arcs AB of the ring subtends angle θ at the centre. Show that that the magnetic field at the centre of the coil is zero and independent θ.
View Solution
Knowledge Check
- Question 1 - Select One
The magnetic field at O is
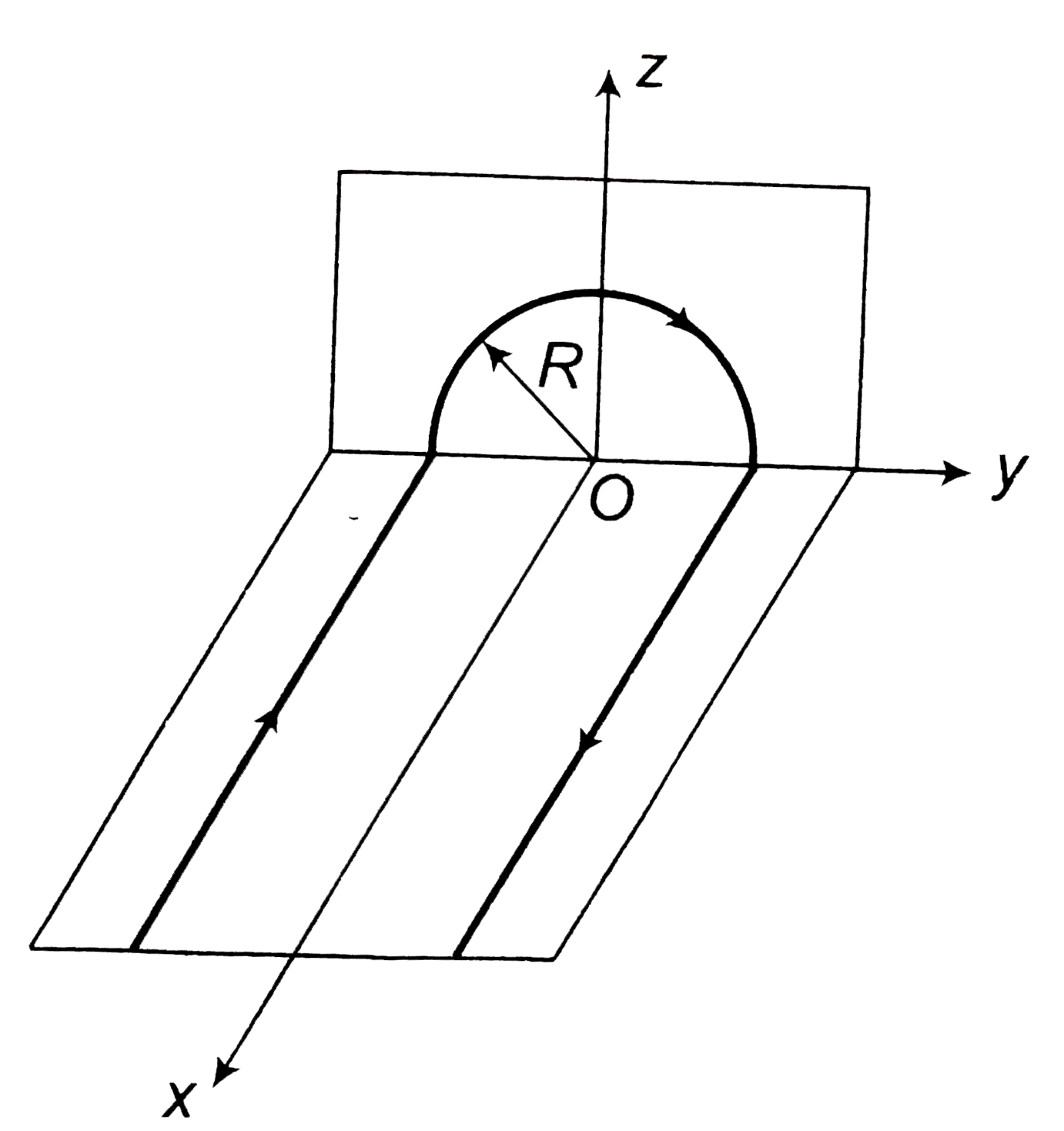 Aμ0I2R√(14+1π2)Bμ0I2R√(12+1π2)Cμ0IR√(14+1π2)Dμ0IR√(14+1π)
Aμ0I2R√(14+1π2)Bμ0I2R√(12+1π2)Cμ0IR√(14+1π2)Dμ0IR√(14+1π) - Question 2 - Select One
The magnetic field at O is
 AzeroB1C2D3
AzeroB1C2D3 - Question 3 - Select One
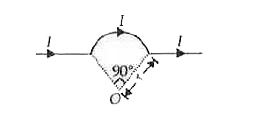
The magnetic field at the centre O of the are shown in the figure is
 A2I(√2+π)×10−7rB2I(√2+π4)×10−7rCI(√2+π)×10−7rDI(√2+π4)×10−7r
A2I(√2+π)×10−7rB2I(√2+π4)×10−7rCI(√2+π)×10−7rDI(√2+π4)×10−7r
The magnetic field at centre , P will be
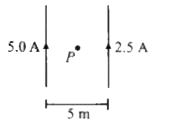 View Solution
View SolutionThe magnetic field at the centre O of the circular portion of radius 3cm carrying current 4 ampere in the wire is
 View Solution
View SolutionWhat is the magnetic field at the centre of arc in the figure below?
 View Solution
View Solution
A conducting wire carrying a current I is bent into the shape as shown. The net magnetic field at the centre O of the circular arc of radius RView SolutionThe magnetic field at the centre the figure is
 View Solution
View Solution

