Cross-section of an infinite cylinder is shown. A current $I$ uniformely distributed over its cross-section is flowing along its length. Now a cylinder of radius $R / 2$ is checked out of this. Then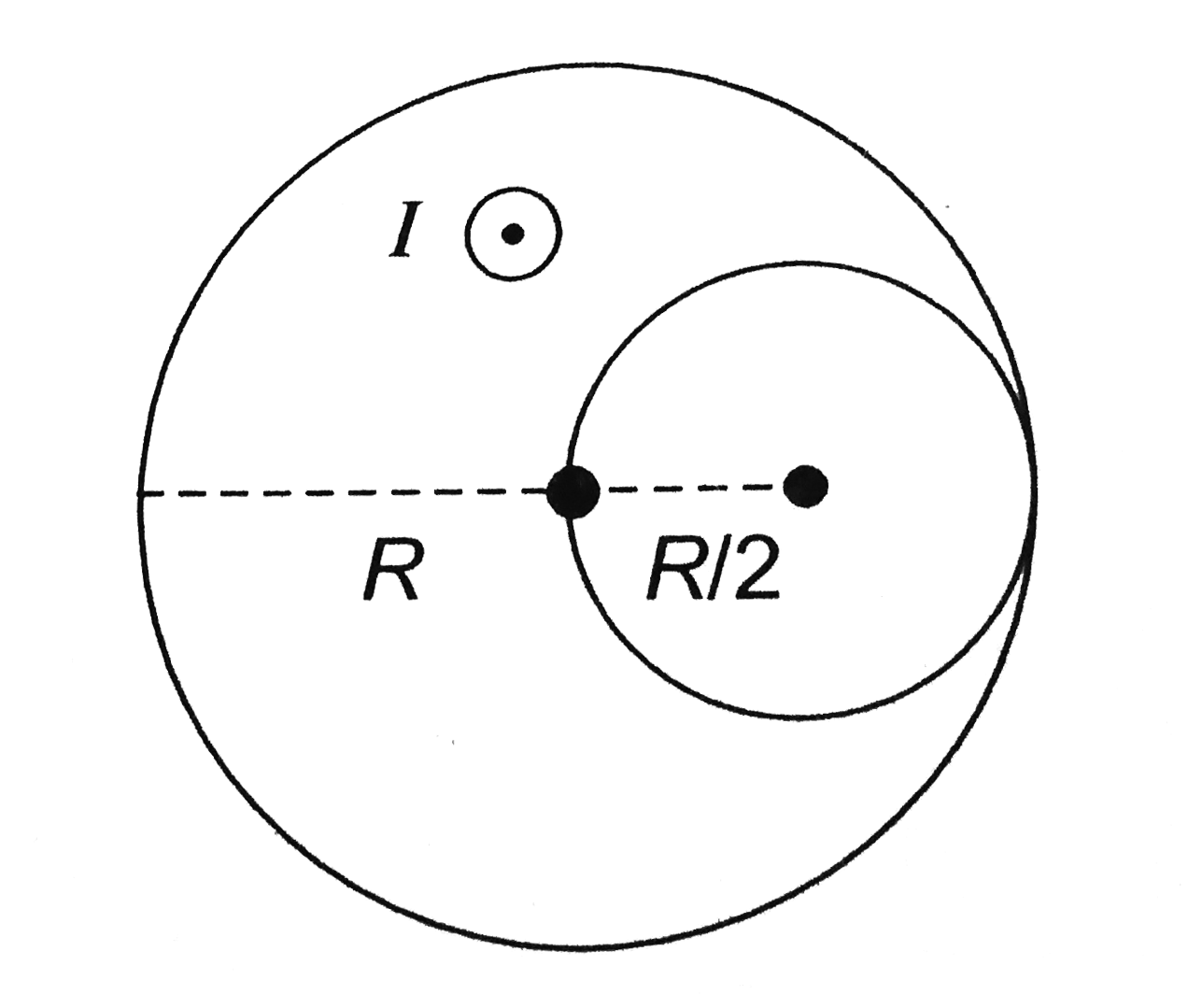

magnetic fields at the centers of both cylinders is same both in magnitude and direction

magnetic fields at the centre of both cylideres is same in magnitude but opposite in direction

magnetic fields at the centres of both cylinders is same in direction but unequal in magnitude.

magnetic field at the centre of both cylinder is opposite in direction and unequal in magnetude

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

A long cylindrical wire carries a steady current which is uniformly distributed across its cross section. Which of the following is true?
AMagnetic field is zero at the centre.
BElectric field is zero at the centre.
CElectric field just outside the cylinder is zero.
DMagnetic field just outside the cylinder is zero.
The figure shows a long coaxial cable in which a current I flows down through the inner cylinder of radius a and the same current flows back up through the outer cylinder of radius 2a. The cylinders are insulated from each other and the current is uniformly distributed over the area of the cross-section in each cylinder. The strength of the magnetic field at a distance $3 a / 2$ from the axis of the cable is

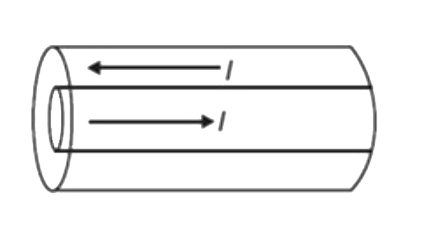A $B = \frac{5 μ_{0} I}{36 π a}$
B $B = \frac{5 μ_{0} I}{12 π a}$
C $B = \frac{7 μ_{0} I}{12 π a}$
D $B = \frac{7 μ_{0} I}{36 π a}$
A long straight wire of radius 10mm carries a current uniformly distributed over its cross section. The ration of magnetic fields due to the wire at distance of 5mm and 15 mm, respectively from the axis of the wire is
A $\frac{3}{4}$
B $\frac{2}{3}$
C $\frac{1}{3}$
D2