In the figure shown , for an angle of incidence , at the top surface , what is the minimum refractive index needed for the internal reflection at vertical face ?
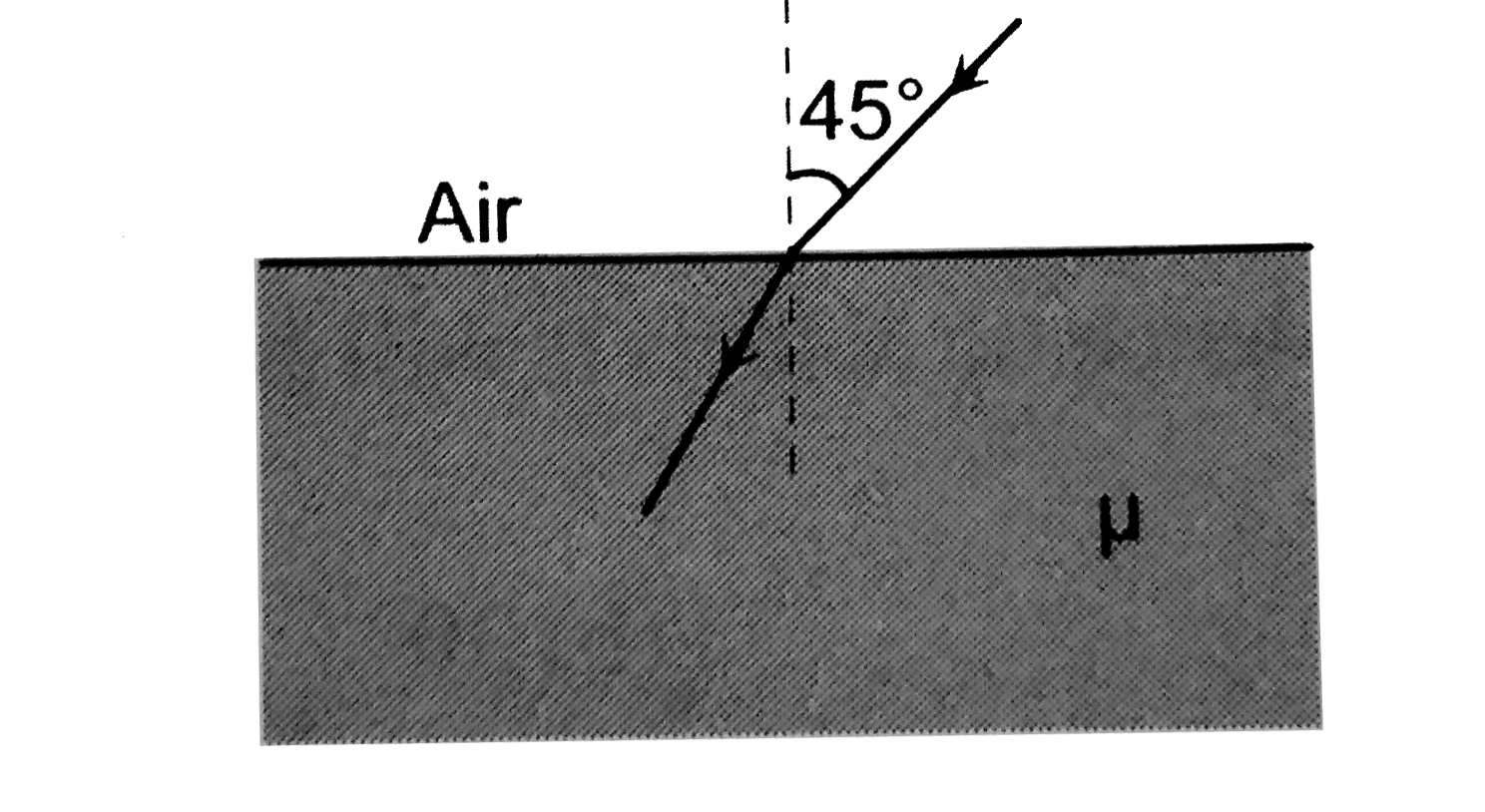
The correct Answer is:B
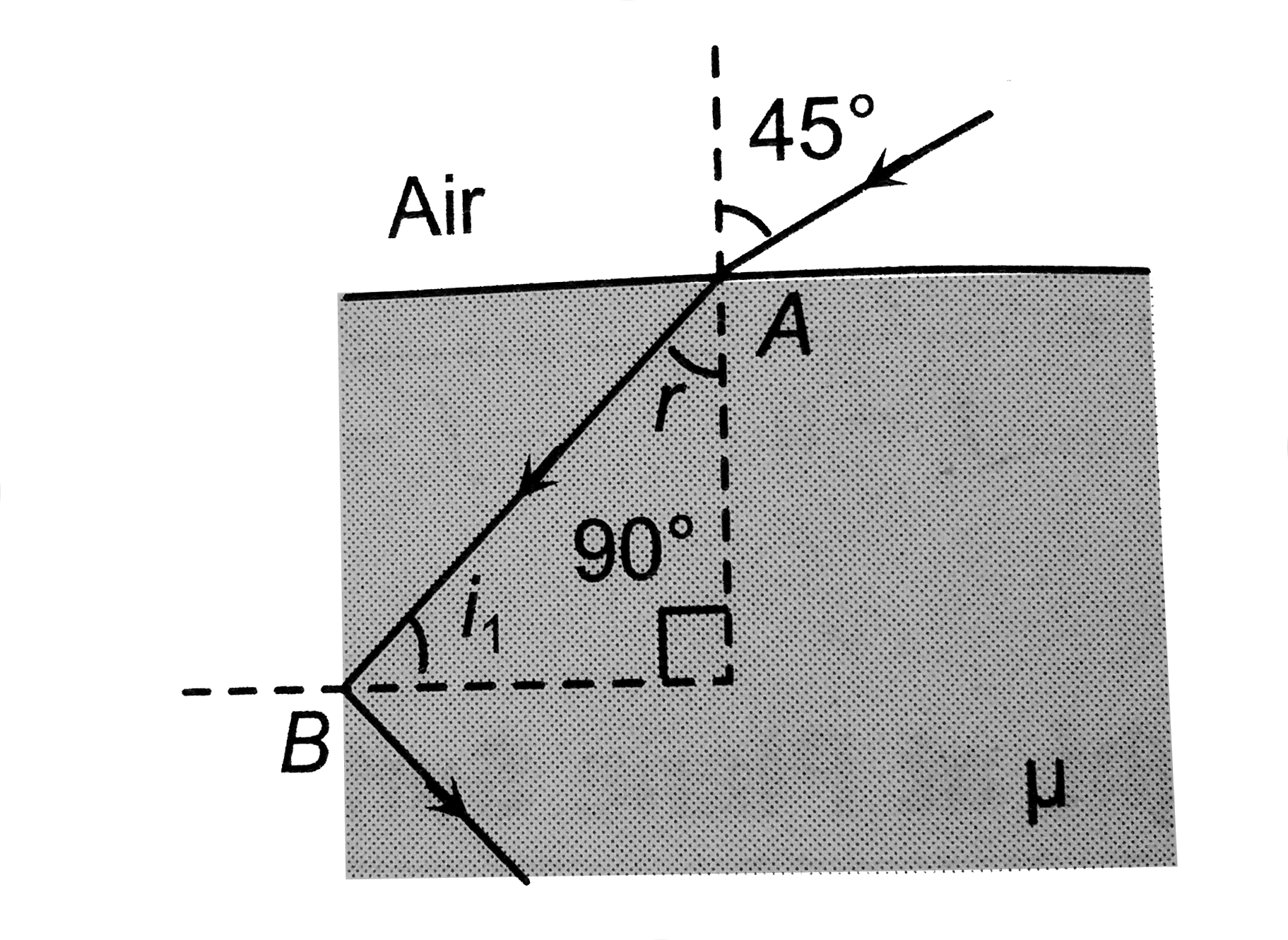
At point , by Snell's law
…(i)
At point , for total internal reflection From figure ,
…(ii)
Now ...(iii)
From equation (ii) and (iii)
Squaring both side and then solving we get
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Refraction At Curved Surface64videos
GEOMETRICAL OPTICS
- BOOK - A2ZCHAPTER - GEOMETRICAL OPTICSEXERCISE - Prism Theory And Dispersion Of Light45videos
GEOMETRICAL OPTICS
- BOOK - A2ZCHAPTER - ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM EXERCISE - Section D - Chapter End Test30videos
ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM
- BOOK - A2ZCHAPTER - MAGNETISM AND MATTEREXERCISE - Section D - Chapter End Test30videos
MAGNETISM AND MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
In the figure shown , for an angle of incidence 45∘, at the top surface , what is the minimum refractive index needed for the internal reflection at vertical face ?
 A√2+12B√3/2C√1/2D√2+1
A√2+12B√3/2C√1/2D√2+1 - Question 2 - Select One
The angle of incidence on the surface of a diamond of refractive index 2.4, if the angle between the reflected and refracted rays is 90° is
Atan−1(2.4)B2sin−1(12.4)Ctan−1(12.4)Dcos−1(12.4) - Question 3 - Select One
A light ray is incident on a transparent sphere of index = √2 , at an angle of incidence =45∘ , What is the deviation of a tiny fraction of the ray, which enters the sphere, undergoes two internal reflections and then refracts out into air?
A270∘B240∘C120∘D180∘
A prism is shown in the figure with prism angle 75∘ and refractive index √3. A light ray incidents on a surface at incident angle θ. Other face is coated with a medium of refractive index n. for θ≤60∘ ray suffers total internal reflection find value of n2.
_PHY_E01_011_Q01.png) View Solution
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face ? The refractive index of the material of the prism is 1.524.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View SolutionA ray of light is incident on the surface of a glass plate of refractive index 1.6 at polarzing angle. Calculate the angles of reflection and refraction.
View SolutionFor a situation shown in figure, find the refrective index of glass so that it will suffer total internal reflection at the vertical surface.
 View Solution
View Solution

